A three-wheeler scooter charges 15 for first kilometer and 8 each for every subsequent kilometer. For a distance of x km, an amount of y is paid. Write the equation representing the above information
To derive the equation representing the cost of a three-wheeler scooter ride based on the given information, we can follow these steps:
Step 1: Understand the Charges
The scooter charges:
- ₹15 for the first kilometer
- ₹8 for each subsequent kilometer
Step 2: Define the Variables
Let:
- x = total distance traveled in kilometers
- y = total amount paid in rupees
Step 3: Calculate Charges for the First Kilometer
For the first kilometer, the charge is ₹15.
Step 4: Calculate Charges for Subsequent Kilometers
If the total distance is x kilometers, then the distance after the first kilometer is x−1 kilometers.
The charge for these subsequent kilometers is:
- ₹8 for each kilometer, so for x−1 kilometers, the charge is 8(x−1).
Step 5: Write the Total Cost Equation
The total amount paid y can be expressed as the sum of the charge for the first kilometer and the charge for the subsequent kilometers:
y=15+8(x−1)
Step 6: Simplify the Equation
Now, simplify the equation:
y=15+8x−8
y=8x+7
Final Equation
Thus, the equation representing the total amount paid for a distance of x kilometers is:
y=8x+7
---
The scooter charges:
- ₹15 for the first kilometer
- ₹8 for each subsequent kilometer
Let:
- x = total distance traveled in kilometers
- y = total amount paid in rupees
For the first kilometer, the charge is ₹15.
If the total distance is x kilometers, then the distance after the first kilometer is x−1 kilometers.
The charge for these subsequent kilometers is:
- ₹8 for each kilometer, so for x−1 kilometers, the charge is 8(x−1).
The total amount paid y can be expressed as the sum of the charge for the first kilometer and the charge for the subsequent kilometers:
y=15+8(x−1)
Now, simplify the equation:
y=15+8x−8
y=8x+7
Thus, the equation representing the total amount paid for a distance of x kilometers is:
y=8x+7
Topper's Solved these Questions
Similar Questions
The taxi fare in a city is as follows: For the first kilometre, the fare is Rs 8 and for the subsequent distance it is Rs 5 per km. Taking the distance covered as x km and total fare as Rs y, write a linear equation for this information, and draw its graph.
View SolutionThe taxi fare in a city is as follows: For the first kilometre, the fare is Rs 8 and for the subsequent distance it is Rs 5 per km. Taking the distance covered as x km and total fare as Rs y, write a linear equation for this information, and draw it's graph
View Solution
Knowledge Check
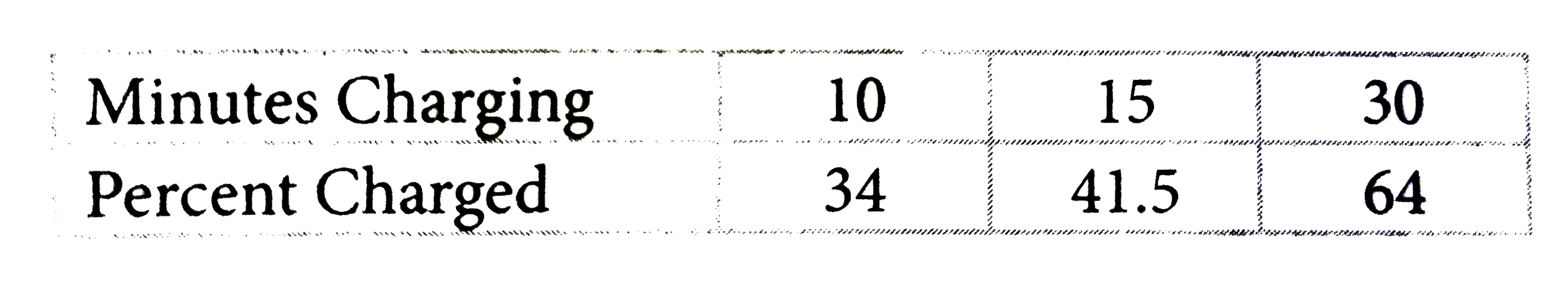
Xia is charging her laptop. She records the battery charge for the first 30 minutes after she plugs it in to get an idea of when it will be completely charged. The table above shows the results. If y is the percent battery charge on Xia's laptop, which linear equation represents the correct relationship between y and x ?Ay=1.5x+19By=2x+14Cy=2.5x+9Dy=10x+34y = 19.99 + 1.50x
The equation above models the total cost y, in dollars, that a company charges a customer to rent a truck for one day and drive the truck x miles. The total cost consists of a flat fee plus a charge per mile driven. When the equation is graphed in the xy-plane, what does the y-intercept of the graph represent in terms of the model?AA flat fee of $19.99BA charge per mile of $1.50CA charge per mile of $19.99DTotal daily charges of $21.49y=−75x+5,000
the equation above models the amount of money y, in dollars, remaining in Bo's bank account x days after the start of the fall semester. The amount of money in Bo's bank account is based on the money he earned over the summer and how much he spends per day during the fall semester. When the equation is graphed in the xy-plane , what does the slope of the graph represent in terms of the model?AThe total amount in Bo's bank accountBDaily spending of $5,000CDaily spending of $75DThe amount of money Bo earned over the summer
A lending library has a fixed charge for the first three days and an additional chargefor each day thereafter. Aarushi paid Rs 27 for a book kept for seven days. If fixed charges are Rs x and per day charges are Rs y. Write the linear equation representing the abvoe information.
View SolutionA number is 27 more than the number obtained by reversing its digits. If its unit's and tens digit are x and y respectively, write the linear equation representing the above statement.
View SolutionIn which of the following situations, does the list of numbers involved make an arithmetic progression, and why?
(i) The taxi fare after each km when the fare is Rs 15 for the first km and Rs 8 for each additional km.
(ii) The amount of air present in a cylinder when a vacuum pump removes 1/4 of the air remaining in the cylinder at a time.
(iii) The cost of digging a well after every metre of digging, when it costs Rs.150 for the first metre and rises by Rs. 50 for each subsequent metre.
(iv) The amount of money in the account every year, when 10000 is deposited at compound interest at 8 % per annum.
View SolutionThe sum of a two digit number and the number obtained by reversing the order of its digits is 121. If units and ten's digit of the number are x and y respectively, then write the linear equation representing the above statement.
View SolutionA start-up company opened with 8 employees. The company’s growth plan assumes that 2 new employees will be hired each quarter (every 3 months) for the first 5 years. If an equation is written in the form y = ax + b to represent the number of employees, y, employed by the company x quarters after the company opened, what is the value of b ?
View Solution
