A wheel has a radius of 18 inches. The distance, in inches, the wheel travels when it rotates through an angle of 23π radians is closest to which value?
45
37
13
11
The correct Answer is:B
To find the distance that a wheel travels when it rotates through an angle of 23π radians, we can use the formula for the length of an arc. The formula is given by:
Arc Length=θ×r
where:
- θ is the angle in radians,
- r is the radius of the circle.
1. Identify the given values:
- Radius r=18 inches
- Angle θ=23π radians
2. Substitute the values into the arc length formula:
Arc Length=(23π)×18
3. Calculate the arc length:
- First, multiply 18 by 23:
18×23=12
- Now, multiply by π:
Arc Length=12π
4. Approximate the value of 12π:
- Using π≈3.14:
12π≈12×3.14=37.68
5. Determine the closest value:
- The distance the wheel travels when it rotates through an angle of 23π radians is approximately 37.68 inches.
Final Answer:
The closest value to the distance the wheel travels is approximately 38 inches.
- θ is the angle in radians,
- r is the radius of the circle.
- Radius r=18 inches
- Angle θ=23π radians
Arc Length=(23π)×18
- First, multiply 18 by 23:
18×23=12
- Now, multiply by π:
Arc Length=12π
- Using π≈3.14:
12π≈12×3.14=37.68
- The distance the wheel travels when it rotates through an angle of 23π radians is approximately 37.68 inches.
The closest value to the distance the wheel travels is approximately 38 inches.
Topper's Solved these Questions
- ADDITIONAL TOPICS IN MATHBOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATHEXERCISE - Grib-In
32 Videos
- ADDITIONAL TOPICS IN MATHBOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATHEXERCISE - EXERCISE
45 Videos
- ADDITIONAL TOPICS IN MATHBOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATHEXERCISE - EXERCISE
45 Videos
- ADDITIONAL TOPICS IN MATH BOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATH EXERCISE - PRACTICE TEST
24 Videos
- COMPLEX NUMBERSBOOK - ENGLISH SATCHAPTER - COMPLEX NUMBERSEXERCISE - EXERCISE
6 Videos
Similar Questions
When the axes are rotated through an angleπ2, find the new coordinates of the point(α,0)
View Solution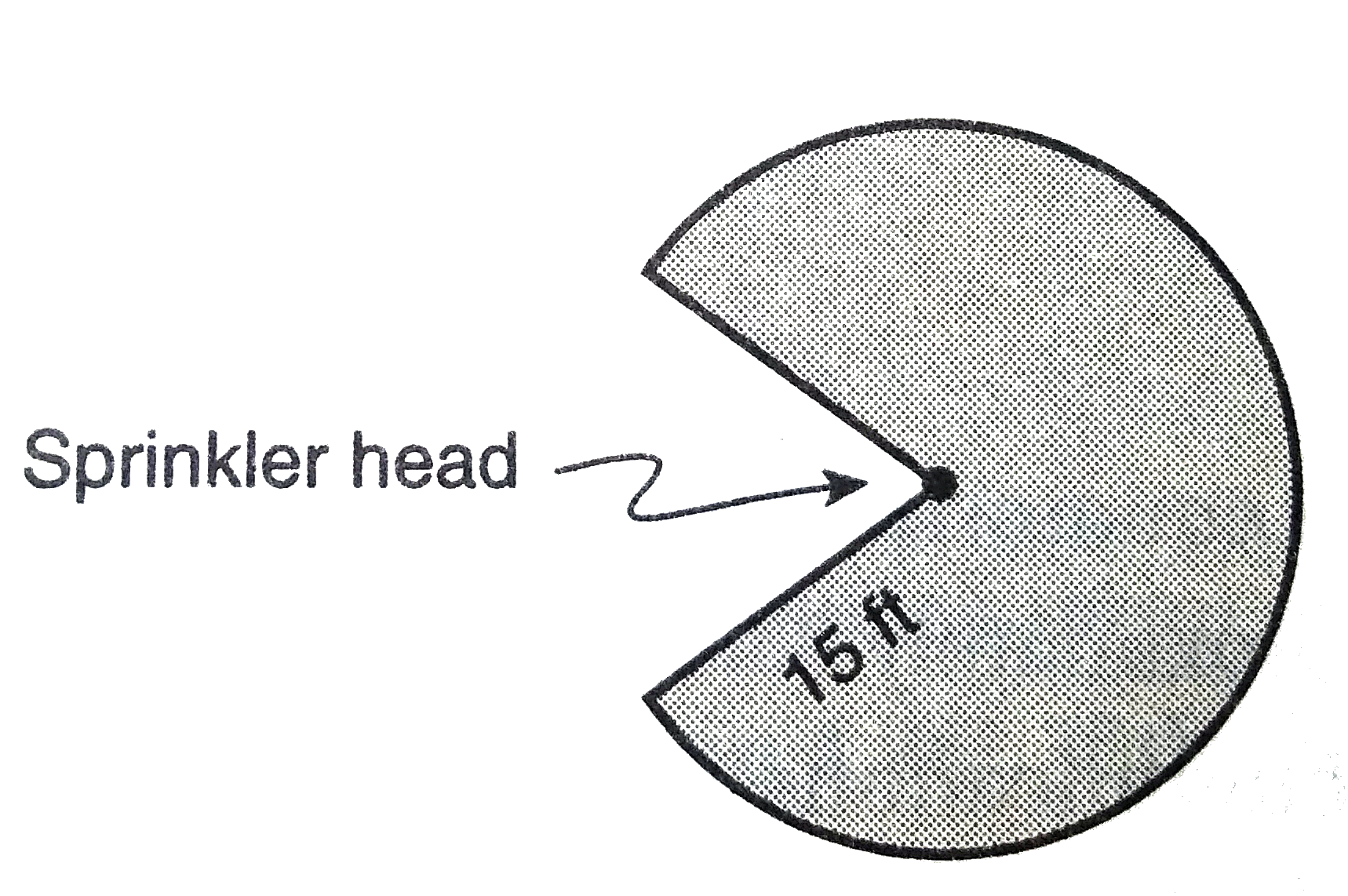
A lawn sprinkler sprays water in a circular pattern at a distance of 15 feet from the sprinkler head which rotates through the angle of 5π3 radians, as shown by the shaded area in the diagram above. What is the area of the lawn, to the nearest square foot, that recieves water from this sprinkler?
View Solution
Knowledge Check
A wedge shaped piece is cut from a circular pizza. The radius of the pizza is 14 inches and the angle of the pointed end of the pizza measures 0.35 radians. The number of inches in the length of the rounded edge of the crust is closest to which values?
A4.0B4.9C5.7D7.5The bottom of a pendulum traces an arc 3 feet in length when the pendulum swings through an angle of 12 radians.What is the number of feet in the length of the pendulum?
A1.5B6C1.5πD6πA circle with a diameter of 8 inches is divided into a number of equal sectors such that each sector has an area of 4π3 square inches. What is the central angle of each sector, in radians
Aπ12Bπ6Cπ3D2π3
Find the area of the sector and length of the arc subtended by a central angle of 2π3 radians in a circle whose radius is 6 inches.
View SolutionThe transformed equation of x2+6xy+8y2=10 when the axes are rotated through an angled π/4 is
View SolutionA wheel is subjected to uniform angular acceleration about its axis with its angular velocity is zero . In the first two seconds , it rotates through an angle θ1 and in the next two seconds , it rotates through an angle θ2 then the ratio of θ2/θ1
View SolutionIf the coordiantes of a point P are transformed to (2,−4√3) when the axes are rotated through an angle 60∘, then P=
View SolutionA formula for the volume of a right circular cone is V=13πr2h, where r is the radius of the base and h is the height of the cone. Using 227 as an approximate value for π, which of the following values is closest to the volume, in cubic inches, of a cone with height 28 inches and radius 6 inches?
View Solution
