A window of a house is h metres above the ground. From the window, the angles of elevation and depression of the top and the bottom of another house situated on the opposite sides of the lane are found to be αandβ respectively. Prove that the height of the other house is h(1+tanαcotβ) metres.Solution in Gujrati
Step by step video & image solution for A window of a house is h metres above the ground. From the window, the angles of elevation and depression of the top and the bottom of another house situated on the opposite sides of the lane are found to be alpha and beta respectively. Prove that the height of the other house is h(1 + tan alpha cot beta) metres. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - KUMAR PRAKASHANCHAPTER - SOME AAPLICATIONS OF TRIGONOMETRYEXERCISE - Practice Thoroughly7videos
SOME AAPLICATIONS OF TRIGONOMETRY
- BOOK - KUMAR PRAKASHANCHAPTER - SOME AAPLICATIONS OF TRIGONOMETRYEXERCISE - Objective Questions24videos
SOME AAPLICATIONS OF TRIGONOMETRY
- BOOK - KUMAR PRAKASHANCHAPTER - SOME AAPLICATIONS OF TRIGONOMETRYEXERCISE - Exercise9.116videos
SOME AAPLICATIONS OF TRIGONOMETRY
- BOOK - KUMAR PRAKASHANCHAPTER - REAL NUMBERS EXERCISE - TEST YOUR SKILLS26videos
REAL NUMBERS
- BOOK - KUMAR PRAKASHANCHAPTER - STATISTICSEXERCISE - Objective Questions (True or false)5videos
STATISTICS
Similar Questions
A jet plane is at a vertical height of h. The angles of depression of two tanks on the ground in the same line with the plane are αandβ(α>β). Prove that the distance between the tanks is h(tanα−tanβ)tanαtanβ
03:48View SolutionA kite is flying at a height of 75m from the level ground, attached to a taut string inclined at 60∘ to the horizontal. Then, the length of the string is …………m
04:28View SolutionA circus artist is climbing a 20m long rope, which is tightly stretched and tied from the top of a vertical pole to the ground. Find the height of the pole, if the angle made by the rope with the ground level is 30∘ (see the given figure)
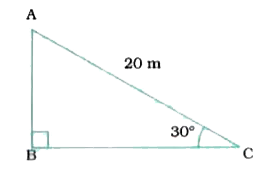 02:58View Solution
02:58View SolutionShow that the square of any positive integer is of the form 4m or 4m + 1 for some integer m.
05:20View SolutionFor any frequency distribution, mean is always greater than mode.
03:09View SolutionFrom a window ( h meter above ground) the angle of elevation of other house is theta and angle of depression is phi. Find the height of the house.
06:14View SolutionFrom a window 15 metres high above the ground in a street, the angles of elevation and depression of the top and the foot of another house on the opposite side of the street are 300and450 respectively show that the height of the opposite house is 23.66 metres (take√3=1.732)
07:28View SolutionFrom the top of a light house, the angles of depression of two ships on the opposite sides of its are observed to be αandβ . If the height of the light house be h metres and the line joining the ships passes through the foot of the light house, show that the distance between the ship is (htanα+tanβtanαtanβ
07:15View SolutionFrom a window, 60 metres high above the ground, of a house in a street, the angles of elevation and respectively. Show that the height of the opposite house is 60(√3+1) metres.
02:53View SolutionA window of a house is h metres above the ground. From the window, the angles of elevation and depression of the top and bottom of another house situated on the opposite side of the lane are found to be αandβ respectively. Prove that the height of the house is h(1+tanα⋅tanβ) metres.
04:33View SolutionFrom a window (h metres high above the ground) of a house in a street, the angle of elevation and depression of the top and the foot of another house on the opposite side of the street are θ and ϕ respectively. Show that the height of the opposite house ish(1+tanθcotϕ) metres.
05:04View SolutionFrom the bottom of a pole of height h the angle of elevation of the top of a tower is alpha and the pole subtends an angle beta at the top of the tower . Prove that the height of the tower is (h cot(alpha - beta))/(cot(alpha - beta) - cot alpha) .
03:20View SolutionFrom the tower 60 m high angles of depression of the top and bottom of a house are αnandβ respectively. If the height of the house be h. then prove that
h=60sin(β−α)cosαsinβ06:08View SolutionFrom a window 60 m high above the ground of a house in a street, the angle of elevation and depression of the top and the foot of another house on the opposite side of the street are 60∘ and 45∘ respectively. Show that the height of opposite house is 60(1+√3) metres.
06:51View SolutionFrom the top and bottom of a building of height h metres, the angles of elevation of the top of a tower are αandβ respectively. Then the height of the tower is :
07:09View Solution

