$A B$ is a chord of $y^{2} = 4 x$ such that normals at $A$ and $B$ intersect at $C (9, 6)$ and the tengent at A and B at point T, find ${(C T)}^{2} / 13$

Text Solution

Verified by Experts

The correct Answer is:A

Normal at A and B intersect at $C (9, 6)$ which lies on parabola. Equation of normal is $y = - t x + 2 t + t^{3}$ . Since it passes through C, we have
$t^{3} - 7 t - 6 = 0$
$∴ T_{1} + t_{2} + 3 = 0$ or $t_{1} + t_{2} = - 3$ ,
$t_{1} t_{2} t_{3} = 6$ , and $t_{1} t_{2} = 2$ .
Now, $A B = \sqrt{{(t_{1}^{2} - t_{2}^{2})}^{2} + {(2 t_{1} - 2 t_{2})}_{1}^{2}}$
$= \sqrt{{(t_{1} - t_{2})}_{1}^{2} [{(t_{1} + t_{2})}_{1}^{2} + 4]}$
$= \sqrt{[{(t_{1} + t_{2})}_{1}^{2} - 4 t_{1} t_{2}] [{(t_{1} + t_{2})}_{1}^{2} + 4]}$
$= \sqrt{[9 - 8] [9 + 4]} = \sqrt{13}$
Area of triangle $A B C = \frac{1}{2} ∣ ∣ ∣ ∣ ∣ \begin{matrix} t_{1}^{2} & 2 t_{1} & 1 t_{2}^{2} & 2 t_{2} & 1 9 & 6 & 1 \end{matrix} ∣ ∣ ∣ ∣ ∣$
$= \frac{1}{2} [18 (t_{1} - t_{2}) - 6 (t_{1}^{2} - t_{2}^{2}) + 2 t_{1} t_{2} (t_{1} - t_{2}]$
$= \frac{1}{2} | t_{1} - t_{2} | [18 - 6 (t_{1} + t_{2}) + 2 t_{1} t_{2}]$
= 20 sq. units
Equation of $A B : y - 2 t_{2} = \frac{2}{t_{1} + t_{2}} (x - t_{2}^{2})$
Or $(t_{1} + t_{2}) y - 2 t_{1} t_{2} - 2 t_{2}^{2} = 2 x - 2 t_{2}^{2}$ or $2 x + 3 y + 4 = 0$
Distance of AB from origin is $\frac{4}{\sqrt{13}}$ .
Line AB meets axis at $C (- 2, 0)$ and $D (0, - 4 / 3)$
$∴$ Area of $Δ O C D = 4 / 3$ sq. units

Updated on:21/07/2023

|

Class 12 MATHS PARABOLA

Topper's Solved these Questions

Similar Questions

Knowledge Check

If normals to parabola $y^{2} = 4 x$ at points A, B, C intersect at (11, 0), then

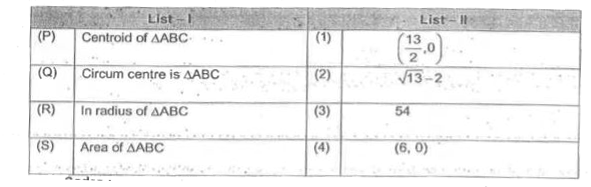A $\begin{matrix} P & Q & R & S 2 & 1 & 4 & 3 \end{matrix}$
B $\begin{matrix} P & Q & R & S 4 & 1 & 2 & 4 \end{matrix}$
C $\begin{matrix} P & Q & R & S 4 & 1 & 2 & 3 \end{matrix}$
D $\begin{matrix} P & Q & R & S 2 & 4 & 1 & 3 \end{matrix}$
Let A and B be two points on $y^{2} = 4 a x$ such that normals to the curve at A and B meet at point C, on the curve, then chord AB will always pass through a fixed point whose coordinates, are
A(2a, 0)
B(-a, 0)
C(-2a, 0)
D(a, 0)
Line $x - y = 1$ intersect the parabola $y^{2} = 4 x$ at A and B. Normals at A and B intersect at C. If D is the point at which line CD is normal to the parabola, then coordinate of point D is
A $(4, - 4)$
B(4,4)
C $(- 4, - 4)$
DNone of these

CENGAGE PUBLICATION-PARABOLA-Matching Column Type

AB is a chord of y^2=4x such that normals at A and B intersect at C(9,...
Text Solution

Home
Ask Doubt
Profile