AB is an equilateral triangle inscribed in a circle of centre O and radius 5cm. Let the diameter through C meet the circle again at D.
Assertion (A) : AD.BD<OB.OC
Reason (R ) : 2(AD2+BD2)=CD2=100sqcm
Both A and R are individually true and R is the correct explanation of A
Both A and R are individually true but R is not the correct explanation of A
A is true but R is false
A is false but R is true
The correct Answer is:D

(A) : Consider AD−BD<OB.OC
Now, OA=5cm (Radius) , OB=5cm (Radius) and
OC=5cm (Radius)
Since, ΔOAD and ΔOAD
are congruent by SAS therefore
AD=OA=5cm
and BD=OB=5cm
thus, AD.BD=5×5=25
and OB.BC=5×5=25
Thus, we have
AD.BD=25=OB.OC
Now (R): 2(AD2+BD2)
=2[25+25]=100
and CD2=(10)2=100
Thus, 2(AD2+BD2)=CD2=100sq.cm.
Hence , (A) is false and (R) is true.
Topper's Solved these Questions
Similar Questions
ABC is an equilateral triangle inscribed in a circle with AB = 5 cm. Let the bisector of the angle A meet BC in X and the circle in "Y. What is the value of AX.AY?
View SolutionIn the given figure, ABC is an equilateral triangle inscribed in a circle of radius 4 cm with centre O, then the area of the shaded region is :
View Solution
Knowledge Check
A ABC is inscribed in a circle with centre o and radius 5 cm and AC is a diameter of the circle. If AB = 8 cm, then BC = ......... cm.
A10B8C6D15Triangle ABC inscribes a circle with centre O and inradius √6cm. The inradius OD divides side BC at D, such that BD=2 cm and DC=8 cm. Find the ratio AB:AC.
A4:7B5:7C4:5Dcannot be determinedA tangent AB at a point A of a circle of radius 5 cm meets a line through the centre O at a point B so that OB = 12 cm , Length AB is
A10 cmB12 cmC9 cmD√119 cm
In Fig. 19.60, ABC is an equilateral triangle inscribed in a circle of radius 4 cm with centre O . Find the area of the shaded region. (FIGURE)
View SolutionABC is an equilateral triangle of side 6 cm, a circle, centre O, passes through the vertices A, B, and C. Find the radius of the circle.
View SolutionIn the figure, ABC is a triangle inscribed in a circle with centre O.
If AB = AC = 15 cm and BC = 5 cm, calculate the radius of the circle.
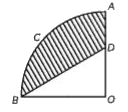
View SolutionIn figure, OACB is a quadrant of a circle with centre O and radius 7 cm. If AD = 2 cm, find the area of the shaded region.
View SolutionA tangent AB, at a point A of a circle of radius 5 cm, meets a line through the centre O at point B such that OB =12 cm. Length AB is
View Solution
