ABC is a right-angled triangle and O is the mid-point of the side opposite to the right angle. Explain why O is equidistant from A, B and C.
Opposite sides are parallel and equal to each other and all the interior angles are right angles.
The property of rectangle states that the diagonals are of equal length and bisect each other.
Hence, AO = OC = BO = OD.
Thus, O is equidistant from A, B and C.
Topper's Solved these Questions
Similar Questions
ABC is a a right angled triangle and O is the mid point of the side opposite to the right angle. Explain Why O is equidistant from A,B and C. (The dotted lines are drawn additionally to help you).

View SolutionProve that the mid-point of the hypotenuse of right angled triangle is equidistant from its vertices.
View Solution
Knowledge Check
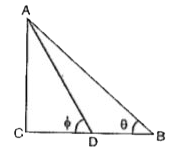
ABC is right angled triangle, right angled at C . D is the midpoint of BC. Then , tanθtanϕ =
 A1B1√2C12D2
A1B1√2C12D2If ABC is a right angled triangle at B and M, N are the mid-points of AB and BC, then 4(AN2+CM2) is equal to :
A4AC2B6AC2C5AC2D54AC2a and b are two side adjacent to the right angle of a right-angled triangle and p is the perpendicular drawn to the hypotenuse from the opposite vertex. Then p2 is equal to
Aa2+b2B1a2+1b2Ca2b2a2+b2Da2−b2
Prove that the mid-point of the hypotenuse of a right triangle is equidistant from its vertices
View SolutionIn Fig. 14.40, a right triangle BOA is given. C is the mid-point of the hypotenuse AB . Show that it is equidistant from the vertices O, A and B . (FIGURE)
View SolutionIn a triangle ABC, right angled at B, then
View SolutionIn a right -angle triangle, the length of the sides containing the right angles area aandb. With the mid-point of each sides as centres three semicircles are drawn outiside the triangle. Find the area of Δ and the semicircles together
View SolutionABC is right triangle , right angled at A, then tanB⋅tanC=_____
View Solution
