ABC is a triangular park with AB = AC = 100 m. A block tower is situated at the midpoint of BC.The angles of elevation of the top of the tower at A and B are cot−1(3.2) and cosec−1(2.6) respectively.The height of the tower is:
In the figure DP is a clock tower of height 'h' standing at D, the midpoint of BC.
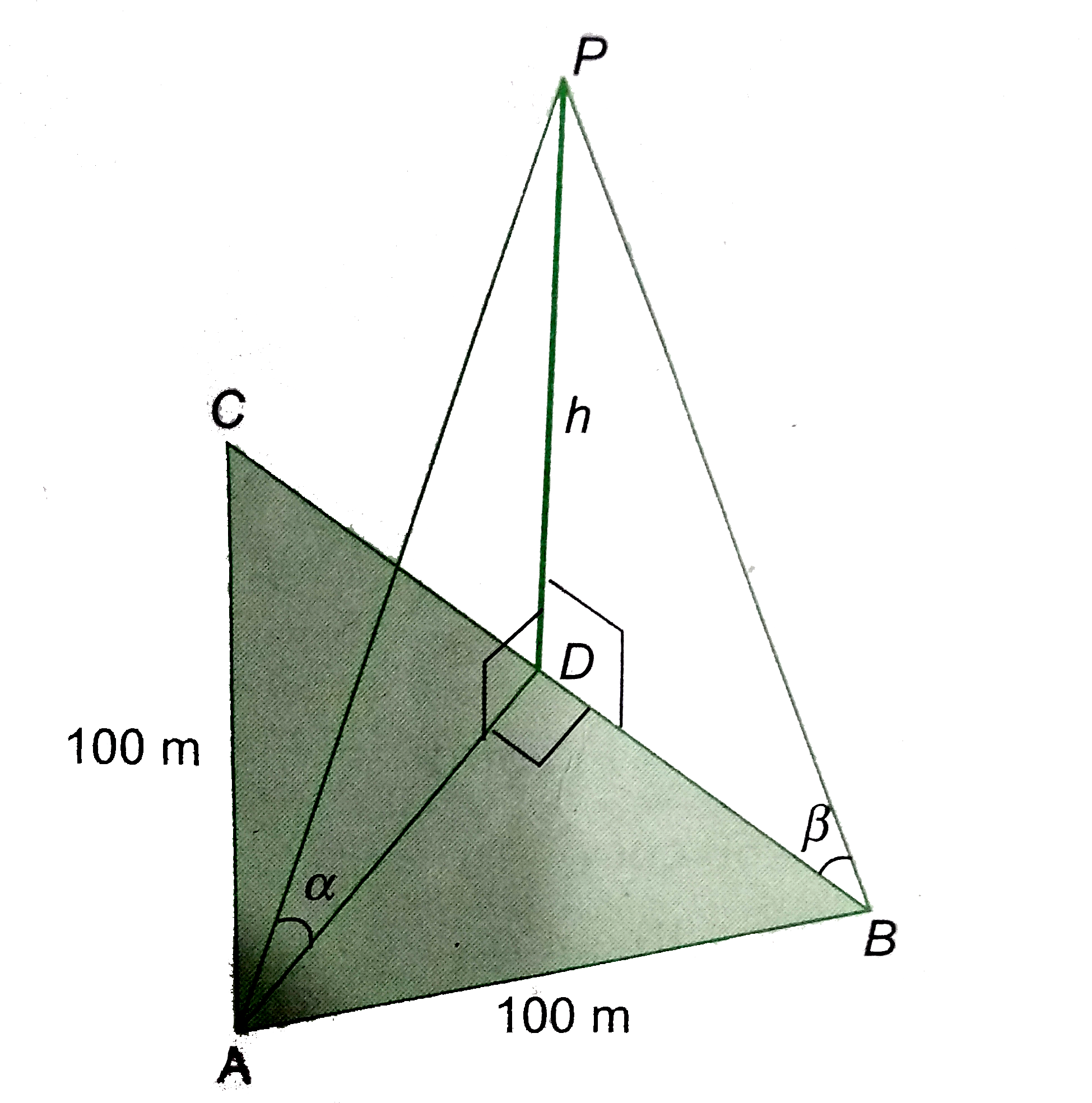
since AB=AC ,AD is perpendicular to BC. In triangle ADP.
tanα=hAD
⇒ADcotα
In triangle PDB ,
tanβ=hBD
⇒BD=hcotβ
Now ,in right triangle ADB,
AB2+AD2+BD2
⇒ 1002=h2cot2α+h2cot2+β
⇒ 1002=h2[cot2α+(cosec2β−1)]
⇒ 1002=h2[(3.2)2+(2.6)2−1)]
⇒ 1002=16h2
⇒ h2=625
⇒ h=25m
Topper's Solved these Questions
- HIGHT AND DISTANCE BOOK - CENGAGECHAPTER - HIGHT AND DISTANCE EXERCISE - Exercise
18 Videos
- HIGHT AND DISTANCE BOOK - CENGAGECHAPTER - HIGHT AND DISTANCE EXERCISE - JEE Previous Year
3 Videos
- GRAPHS OF TRIGONOMETRIC FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise
22 Videos
- HYPERBOLABOOK - CENGAGECHAPTER - HYPERBOLAEXERCISE - JEE Advanced Previous Year
14 Videos
Similar Questions
ABC is a triangular park with AB=AC=100m. A clock tower is situated at the mid-point of BC. The angle of elevation, If the top of the tower at A and B are cot−13.2 and cosec−12.6, respectively. The height of the tower is
View SolutionABC is a triangular park with AB = AC = 100 metres. A vertical tower is situated at the mid-point of BC. If the angles of elevation of the top of the tower at A and B are cot−1(3√2) and cosec−1(2√2) respectively, then the height of the tower (in metres) is :
View Solution
Knowledge Check
ABC is a triangular park with AB+AC=100 m. a clock tower is situated at the mid-point of BC. The angles of elevation of the top of the tower at A and B are cot−1 3⋅2andcosec−1 2⋅6 respectively. The height of the tower is
A16 mB25 mC50 mDNoneABC is a triangular park with AB = AC = 100 m. A vertical tower is situated at the mid-point of BC. If the angles of elevation of the top of the tower at A and B are cot−1(3√2) and cosec−1(2√2) respectively, then the height of the tower (in m) is
A25B20C10√5D1003√3ABC is a triangular park with AB = AC = 100 metres. A vertical tower is stiuated at the mid-point of BC. If the angles of elevation of the top of the tower at A and B are cot−1(3√2) and cosec−1(2√2) respectively,then the height of the tower (in meters) is :
A1003√3B10√5C20D25
ABC is a triangular park with AB=AC=100m. A Television tower stands at the mid point of BC. The angles of elevations of the top of tower at A,B,C are 45∘,60∘,60∘ respectively Then the height of tower is
View SolutionPQR is a triangular park with PQ=PR=200m . A T.V tower stands at the mid-point of QR. If the angles of elevation of the top of the tower at P , Q and R respectively 45∘ , 30∘ and 30∘ then the height of the tower in m is
View SolutionPQR is a triangular park with PQ=PR=200m . A T.V tower stands at the mid-point of QR. If the angles of elevation of the top of the tower at P , Q and R respectively 45∘ , 30∘ and 30∘ then the height of the tower in m is
View SolutionPQR is a triangular park with PQ=PR=200m . A T.V tower stands at the mid-point of QR. If the angles of elevation of the top of the tower at P , Q and R respectively 45∘ , 30∘ and 30∘ then the height of the tower in m is
View SolutionThe angles of elevation of the top of a tower at the top and the foot of a pole 10 m high are 30∘and60∘ respectively. The height of the tower is
View Solution
