ABC is an isosceles triangle in the given circle with centre O. ∠ABC=420, ∠CDE is equal to

84∘
138∘
96∘
148∘
The correct Answer is:C
Step by step video, text & image solution for ABC is an isosceles triangle in the given circle with centre O. angle ABC = 42 ^0, angle CDE is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 7 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CIRCLES BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - CIRCLES EXERCISE - SELF ASSESSMENT SHEET
10 Videos
- CIRCLES BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - CIRCLES EXERCISE - UNIT TEST
20 Videos
- CIRCLES BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - CIRCLES EXERCISE - UNIT TEST
20 Videos
- AVERAGE BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - AVERAGE EXERCISE - UNIT TEST -3
20 Videos
- CIRCUMFERENCE AND AREA OF A CIRCLE BOOK - S CHAND IIT JEE FOUNDATIONCHAPTER - CIRCUMFERENCE AND AREA OF A CIRCLE EXERCISE - SELF ASSESSMENT SHEET - 22
10 Videos
Similar Questions
In figure , ABC is the triangle inscribed in a circle with center O. show that angle A + angle OBC=90∘
View SolutionIf △ABC is an isosceles triangle with AB = AC and ∠ABC is 65∘, then ∠BCA and ∠BAC are, respectively
यदि △ABC, AB = AC और ∠ABC=65∘ के साथ एक समद्विबाहु त्रिभुज है, तो क्रमशः ∠BCA और ∠BAC है:
View Solution
Knowledge Check
ABC is an isosceles triangle in the given circle with centre O. ∠ABC=420, ∠CDE is equal to
 A84∘B138∘C96∘D148∘
A84∘B138∘C96∘D148∘O is the circumcentre of the △ABC. If ∠BAC=50∘, then ∠OBC is equal to
A30∘B60∘C40∘D50∘In △ABC ,AB=AC , ∠B=40∘ Then ∠C is equal to
A50∘B40∘C80∘D140∘
In figure ABC is an isosceles triangle , right angled at C . Therefore

View SolutionABC is an isosceles right angle Δ . If Perimeter is P find Area
View SolutionIn Δ ABC, O is the circum centre of the circle. If ∠OBC=37∘ then ∠BAC is equal to :
View SolutionO is the centre of a circle ∠AOB=90∘and∠BOC=120∘.∠ABC is equal to
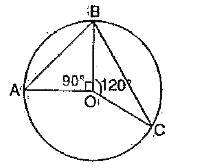
View SolutionIf an equilateral triangle ABC is inscribed in a circle with centre O then the measure of ∠AOB is
View Solution
