A,B,C,D develop 18 items. Five items jointly by A and C, four items by A and D, four items by B and C and five items by B and D. The number of ways of selecting eight ites out of 18 so that the selected ones belong equally to A,B,C,D is
5226
5626
4418
4936
The correct Answer is:B
To solve the problem step by step, we need to analyze the contributions of each pair of developers (A, B, C, D) to the total of 18 items and then find the number of ways to select 8 items such that they are equally distributed among A, B, C, and D.
Step 1: Understand the contributions of each pair
We have the following contributions:
- Items developed by A and C: 5 items
- Items developed by A and D: 4 items
- Items developed by B and C: 4 items
- Items developed by B and D: 5 items
Step 2: Set up the selection criteria
We need to select 8 items such that:
- 2 items from A and C
- 2 items from A and D
- 2 items from B and C
- 2 items from B and D
Step 3: Calculate the combinations for each case
We will calculate the number of ways to choose 2 items from each pair of developers.
1. Choosing 2 items from A and C (5 items):
Ways=(52)
2. Choosing 2 items from A and D (4 items):
Ways=(42)
3. Choosing 2 items from B and C (4 items):
Ways=(42)
4. Choosing 2 items from B and D (5 items):
Ways=(52)
Step 4: Calculate the total number of ways
The total number of ways to select the items is the product of the combinations calculated in Step 3:
Total Ways=(52)×(42)×(42)×(52)
Step 5: Substitute the values and calculate
Now, we will calculate each combination:
- (52)=10
- (42)=6
Now substituting these values into the total ways:
Total Ways=10×6×6×10
=10×10×36=100×36=3600
Step 6: Consider other combinations
We also need to consider other distributions of items among A, B, C, and D. The other cases include:
1. Choosing 3 from A and C, 1 from A and D, 1 from B and C, and 3 from B and D.
2. Choosing 4 from A and C, 0 from A and D, 4 from B and C, and 0 from B and D.
3. And so on...
Final Calculation
After considering all cases, we sum up all the ways calculated from each case.
The final answer after summing all valid cases will yield:
Total Ways=5626
Conclusion
Thus, the number of ways of selecting 8 items out of 18 so that the selected ones belong equally to A, B, C, and D is 5626.
We have the following contributions:
- Items developed by A and C: 5 items
- Items developed by A and D: 4 items
- Items developed by B and C: 4 items
- Items developed by B and D: 5 items
We need to select 8 items such that:
- 2 items from A and C
- 2 items from A and D
- 2 items from B and C
- 2 items from B and D
We will calculate the number of ways to choose 2 items from each pair of developers.
Ways=(52)
2. Choosing 2 items from A and D (4 items):
Ways=(42)
Ways=(42)
Ways=(52)
The total number of ways to select the items is the product of the combinations calculated in Step 3:
Total Ways=(52)×(42)×(42)×(52)
Now, we will calculate each combination:
- (52)=10
- (42)=6
Total Ways=10×6×6×10
=10×10×36=100×36=3600
We also need to consider other distributions of items among A, B, C, and D. The other cases include:
1. Choosing 3 from A and C, 1 from A and D, 1 from B and C, and 3 from B and D.
2. Choosing 4 from A and C, 0 from A and D, 4 from B and C, and 0 from B and D.
3. And so on...
After considering all cases, we sum up all the ways calculated from each case.
Total Ways=5626
Thus, the number of ways of selecting 8 items out of 18 so that the selected ones belong equally to A, B, C, and D is 5626.
Topper's Solved these Questions
- PERMUTATION AND COMBINATIONBOOK - CENGAGE ENGLISHCHAPTER - PERMUTATION AND COMBINATIONEXERCISE - Multiple Correct Answer
2 Videos
- PERMUTATION AND COMBINATIONBOOK - CENGAGE ENGLISHCHAPTER - PERMUTATION AND COMBINATIONEXERCISE - Comprehension
8 Videos
- PARABOLABOOK - CENGAGE ENGLISHCHAPTER - PARABOLAEXERCISE - Matching Column Type
1 Videos
- PRINCIPLE OF MATHEMATICAL INDUCTIONBOOK - CENGAGE ENGLISHCHAPTER - PRINCIPLE OF MATHEMATICAL INDUCTIONEXERCISE - Sovled Examples
22 Videos
Similar Questions
Knowledge Check
Of all the articles in a box, 80% are satisfactory, while 20% are not. The probability of
obtaining exactly five good items out of eight randomly selected articles isA0.003B0.013C0.132D0.147Of all the articles in a box, 80% are satisfactory, while 20% are not. The probability of
obtaining exactly five good items out of eight randomly selected articles isA0.003B0.013C0.132D0.147A manufacturer sells an item to B at Rs.12,000. B sells it to Cand C to D. If the rate of GST is 18% and profit at each stage of selling is Rs. 2,000, then the price including GST, paid by D is:
ARs.17,440BRs.16,000CRs.16,760DRs.18,880
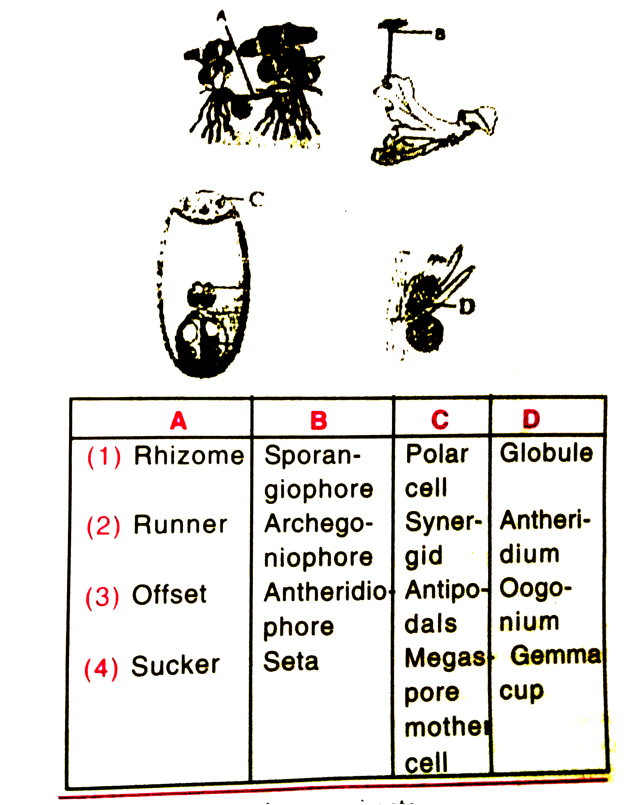
Examine the figure A,B,C and D. In which one of the four options all the items A,B,C and D are correct

View SolutionExamine the figure A,B,C and D. In which one of the four options all the items A,B,C and D are correct

View SolutionThe number of times a particular item occurs in a given data is called its variation (b) frequency (d) class-size
View SolutionIn Figure, find the four angles A, B, C and D in the parallelogram ABCD
View SolutionIf a,b,c ,d are four distinct numbers such that : a+c=2d and b+d=2c , Which of the following is the correct expression for the average of the four numbers ?
View Solution