ABCD is a rectangle with length 1 dm and breadth 42 m. The diagonal AC is drawn. Find area of ABCD.
As we know, 1dm=0.1m ⇒10m8dm=10.8m
Area of ABCD=Area of(△ADC)+Area(△ABC)
Area of triangle =12×base×height
⇒Area of ABCD =12×10.8×42+12×10.8×42 =10.8×42=453.6m2.
Topper's Solved these Questions
- BOOK - RD SHARMACHAPTER - LINEAR EQUATION IN ONE VARIABLEEXERCISE - All Questions107videos
LINEAR EQUATION IN ONE VARIABLE
- BOOK - RD SHARMACHAPTER - MENSURATION-II (VOLUMES AND SURFACE AREAS OF A CUBOID AND A CUBE)EXERCISE - All Questions134videos
MENSURATION-II (VOLUMES AND SURFACE AREAS OF A CUBOID AND A CUBE)
Similar Questions
Knowledge Check
- Question 1 - Select One
ABCD is a rectangle. Find x.
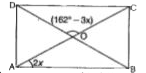 A54∘B36∘C24∘D18∘
A54∘B36∘C24∘D18∘ - Question 2 - Select One
The length of a rectangle is 1 cm more than its breadth. The diagonal is 29 cm . Find the area of the rectangle.
A481cm2B841cm2C420cm2D870m2 - Question 3 - Select One
The length of a rectangle is 1 cm more than its breadth. The diagonal is 29 cm . Find the area of the rectangle.
A481cm2B841cm2C420cm2D870m2
The length of a rectangle is greater than twice its breadth by 2 cm. The length of its diagonal is 13 cm. Find the length and breadth of the rectangle.
View SolutionFind the diagonal of a rectangle whose length is 35cm and breadth is 12cm.
 View Solution
View SolutionIn the given figure ABCD is a rectangle inscribed in a circle having length 8 cm and breadth 6 cm. If π=3.14 then the area of the shaded region is
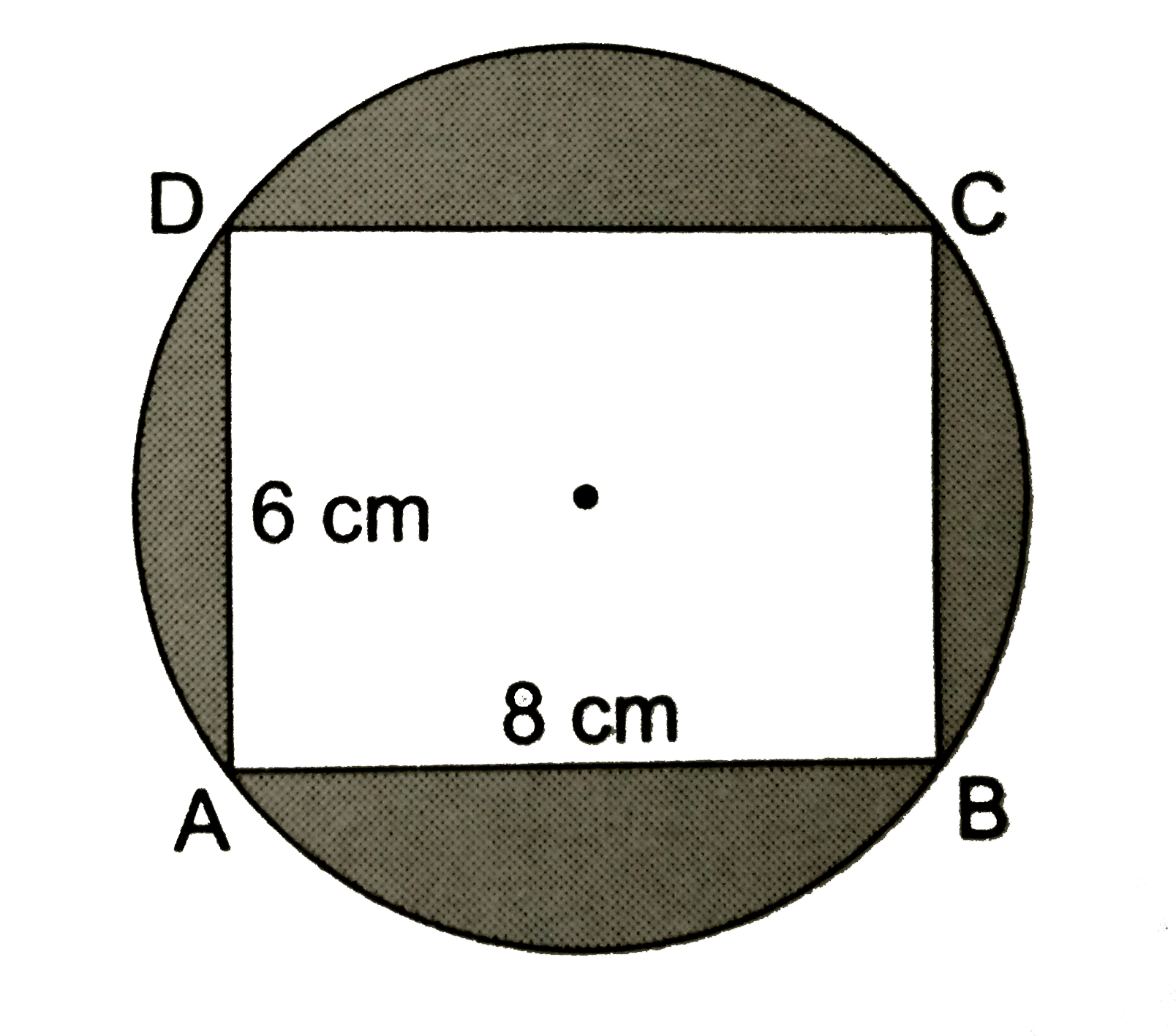 View Solution
View SolutionFind the perimeter of a rectangle in which:
(i) length=16.8 cm and breadth =6.2 cm
(ii) length=2m 25cm and breadth=1m 50cm
(iii) length=8m 5dm and breadth =6m 8 dmView SolutionABCD is a rectangle in which diagonal AC bisects ∠A as well as ∠C. Show that
(i) ABCD is a square
(ii) diagonal AD bisects ∠B
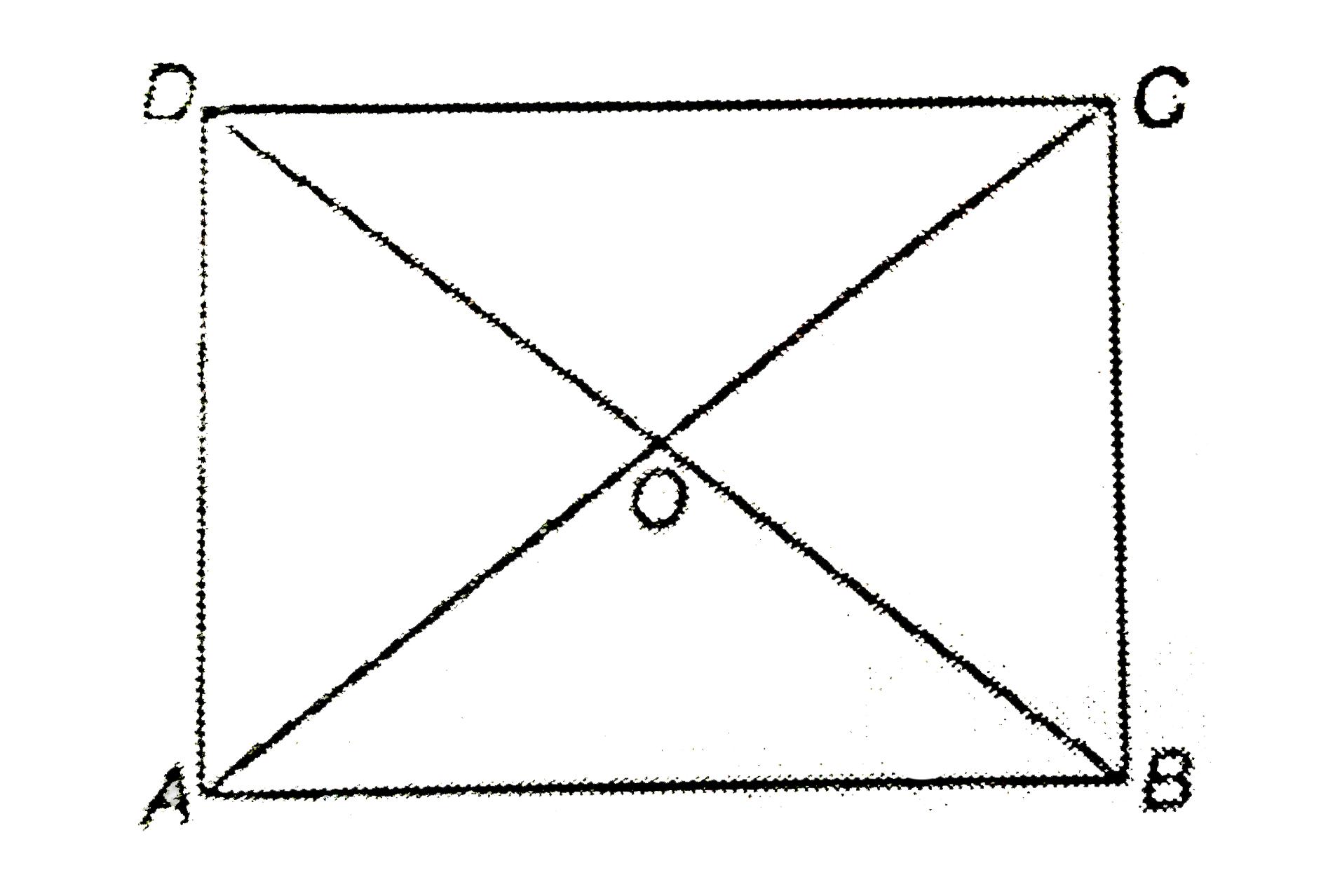 View Solution
View Solution


