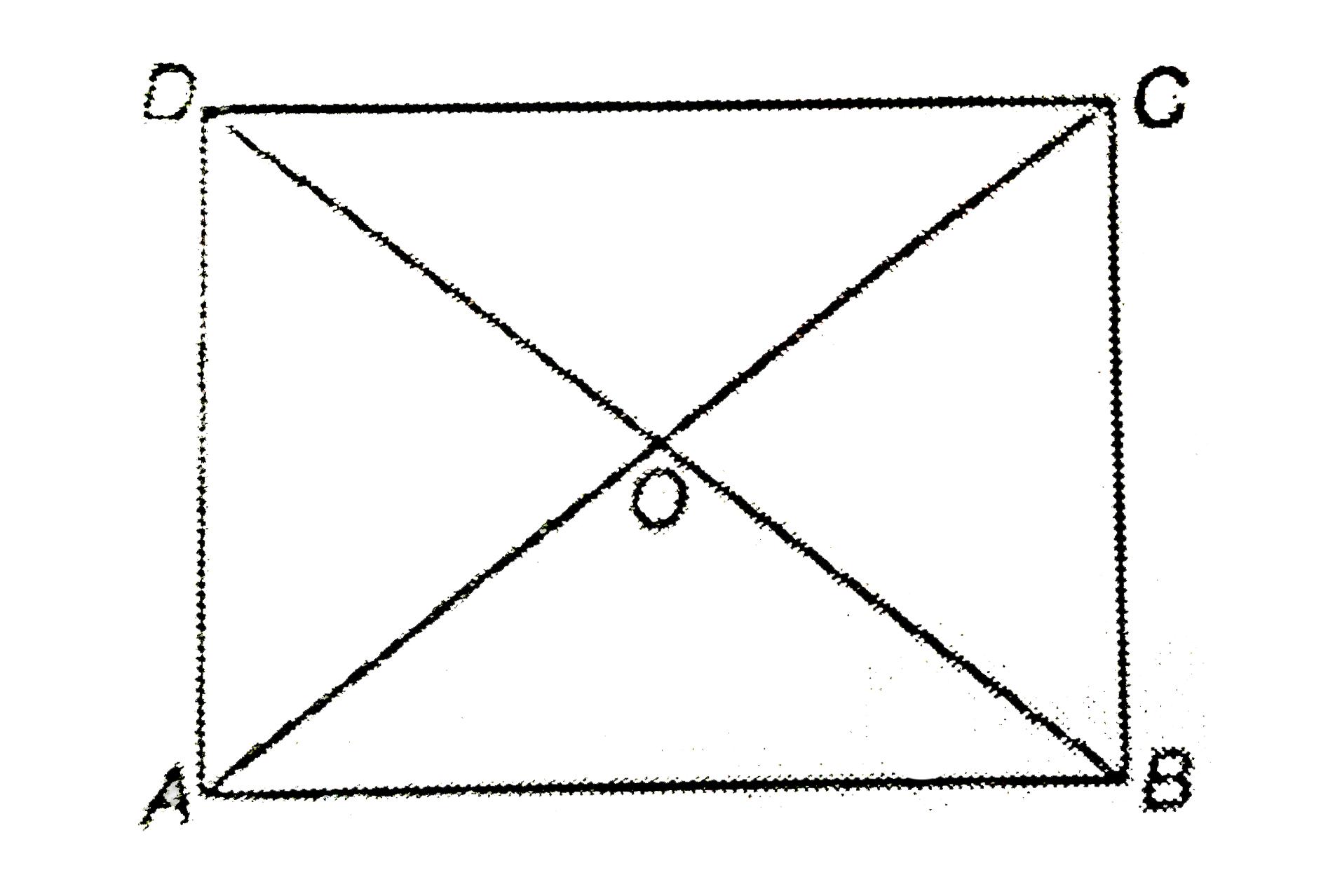
ABCD is a rhombus. Show that diagonal AC bisects ∠Aas well as ∠Cand diagonal BD bisects ∠Bas well as ∠D.
To prove that the diagonals of rhombus ABCD bisect the angles at the vertices, we will follow these steps:
Step 1: Understand the Properties of a Rhombus
A rhombus is a type of quadrilateral where all four sides are equal in length. The diagonals of a rhombus bisect each other at right angles and also bisect the angles at the vertices.
Step 2: Identify the Triangles
Consider triangles ABC and ADC formed by diagonal AC, and triangles ABD and BCD formed by diagonal BD.
Step 3: Prove Triangle Congruence
1. In triangle ABC:
- AB = AD (since all sides of a rhombus are equal)
- BC = CD (again, since all sides are equal)
- AC = AC (common side)
By the Side-Side-Side (SSS) congruence criterion, triangle ABC is congruent to triangle ADC.
Step 4: Conclude about Angles A and C
Since triangles ABC and ADC are congruent, we have:
- ∠BAC = ∠DAC
This means that diagonal AC bisects ∠A and ∠C.
Step 5: Repeat for the Other Diagonal
1. Now consider triangles ABD and BCD:
- AB = AD (sides of rhombus)
- BC = CD (sides of rhombus)
- BD = BD (common side)
By the SSS congruence criterion, triangle ABD is congruent to triangle BCD.
Step 6: Conclude about Angles B and D
Since triangles ABD and BCD are congruent, we have:
- ∠ABD = ∠CBD
This means that diagonal BD bisects ∠B and ∠D.
Final Conclusion
Thus, we have shown that:
- Diagonal AC bisects ∠A and ∠C.
- Diagonal BD bisects ∠B and ∠D.
A rhombus is a type of quadrilateral where all four sides are equal in length. The diagonals of a rhombus bisect each other at right angles and also bisect the angles at the vertices.
Consider triangles ABC and ADC formed by diagonal AC, and triangles ABD and BCD formed by diagonal BD.
1. In triangle ABC:
- AB = AD (since all sides of a rhombus are equal)
- BC = CD (again, since all sides are equal)
- AC = AC (common side)
Since triangles ABC and ADC are congruent, we have:
- ∠BAC = ∠DAC
This means that diagonal AC bisects ∠A and ∠C.
1. Now consider triangles ABD and BCD:
- AB = AD (sides of rhombus)
- BC = CD (sides of rhombus)
- BD = BD (common side)
Since triangles ABD and BCD are congruent, we have:
- ∠ABD = ∠CBD
This means that diagonal BD bisects ∠B and ∠D.
Thus, we have shown that:
- Diagonal AC bisects ∠A and ∠C.
- Diagonal BD bisects ∠B and ∠D.
Topper's Solved these Questions
Similar Questions
ABCD is a rectangle in which diagonal AC bisects ∠Aas well as ∠C. Show that:(i) ABCD is a square (ii) diagonal BD bisects ∠Bas well as ∠D..
View SolutionABCD is a rectangle in which diagonal AC bisects ∠A as well as ∠C . Show that: (i) ABCD is a square (ii) diagonal BD bisects ∠B as well as ∠D. .
View SolutionABCD is a rectangle in which diagonal AC bisects ∠A as well as ∠C. Show that
(i) ABCD is a square
(ii) diagonal BD bisects ∠B
 View Solution
View SolutionEach diagonal of a rhombus bisects it.
View SolutionShow that the diagonals of a rhombus bisect each other at right angles.
View SolutionABCD is a rhombus with ∠ABC=560. Determine ∠ACD.
View SolutionABCD is a rectangle in which diagonal BD bisects ∠B. Show that ABCD is a square.
View SolutionShow that each diagonal of a rhombus bisects the angle through which it passes.
View SolutionABCD is a rhombus. Its diagonals ACandBD intersect at the point M and satisfy BD=2AC.If he point DandM represent the complex numbers 1+iand2−i respectively, then A represents the complex number......or......
View SolutionIn a cyclic quadrilateral ABCD the diagonal AC bisects the angle BCD. Prove that the diagonal BD is parallel to the tangent to the circle at point A.
View Solution

