α,β are roots of y2−2y−7=0 find,
(i) α2+β2 (ii) α3+β3 Solution in Tamil
The correct Answer is:(i) α2+β2=18 (ii) α3+β3=50
Step by step video, text & image solution for alpha, beta are roots of y^(2)-2y-7=0 find, (i) alpha^(2)+beta^(2) " (ii) " alpha^(3)+beta^(3) by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- QUADRATIC EQUATIONSBOOK - CHETAN PUBLICATIONCHAPTER - QUADRATIC EQUATIONSEXERCISE - PRACTICE SET 2.6
10 Videos
- QUADRATIC EQUATIONSBOOK - CHETAN PUBLICATIONCHAPTER - QUADRATIC EQUATIONSEXERCISE - PROBLEM SET 2
50 Videos
- QUADRATIC EQUATIONSBOOK - CHETAN PUBLICATIONCHAPTER - QUADRATIC EQUATIONSEXERCISE - PRACTICE SET 2.4
10 Videos
- PYTHAGORAS THEOREMBOOK - CHETAN PUBLICATIONCHAPTER - PYTHAGORAS THEOREMEXERCISE - ASSIGNMENT -5
1 Videos
- SIMILARITY BOOK - CHETAN PUBLICATIONCHAPTER - SIMILARITY EXERCISE - ASSIGNMENT-1
12 Videos
Similar Questions
Product of Pragati's age 2 years ago and 3 years hence is 84. Find her present age.
View SolutionWhich of the following are quadratic equations?
x2+2x+11=0
View SolutionFind the values of a,b,c for the following quadratic equations by comparing with standard form :
x2−7x+5=0
View SolutionSolve the following sub question :
In an equilateral triangle ABC, the side BC is trisected at D. prove that 9AD2=7AB2
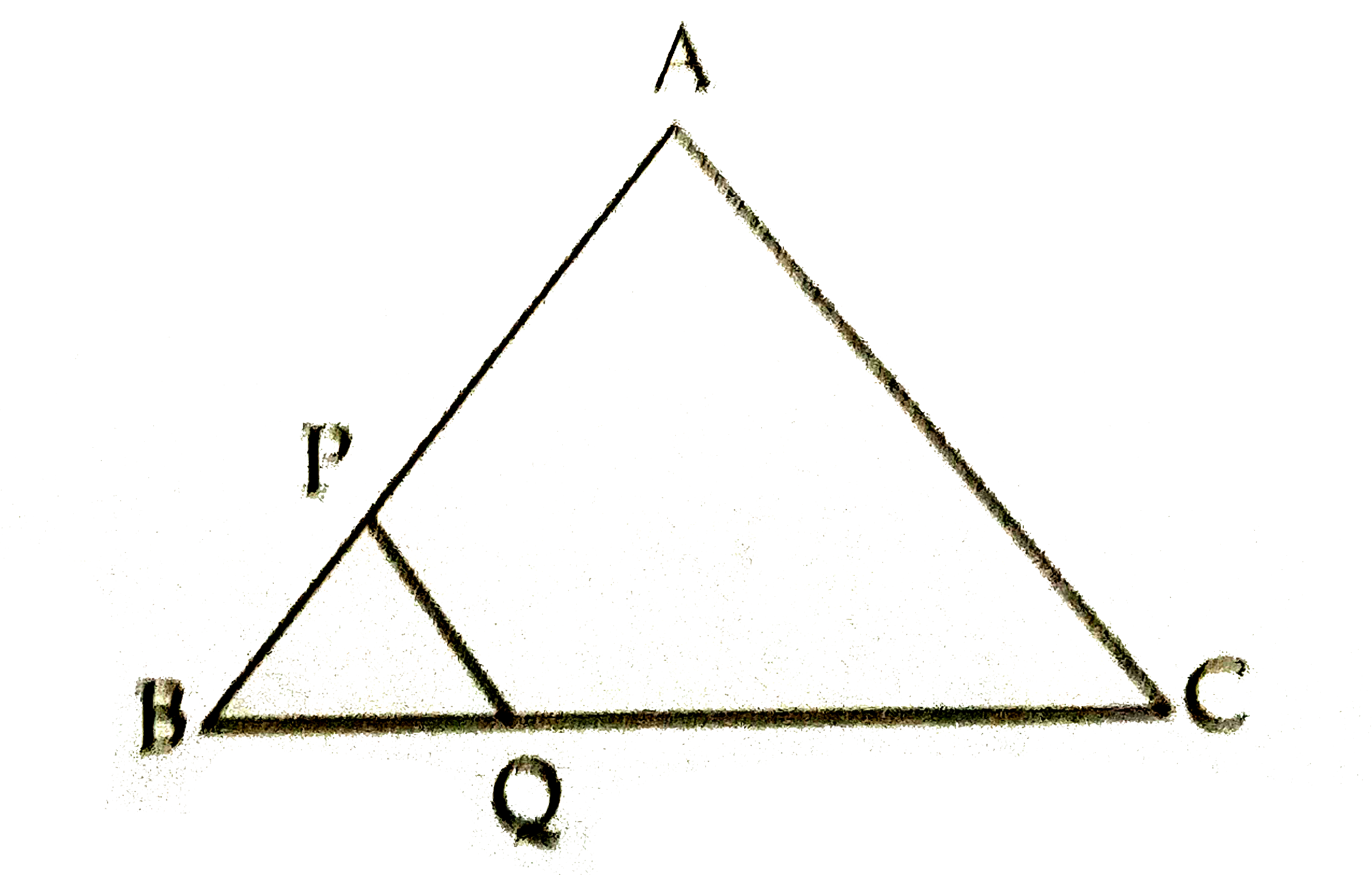
View SolutionSelect the appropriate alternative : In the adjoining figure ,PQ∣∣AC.BP=6,PA=8,BQ=9 , then QC = ……………
View SolutionIf α≠β and α2=5α−3andβ2=5β−3. find the equation whose roots are α/βandβ/α.
View SolutionIf alpha,beta are the roots of 2x^(2)+x+7=0 find the value of alpha^(2)beta+alpha beta^(2)
View SolutionIf alpha!=beta but alpha^(2)=5 alpha-3,beta^(2)=5 beta-3, then find the equation whose roots are (alpha)/(beta) and (beta)/(alpha)
View Solutionα,β are root of y2−2y−7=0 find α2+β2
View Solutionα,β are root of y2−2y−7=0 find α3+β3
View SolutionIf α and β are roots of x2+5x−1=0, then find (i)α3+β3(ii)α2+β2
View SolutionIf α and β are the roots of equation x2−4x+1=0, find (i) α2+β2 (ii) α3+β3
View SolutionIf α and β are the roots of the equation 3x2−5x+2=0, find the value of
(i) αβ+βα (ii) α−β (iii) α2β+β2α
View Solutionα,β are roots of y2−2y−7=0. Find α2+β2
View Solutionα,β are roots of y2−2y−7=0. Find α3+β3
View Solution
