Amisha has a square plot of side m and another triangular plot with base and height each equal to m। What is the total area of both plots?
To find the total area of Amisha's square and triangular plots, we will follow these steps:
Step 1: Calculate the area of the square plot.
The formula for the area of a square is given by:
Area of square=side2
Given that the side of the square is m:
Area of square=m2
Step 2: Calculate the area of the triangular plot.
The formula for the area of a triangle is given by:
Area of triangle=12×base×height
In this case, both the base and height of the triangle are equal to m:
Area of triangle=12×m×m=m22
Step 3: Add the areas of both plots to find the total area.
Now, we need to add the area of the square and the area of the triangle:
Total area=Area of square+Area of triangle
Substituting the areas we calculated:
Total area=m2+m22
Step 4: Find a common denominator to combine the terms.
To add these two terms, we can express m2 as a fraction with a denominator of 2:
m2=2m22
Now we can add:
Total area=2m22+m22=2m2+m22=3m22
Final Answer:
The total area of both plots is:
3m22
---
The formula for the area of a square is given by:
Area of square=side2
Given that the side of the square is m:
Area of square=m2
The formula for the area of a triangle is given by:
Area of triangle=12×base×height
In this case, both the base and height of the triangle are equal to m:
Area of triangle=12×m×m=m22
Now, we need to add the area of the square and the area of the triangle:
Total area=Area of square+Area of triangle
Substituting the areas we calculated:
Total area=m2+m22
To add these two terms, we can express m2 as a fraction with a denominator of 2:
m2=2m22
Now we can add:
Total area=2m22+m22=2m2+m22=3m22
The total area of both plots is:
3m22
Topper's Solved these Questions
- ALGEBRAIC EXPRESSIONSBOOK - NCERT EXEMPLARCHAPTER - ALGEBRAIC EXPRESSIONSEXERCISE - Think and Discuss
1 Videos
- ALGEBRAIC EXPRESSIONSBOOK - NCERT EXEMPLARCHAPTER - ALGEBRAIC EXPRESSIONSEXERCISE - Check understanding
1 Videos
- ALGEBRAIC EXPRESSIONSBOOK - NCERT EXEMPLARCHAPTER - ALGEBRAIC EXPRESSIONSEXERCISE - Exercise(True or false)
20 Videos
- COMPARING QUANTITIESBOOK - NCERT EXEMPLARCHAPTER - COMPARING QUANTITIESEXERCISE - THINK AND DISCUSS
24 Videos
Similar Questions
Knowledge Check
The sides of a rectangular plot are in the ratio 5 : 4 and its area is equal to 500 sq m. The perimeter of the plot is
A80 mB100 mC90 mD95 mWhat is the area of the plot shown in the figure ?
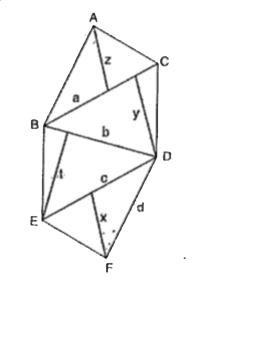 A12(az+by+ct+dx)B12(bt+cx+ay+az)C12(cx+bt+by+az)D12(d+t)(c+x)+12(a+b)(y+z)
A12(az+by+ct+dx)B12(bt+cx+ay+az)C12(cx+bt+by+az)D12(d+t)(c+x)+12(a+b)(y+z)Two plots of land have same perimeter. One is a square of side 40 m while the other is a rectangle whose breadth is 15m.
What is the area of the square plot ?A160m2B1600m2C1650m2D1720m2
The perimeter of a rectangular plot of land is 80 m and its breadth is 16 m . Find the length and area of the plot .
View SolutionArjun bought a rectangular plot with length x and breadth y and then sold a triangular part of it whose base is y and height is z. Find the area of the remaining part of the plot.
View SolutionIn exchange of a square plot one of whose sides is 84m, a man wants to buy a rectangular plot 144 m long and of the same areas of the square plot. Find the width of the rectangular plot.
View SolutionThe length of a rectangular plot of area 6513 m2 is 1214 m. What is the width of the plot?
View SolutionIf the perimeter of a rectangular plot is 34 metres and its area is 60 square metres, what is the length of each of the shorter side?
View Solution
