An ellipse has point (1,−1)and(2,−1) as its foci and x+y−5=0 as one of its tangents. Then the point where this line touches the ellipse is (329,229) (b) (239,29) (349,119) (d) none of these
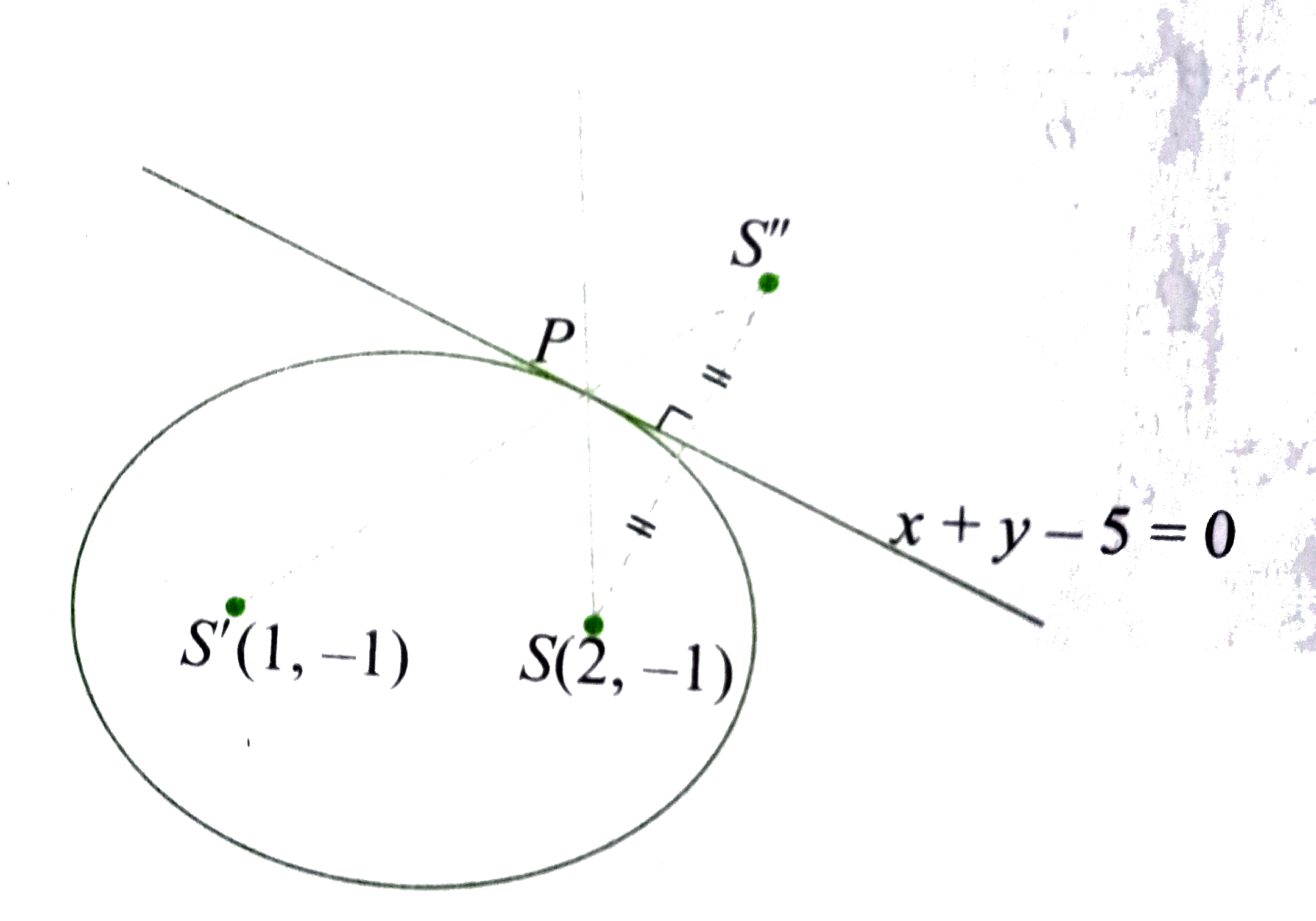
Image of focus S(2,-1) in the tangennt at P in the tangent at P is lies on the line S'P
Let image S'''≡(h,k)
∴h−21=k+11=2(2−2−5)2=4
⇒S'''=(6,3)
Equation of line SS'' is
4x-5y=9
Soving tangent and SS'' , we get P≡(349,119)
Similar Questions
An ellipse has point (1,−1)and(2,−1) as its foci and x+y−5=0 as one of its tangents. Then the point where this line touches the ellipse is (a) (329,229) (b) (239,29) (c) (349,119) (d) none of these
View SolutionAn ellipse has the points (1,−1)and(2,−1) as its foci and x+y=5 as one of its tangent then the value of a2+b2 where a,b are the lenghta of semi major and minor axes of ellipse respectively is :
View SolutionFind the equation of tangent at the point θ=π3 to the ellipse x29+y24=1
View SolutionShow that the point (9,4) lies outside the ellipse
x210+y29=1View SolutionFind the number of rational points on the ellipse x29+y24=1.
View SolutionFind the number of rational points on the ellipse x29+y24=1.
View SolutionThe normal to the curve 2x2+y2=12 at the point (2,2) cuts the curve again at (a) (−229,−29) (b) (229,29) (−2,−2) (d) none of these
View SolutionFind the equations of the tangents drawn from the point (2, 3) to the ellipse 9x2+16y2=144.
View SolutionFind the equations of the tangents drawn from the point (2, 3) to the ellipse 9x2+16y2=144.
View SolutionFind the equations of the tangents drawn from the point (2, 3) to the ellipse 9x2+16y2=144.
View Solution

