An ellipse has point (1,−1)and(2,−1) as its foci and x+y−5=0 as one of its tangents. Then the point where this line touches the ellipse is (329,229) (b) (239,29) (349,119) (d) none of theseSolution in Tamil
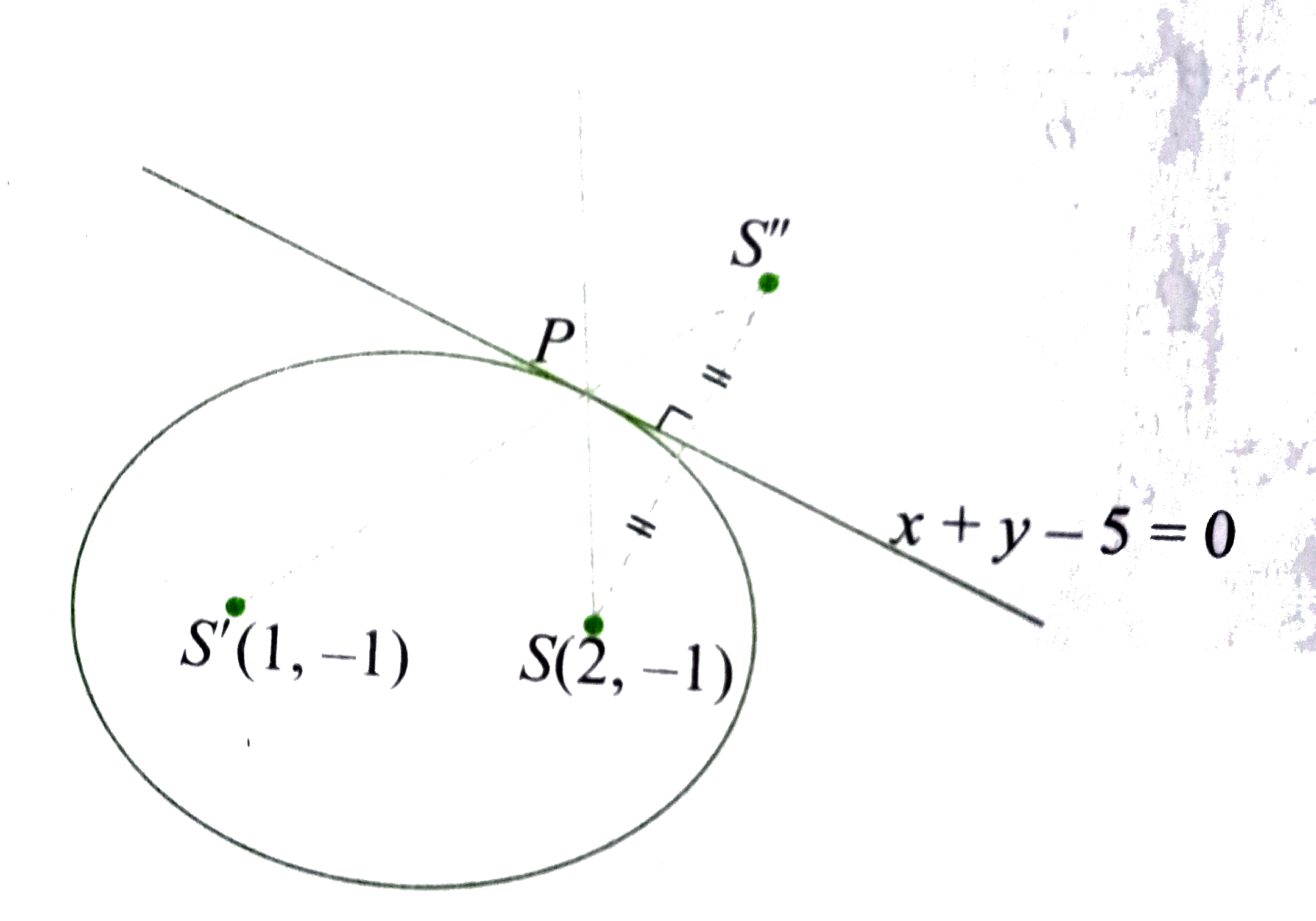
Image of focus S(2,-1) in the tangennt at P in the tangent at P is lies on the line S'P
Let image S'''≡(h,k)
∴h−21=k+11=2(2−2−5)2=4
⇒S'''=(6,3)
Equation of line SS'' is
4x-5y=9
Soving tangent and SS'' , we get P≡(349,119)
Similar Questions
An ellipse has point (1,−1)and(2,−1) as its foci and x+y−5=0 as one of its tangents. Then the point where this line touches the ellipse is (a) (329,229) (b) (239,29) (c) (349,119) (d) none of these
06:08View SolutionThe normal to the curve 2x2+y2=12 at the point (2,2) cuts the curve again at (a) (−229,−29) (b) (229,29) (−2,−2) (d) none of these
04:25View SolutionOn the ellipse 4x2+9y2=1, the point at which the tangent are parallel to 8x=9y are
05:36View SolutionThe coordinates of the foci of the ellipse x216+y29=1 will be-
View SolutionThe normal to the curve 2x2+y2=12 at the point (2,2) cuts the curve again at (A) (−229,−29) (B) (229,29) (C) (−2,−2) (D) none of these
08:00View SolutionAn ellipse has point (1,−1)and(2,−1) as its foci and x+y−5=0 as one of its tangents. Then the point where this line touches the ellipse is (a) (329,229) (b) (239,29) (c) (349,119) (d) none of these
04:26View SolutionThe line x=t meets the ellipse x^2+(y^2)/9=1 at real and distinct points if and only if. |t| 1 (d) none of these
01:26View SolutionTangents are drawn from the point P(3, 4) to the ellipse x^2/9+y^2/4=1 touching the ellipse at points A and B.
07:59View Solution

