Area lying in the first quadrant and bounded by the circle x2+y2=4 the line x=√3y and x-axis , is
A
π sq units
B
π2 sq units
C
π3 sq units
D
None of these
Video Solution
Text Solution
The correct Answer is:C
Intersection points of the curves are (0, 0) and ((√3,1)
Therefore,
Required area =∫10(x2−x1)dy=∫10(√4−y2−√3y)dy
=[12y√4−y2+12(4)sin−1.y2−√3y22]10
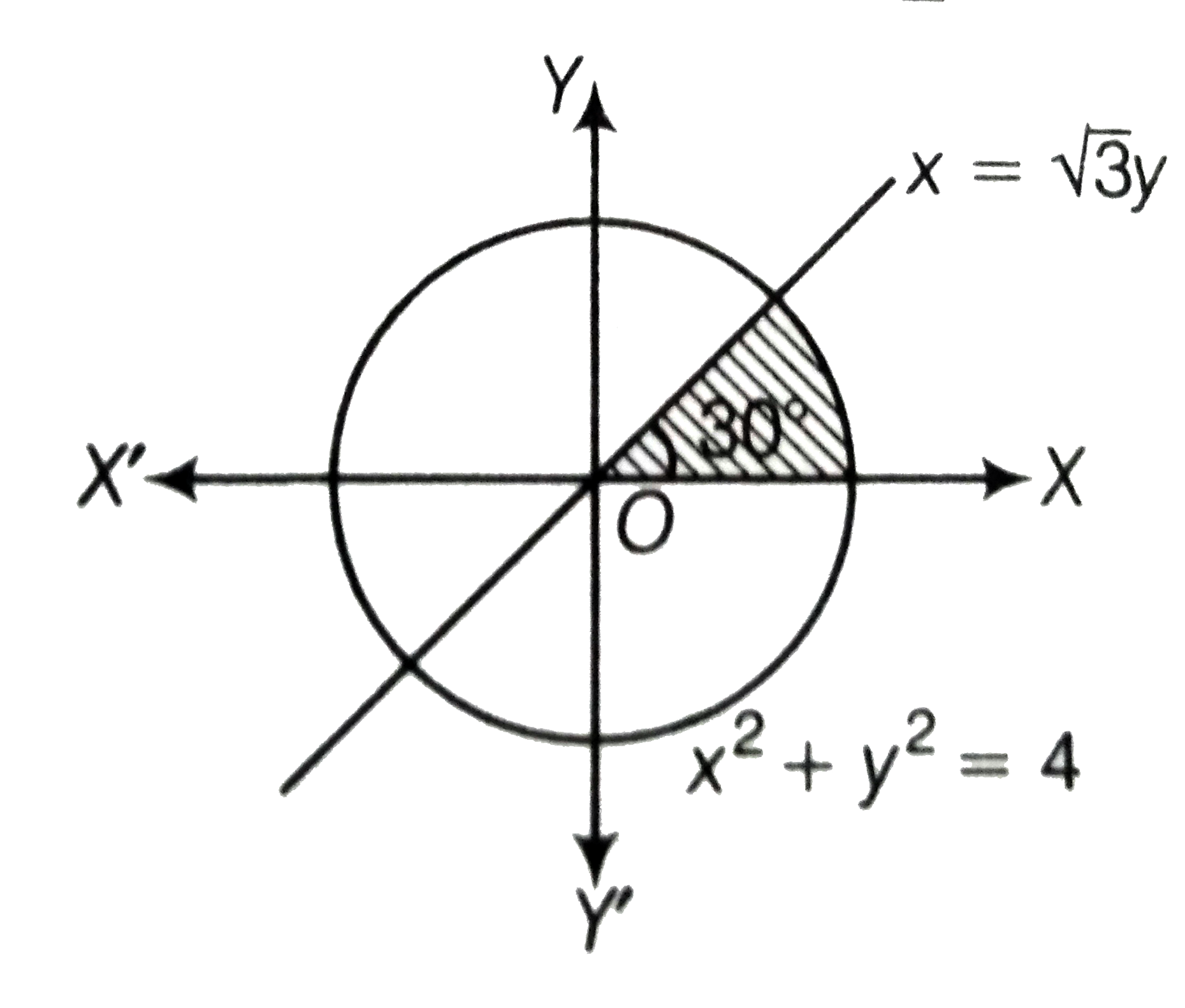
=√32+2sin−1(12)−√32−2sin−10
=π3 sq units
Alternate Method
Area =θ360∘×πr2=30360×π(2)2=π3 sq units
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - APPLICATIONS OF DEFINITE INTEGRALS EXERCISE - Exercise 234videos
APPLICATIONS OF DEFINITE INTEGRALS
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - APPLICATIONS OF DEFINITE INTEGRALS EXERCISE - MHT CET Corner6videos
APPLICATIONS OF DEFINITE INTEGRALS
- BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - MHT CET CORNER21videos
APPLICATIONS OF DERIVATIVES
Similar Questions
Knowledge Check
- Question 1 - Select One
Area lying in the first quadrant and bounded by the circle x2+y2=4 the line x=√3y and x-axis , is
AπBπ/2Cπ/3DNone of these - Question 2 - Select One
Area lying in the first quadrant and bounded by the circle x2+y2=4 and the lines x=0andx=2 is :
AπBπ2Cπ2Dπ4 - Question 3 - Select One
Area lying in the first quadrant and bounded by the circle x2+y2=4 and the lines x=0andx=2 is
AπBπ2Cπ3Dπ4.
In the first quadrant the area of the circle x2+y2=4 and the line x=0,x=2 is
View SolutionThe area lying in the first quadrant and bounded by the circle x2+y2=4 and the line x=√3y is :
View SolutionFind the area lying in the first quadrant and bounded by the curve y=x3 and the line y=4x.
View SolutionArea of smaller part in the first quadrant bounded by the semi-circle y=√4−x2, the line y=x√3 and X -axis, is
View SolutionThe area in the positive quadrant enclosed by the circle x2+y2=4, the line x=y√3 and X-axis is
View Solution

