At a distance of 100 feet, the angle of elevation from the horizontal ground to the top of a building is 42∘ .
The height of the building is
67 feet
74 feet
90 feet
110 feet
The correct Answer is:C
To find the height of the building based on the given angle of elevation and distance from the building, we can use trigonometric principles. Here’s a step-by-step solution:
Step 1: Understand the problem
We are given:
- The distance from the observer to the building (base) = 100 feet
- The angle of elevation (θ) = 42 degrees
We need to find the height of the building (h).
Step 2: Set up the trigonometric relationship
In a right triangle formed by the height of the building, the distance from the observer to the base of the building, and the line of sight to the top of the building, we can use the tangent function:
tan(θ)=oppositeadjacent
Here, the opposite side is the height of the building (h), and the adjacent side is the distance from the observer to the building (100 feet).
Step 3: Write the equation
Using the tangent function:
tan(42∘)=h100
Step 4: Solve for h
Rearranging the equation to solve for h gives:
h=100⋅tan(42∘)
Step 5: Calculate the value of h
Now we need to calculate tan(42∘). Using a calculator:
tan(42∘)≈0.9004
Now substituting this value back into the equation for h:
h≈100⋅0.9004≈90.04 feet
Step 6: Round the answer
Since we are looking for a practical answer, we can round this to:
h≈90 feet
Final Answer
The height of the building is approximately 90 feet.
---
We are given:
- The distance from the observer to the building (base) = 100 feet
- The angle of elevation (θ) = 42 degrees
In a right triangle formed by the height of the building, the distance from the observer to the base of the building, and the line of sight to the top of the building, we can use the tangent function:
tan(θ)=oppositeadjacent
Here, the opposite side is the height of the building (h), and the adjacent side is the distance from the observer to the building (100 feet).
Using the tangent function:
tan(42∘)=h100
Rearranging the equation to solve for h gives:
h=100⋅tan(42∘)
Now we need to calculate tan(42∘). Using a calculator:
tan(42∘)≈0.9004
Now substituting this value back into the equation for h:
h≈100⋅0.9004≈90.04 feet
Since we are looking for a practical answer, we can round this to:
h≈90 feet
The height of the building is approximately 90 feet.
Topper's Solved these Questions
Similar Questions
The angle of elevation of the top of a building from the foot of the tower is 30∘ and the angle of elevation of the top of the tower from the foot of the building is 45∘. If the tower is 30 m high, find the height of the building.
View SolutionThe angle of elevation of the top of a building from the foot of the tower is 30oand the angle of elevation of the top of the tower from the foot of the building is 60o. If the tower is 50 m high, find the height of the building.
View SolutionA 1.5m tall boy is standing at some distance from a 30m tall building. The angle of elevation from his eyes to the top of the building increases from 30∘ to 60∘ as he walks towards the building. Find the distance he walked towards the building.
View SolutionThe angle of elevation of the top of a building from the foot of the tower is 30° and the angle of elevation of the top of the tower from the foot of the building is 60°. If the tower is 60 m high, find the height of the building.
View SolutionFrom a point P on the ground the angle of elevation of the top of a 10 m tall building is 30o. A flag is hoisted at the top of the building and the angle of elevation of the top of the flagstaff from P is 45o. Find the length of the flagstaff
View SolutionThe angle of elevation of the top of the building from the foot of the tower is 30o and the angle of the top of the tower from the foot of the building is 60o . If the tower is 50m high, find the height of the building.
View SolutionA statue, 1.6 m tall, stands on the top of a pedestal. From a point on the ground, the angle of elevation of the top of the statue is 60∘and from the same point the angle of elevation of the top of the pedestal is 45∘. Find the height of the pedistal
View SolutionA statue 1.6m tall stands on the top of pedestal. From a point on the ground, the angle of elevation of the top of the statue is 60∘ and from the same point the angle of elevation of the top of the pedestal is 45∘ . Find the height of the pedestal.
View SolutionThe angle of elevation of the top of an incomplete vertical pillar at a horizontal distance of 100 m from its base is 45∘. If the angle of elevation of the top of the complete pillar at the same point is to be 60∘, then the height of the incomplete pillar is to be increased by
View SolutionIn the figure-2, the angle of elevation of the top of tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m. find the distance of the point from the foot of the tower.
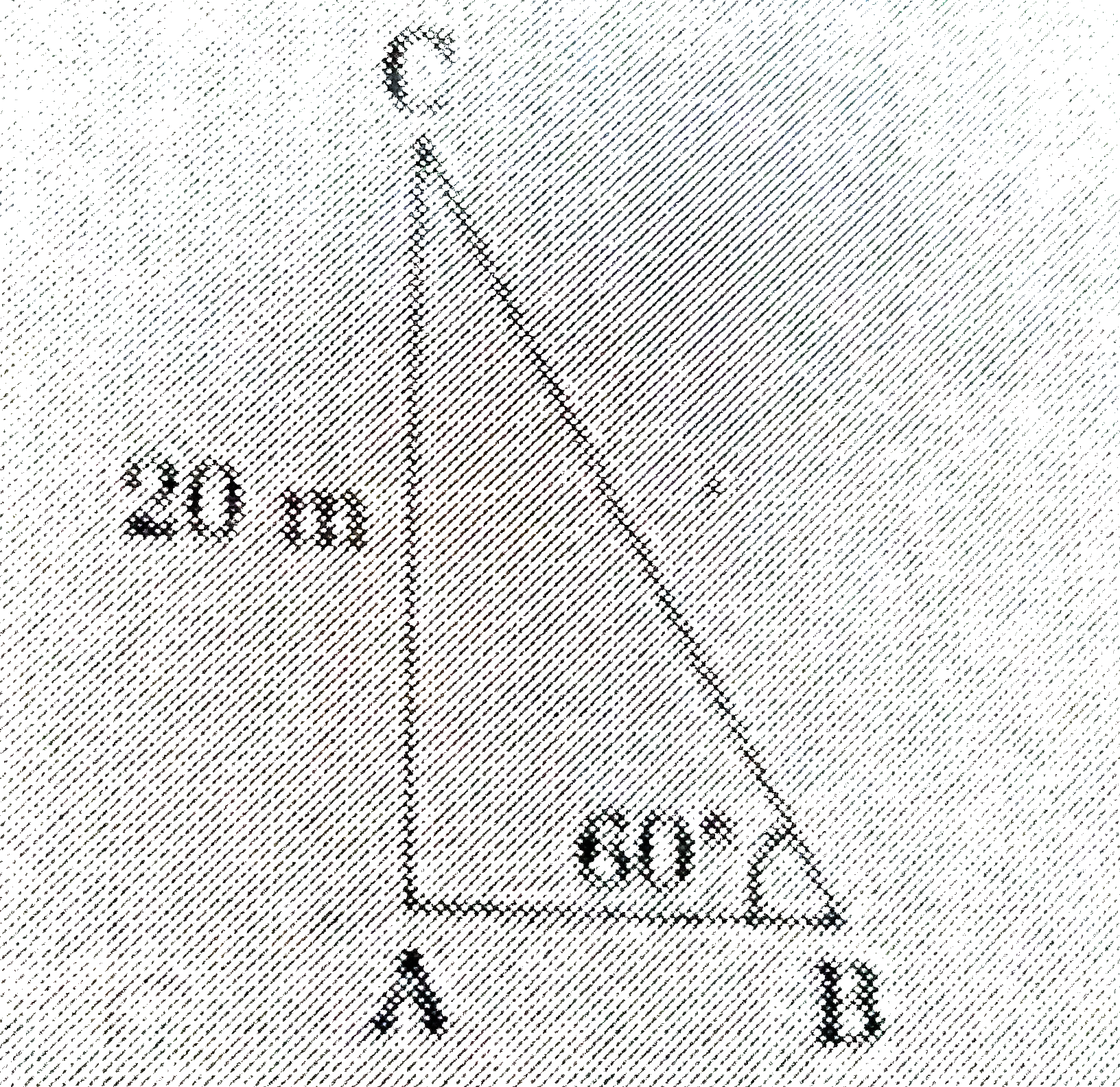
View Solution
