Atul is playing in a playground which is of the form of a parallelogram. He observes that the diagonals of the playground are 80 m and 60 m long. So, the playground can be the shape of ____
Rectangle
Rhombus
Kite
Square
The correct Answer is:B
To determine the shape of the playground that Atul is playing in, we need to analyze the properties of the diagonals of a parallelogram.
1. Understanding the Properties of Diagonals in Parallelograms:
- In a parallelogram, the diagonals bisect each other but are not necessarily equal in length.
2. Given Information:
- The lengths of the diagonals are 80 m and 60 m.
3. Identifying the Possible Shapes:
- The question states that the playground is in the form of a parallelogram. The common types of parallelograms are rectangles, rhombuses, and squares.
- In rectangles and squares, the diagonals are equal. Since the diagonals here are 80 m and 60 m (which are not equal), the playground cannot be a rectangle or a square.
4. Considering Rhombus and Kite:
- A rhombus is a type of parallelogram where the diagonals are not equal but they bisect each other at right angles.
- A kite is not a parallelogram, so it cannot be considered in this case.
5. Conclusion:
- Since the only remaining option that fits the description of having unequal diagonals while still being a parallelogram is a rhombus, we conclude that the shape of the playground must be a rhombus.
Final Answer:
The playground can be the shape of a rhombus.
- In a parallelogram, the diagonals bisect each other but are not necessarily equal in length.
- The lengths of the diagonals are 80 m and 60 m.
- The question states that the playground is in the form of a parallelogram. The common types of parallelograms are rectangles, rhombuses, and squares.
- In rectangles and squares, the diagonals are equal. Since the diagonals here are 80 m and 60 m (which are not equal), the playground cannot be a rectangle or a square.
- A rhombus is a type of parallelogram where the diagonals are not equal but they bisect each other at right angles.
- A kite is not a parallelogram, so it cannot be considered in this case.
- Since the only remaining option that fits the description of having unequal diagonals while still being a parallelogram is a rhombus, we conclude that the shape of the playground must be a rhombus.
The playground can be the shape of a rhombus.
Topper's Solved these Questions
- IMO QUESTION PAPER 2020-21 SET-ABOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - IMO QUESTION PAPER 2020-21 SET-AEXERCISE - ACHIEVERS SECTION
5 Videos
- IMO QUESTION PAPER 2020-21 SET-ABOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - IMO QUESTION PAPER 2020-21 SET-AEXERCISE - ACHIEVERS SECTION
5 Videos
- IMO QUESTION PAPER 2019-20 SET-BBOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - IMO QUESTION PAPER 2019-20 SET-BEXERCISE - ACHIEVERS SECTION
5 Videos
- LOGICAL REASONING BOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - LOGICAL REASONING EXERCISE - VERBAL REASONING
1 Videos
Similar Questions
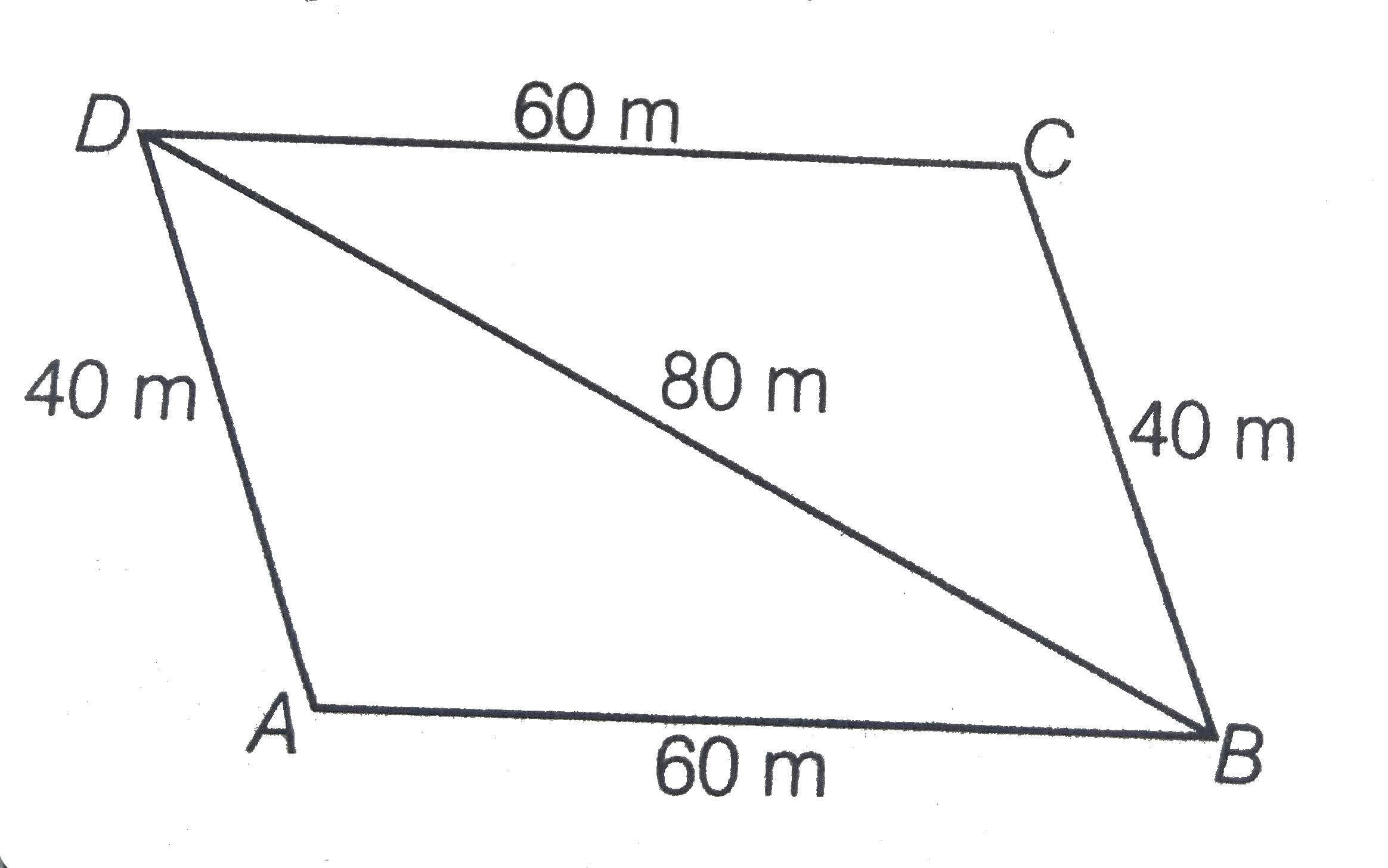
A field in the form of a parallelogram has sides 60 m and 40 m and one of its diagonals is 80 m long. Find the area of the parallelogram.
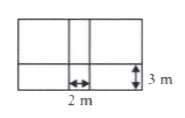
View SolutionA school playground is divided by a 2 m wide path which is parallel to the width of the playground and a 3 m wide path which is parallel to the length of the ground (see figure).
If the length and width of the playground are 120 m and 80 m respectively, then find the area of the remaining playground.
View Solution
Knowledge Check
A parallelogram has two sides 60 m and 25 m and a diagonal 65 m long. The area of the parallelogram is :
A1000 m2B1400 m2C1600 m2D1500 m2The diameter of a roller, 1 m long, is 84 cm. If it takes 500 complete revolutions to level a playground, the area of the playground is
A1440m2B1320m2C1260m2D1550m2A field in the form of a parallelogram has sides 60 m and 40 m and one of its diagonals is 80 m long. Find the area of the parallelogram.
 A480 sq. mB320 sq. mC600√15sq.mD450√15 sq . M
A480 sq. mB320 sq. mC600√15sq.mD450√15 sq . M
The area of a rectangular playground is 300 m2. The length of the playground is 2,000 cm. The width of the playground is
View SolutionA piece of land is in the form of a parallelogram and the perimeter of the land is 86 m. The length of one side exceeds the other by 13 m and one of the diagonals is 41 m.
What is the shorter height of the parallelogram?
View SolutionA piece of land is in the form of a parallelogram and the perimeter of the land is 86 m. The length of one side exceeds the other by 13 m and one of the diagonals is 41 m.
What is the area of the parallelogram ?
View SolutionThe length of a rectangular playground is 2m longer than its breadth. If the area of the playground is 195sq.m. Find the length and breadth of the field
View SolutionIn a circular park of diameter 80m, there is a square-shaped playground of maximum area. The area of the playground is
View Solution
