Calculate Kari Pearson's coefficient of correlation between the values of x and y for the following data :
n=10,∑x=55,∑y=40,∑x2=385,∑y2=192and∑(x+y)2=947
The correct Answer is:−0.68
To calculate the Karl Pearson's coefficient of correlation (r) between the values of x and y, we will use the following formula:
r=n∑xy−∑x∑y√(n∑x2−(∑x)2)(n∑y2−(∑y)2)
Step 1: Identify the given values
From the problem statement, we have:
- n=10
- ∑x=55
- ∑y=40
- ∑x2=385
- ∑y2=192
- ∑(x+y)2=947
Step 2: Find ∑xy
We know that:
∑(x+y)2=∑x2+∑y2+2∑xy
Substituting the known values:
947=385+192+2∑xy
This simplifies to:
947=577+2∑xy
Now, isolating ∑xy:
2∑xy=947−577
2∑xy=370
∑xy=3702=185
Step 3: Substitute values into the formula
Now that we have ∑xy=185, we can substitute all values into the formula for r:
r=10⋅185−55⋅40√(10⋅385−552)(10⋅192−402)
Step 4: Calculate the numerator
Calculating the numerator:
10⋅185=1850
55⋅40=2200
Numerator=1850−2200=−350
Step 5: Calculate the denominator
Calculating the denominator:
1. Calculate n∑x2−(∑x)2:
10⋅385−552=3850−3025=1825
2. Calculate n∑y2−(∑y)2:
10⋅192−402=1920−1600=320
3. Now, calculate the denominator:
Denominator=√1825⋅320
Calculating 1825⋅320:
1825⋅320=584000
√584000≈764.42
Step 6: Final Calculation of r
Now substituting back into the formula:
r=−350764.42≈−0.458
Final Answer
Thus, the Karl Pearson's coefficient of correlation r≈−0.458.
From the problem statement, we have:
- n=10
- ∑x=55
- ∑y=40
- ∑x2=385
- ∑y2=192
- ∑(x+y)2=947
We know that:
2∑xy=370
∑xy=3702=185
Now that we have ∑xy=185, we can substitute all values into the formula for r:
Calculating the numerator:
55⋅40=2200
Numerator=1850−2200=−350
Calculating the denominator:
√584000≈764.42
Now substituting back into the formula:
Thus, the Karl Pearson's coefficient of correlation r≈−0.458.
Topper's Solved these Questions
Similar Questions
Find the Karl Pearson's co-efficient of correlation between x and y for the following data :
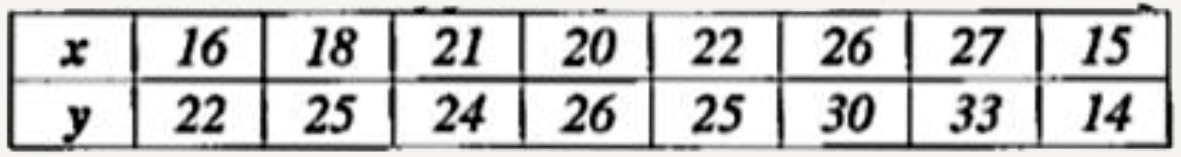
View SolutionFind the Karl Pearson's coefficient of correlation between X and Y for the following data :
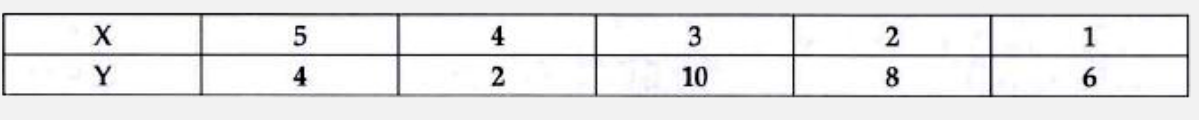
View SolutionCaculate Karl Pearson 's coefficient of correlation between X and Y from the following data :
Assume 80 and 130 as the mean values for X and Y respectively .
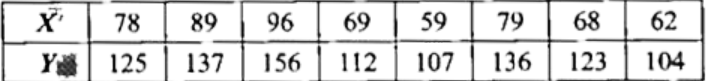
View SolutionFind the Karl Pearson's coefficient of correlation between x and y for the following data:
x1618212022262715y2225242625303314
View SolutionIf ∑x=50,∑y=−30,∑xy=115andn=10, the covariance is
View Solutionx and y are a pair of correlated variables . Ten observations of their values (xpyt) have the following result : ∑x=55,∑y=55,∑x2=385,∑xy=350. Predict the value of y when the value of x is 6
View SolutionThe sum of x2−y2andy2−x2 is
View SolutionFor a bivariate date, you are given following information:
∑x=125,∑x2=1650,∑y=100,∑y2=1500,∑xy=50,n=25
Find line of regression.
View SolutionFrom the following data find regression equation of y on x, Σx=55,Σy=88,Σx2=385,Σy2=1114,Σxy=586,n=10
View SolutionIf ∑x1=16,∑y1=48
∑(x1−3)(y1−4)=22andn=2, find the cov (x,y).
View Solution
