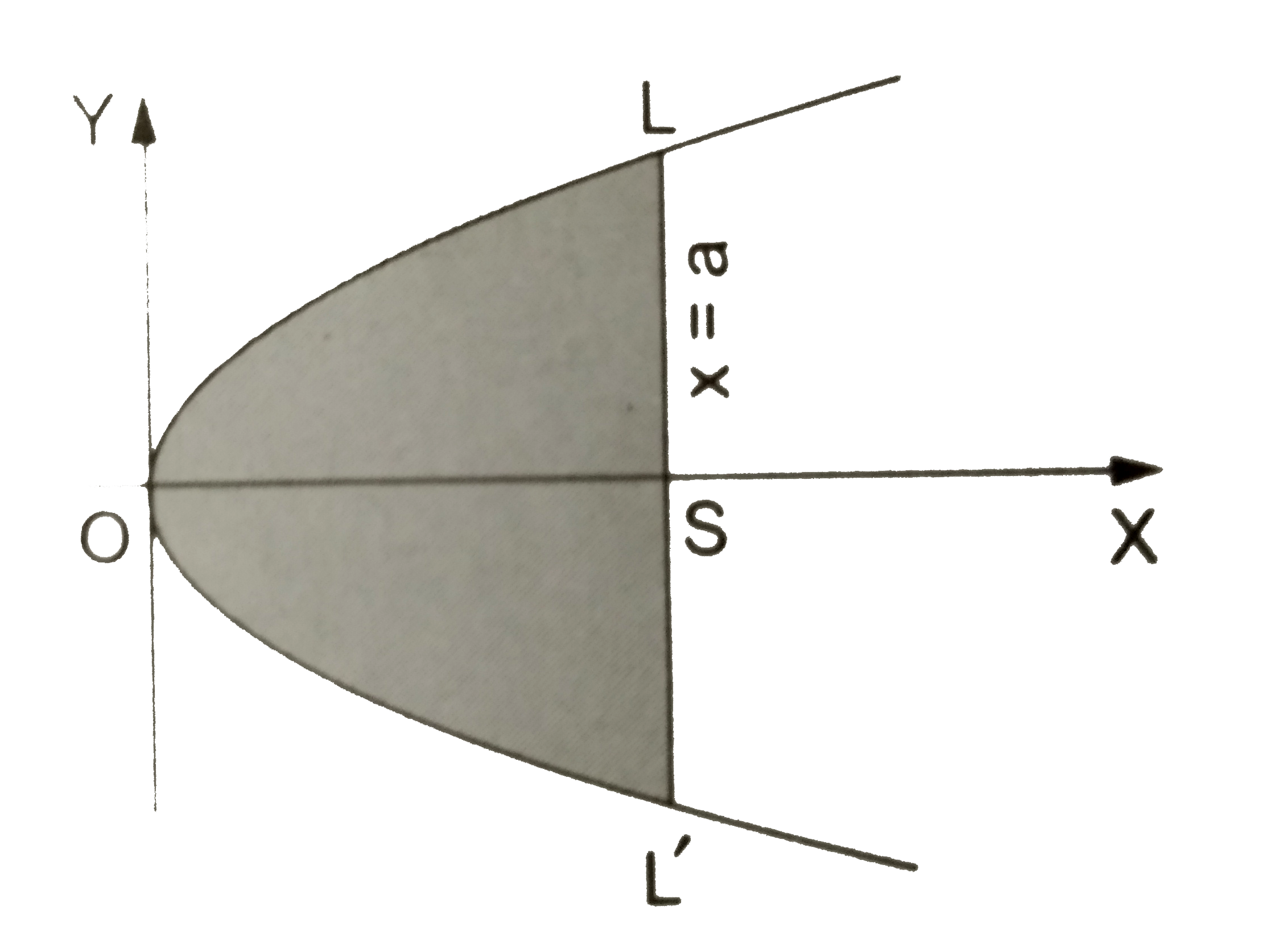
Calculate the area bouded by the parabola $y^{2} = 4 a x$ and its latus rectum

A

$\frac{5}{3} a^{2}$ sq units

B

$8 a^{2}$ sq units

C

$\frac{8}{3} a^{2}$ sq units

D

$\frac{8}{3} a^{2}$ sq units

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

Let s(a,0) be the foucus of the parabola $y^{2} = 4 a x$ The its latus rectum LSL is the line parallel to the y-axis at a distance a from it
So,its equation is x =a
Since the equation of the parabola contains only even powers of y, it is symmetrical about the x-axis.
$∴$ required area
= area LOL'L
= (area LOSL)+(area SOL's)
$= 2 \times (a r e a L O S L)$
$2 \underset{0}{\int^{a}} y d x = 2 . \underset{0}{\int^{a}} 2 \sqrt{a x} d x$
$= 4 \sqrt{a} \frac{.2}{3} {[x^{3 / 2}]}_{0}^{a} = \frac{8}{3} a^{2}$ sq units

|

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Calculate the area bouded by the parabola $y^{2} = 4 a x$ and its latus rectum
A $\frac{8}{3} a^{2}$
B $\frac{8}{3} a$
C $\frac{4}{3} a$
D $\frac{4}{3} a^{2}$
What is the area of the parabola $y^{2} = x$ bounde by its latus rectum ?
A $\frac{1}{12}$ square unit
B $\frac{1}{6}$ square unit
C $\frac{1}{3}$ square unit
DNone of the above

RS AGGARWAL-AREA OF BOUNDED REGIONS -Exercise 17

Home
Ask Doubt
Profile