Doubtnut Promotions Sticky

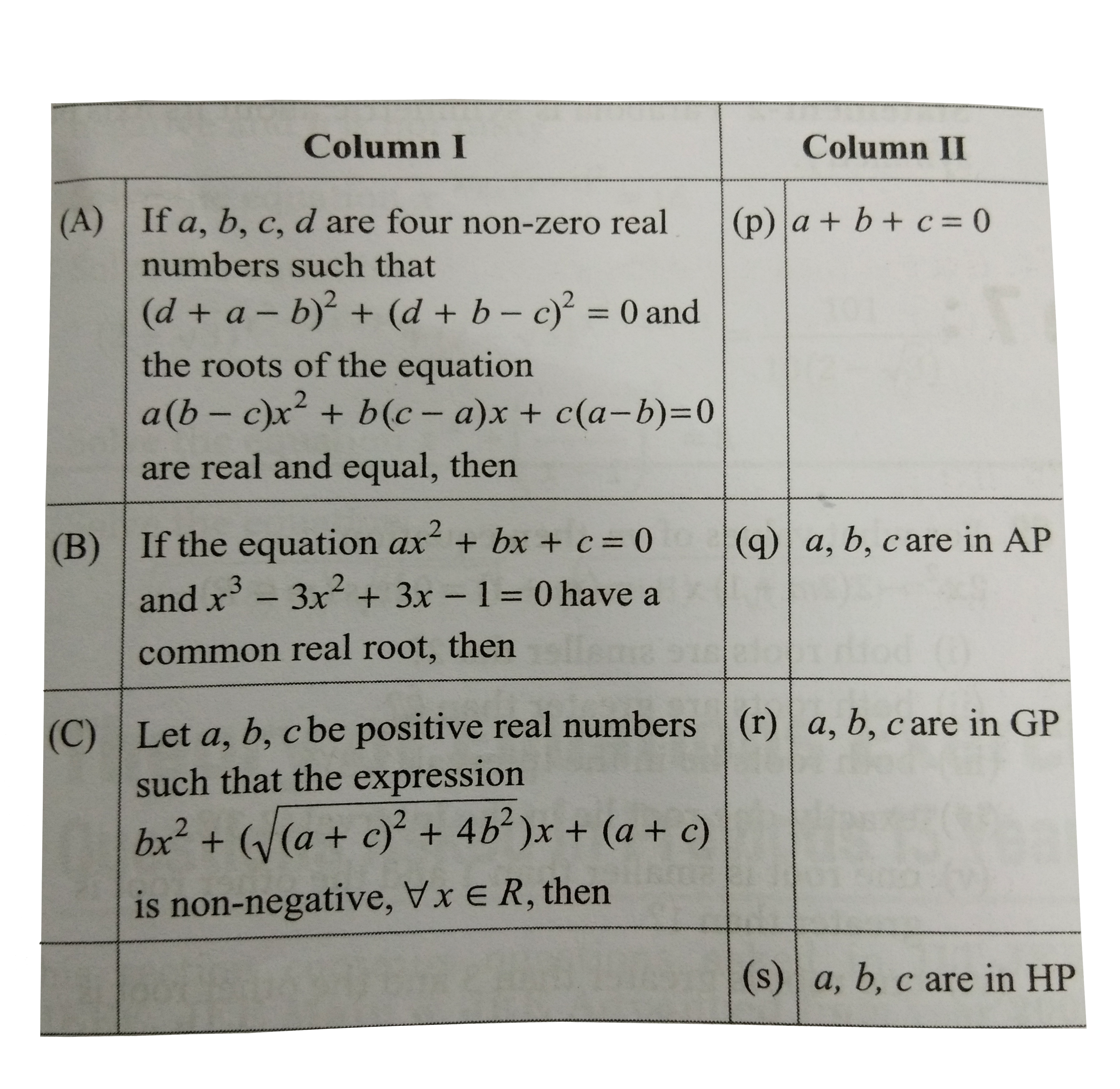
Column I contains rational algebraic expressions and Column II contains possible integers. Column I contains rational algebraic expressions and Column II contains possible integers.Solution in Punjabi

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:(A)→(p,q,r,s),(B)→(p,q),(C)t(s)

A→(p,q,r,s,),B→(p,q),C→(s)
(A) Let y=f(x)=x3−6x2+9x+λ
f'(x)=3x2−12x+9=0
∴x=1,3
f''(x)=6x−12
f''(1)<0 and f''(3)>0
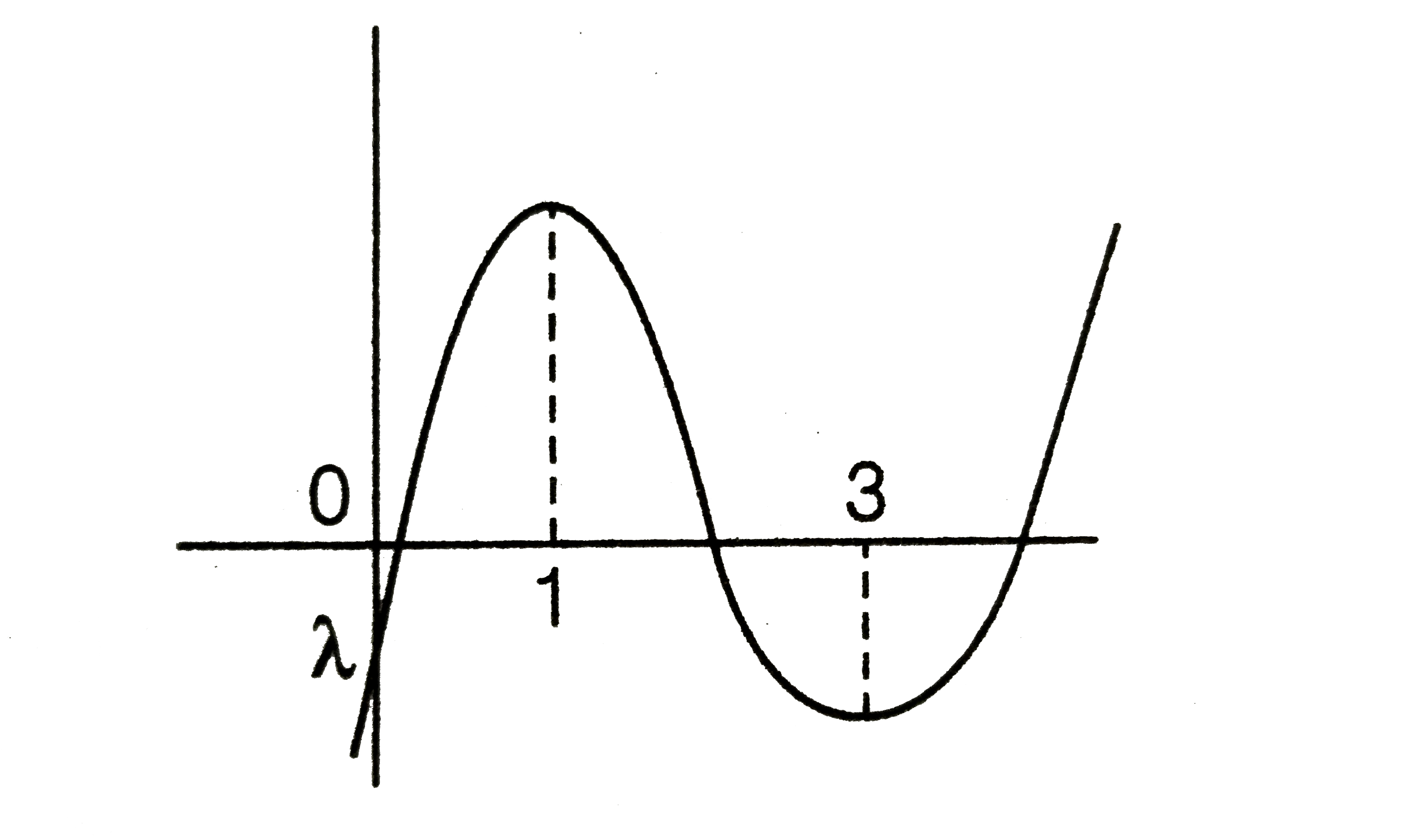
ocr_image
Also f(0)<0→λ<0
f(1)>0
⇒1−6+9+λ>0
⇒λ>−4......ii and f(3)<0 ltbr ⇒27−54+27+λ<0
⇒λ<0
From Eqs i and ii , iii we get
−4<ladma<0
⇒−3<λ+1<1
∴[λ+1]=−3,−2,−1,0
∴|[λ+1]|=3,2,1,0
(B) ∵x2+x+1>0,∀xεR
Given −3<x2−λx−2x2+x+1<2
⇒−3x2−3x−3<x2−λx−2<2x2+2x+2
⇒4x2−(λ+2)x+4>0
∴(λ−3)2−4.4.1<0
and (λ+2)2−4.1.4<0
⇒(λ−3)2−42<0
and (λ+2)2−42<0
⇒−4<λ−3<4
and −4<λ+2<4
or −1<λ<7
an −6<λ<2
We get −1<λ<2
∴[λ]=−1,0,1
⇒|[λ]|=0,1
(C)∵(b−c)+(c−a)+(a−b)=0
∴x=1 is a root of
(b−c)x2_(c−a)x+(a−b)=0
Also x=1 satisfies
x2+λx+1=0
⇒1+λ+1=0
∴λ=−2
Nowλ−1=−3
[λ−1]=−3
⇒|[λ−1]|=3

|
Updated on:21/07/2023
Class 12MATHSQUADRATIC EQUATIONS

Topper's Solved these Questions

Similar Questions