Consider a triangle with vertices and Find the equation of altitude through vertex the equation of median through vertex the equation of internal angle bisector of
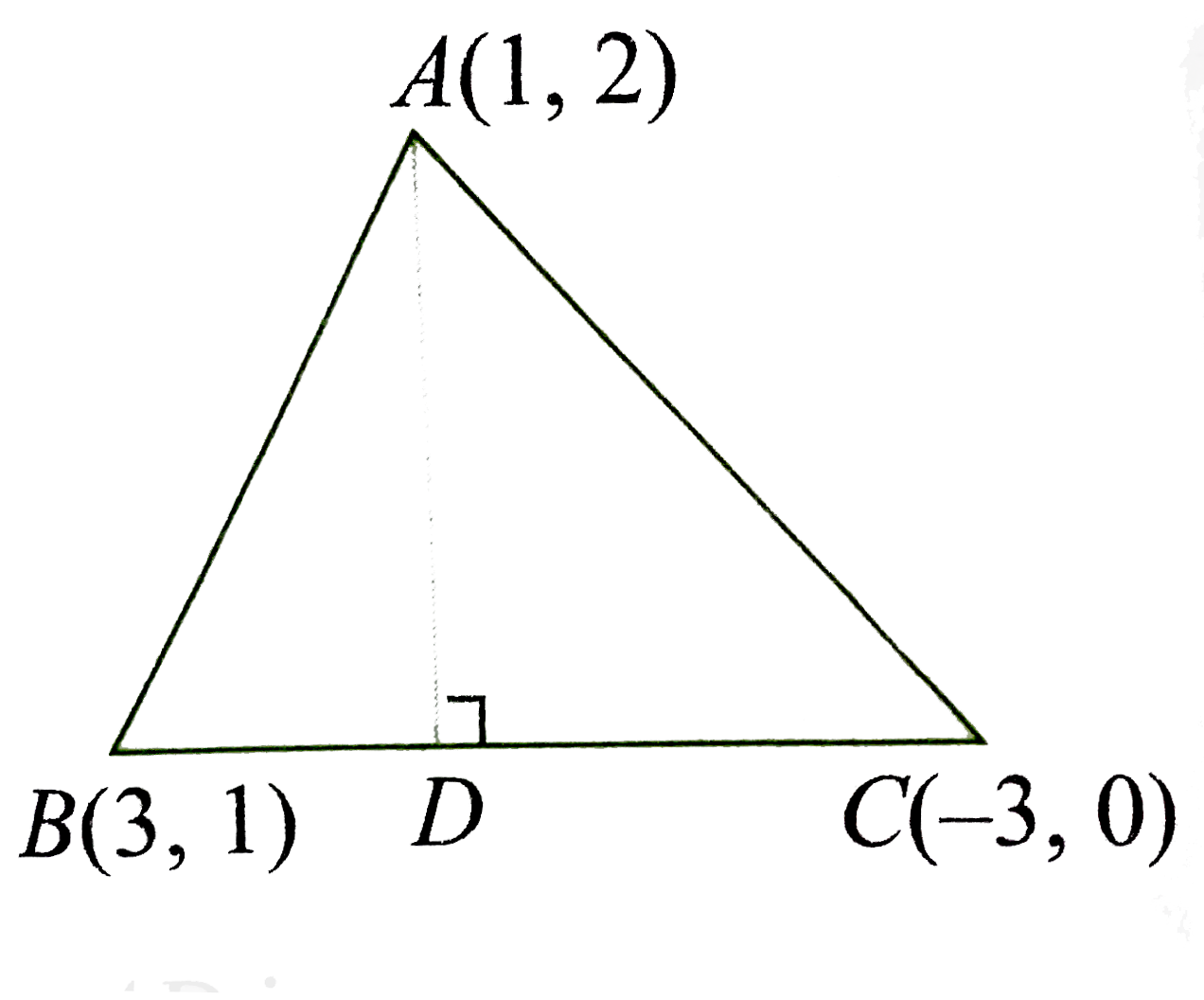
Let the altitude through A be AD.
Now, AD BC
Slope of BC
therefore, the equation of altitude AD is
y-2 = -6(x-1)
or 6x + y - 8 = 0

(ii) The median through A passes through the midpoint of BC.
Now, the midpoint of BC is E(3-3)/2, (1+0)/2) or E(0,1/2).
Therefore, the equation of
median AE is
or 3x-2y+1 = 0
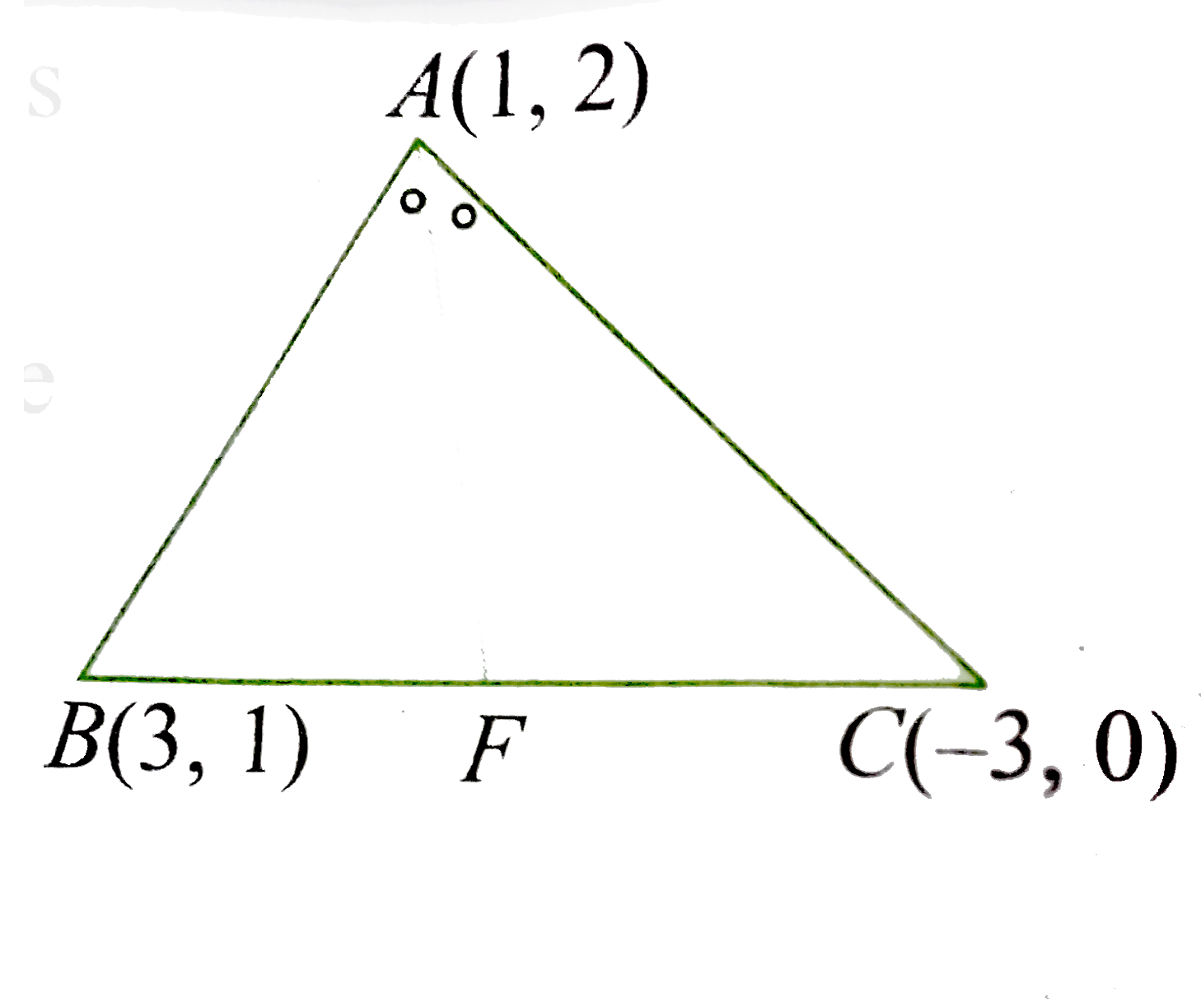
Let the internal bisector of A meets the side BC in F.
Now, the point F divides BC in the ratio
Therefore, the coordinates of F are
Therefore, the slope of AF is .
Hence, the equation of AF is x-1 = 0.
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.123videos
STRAIGHT LINES
- BOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.24videos
STRAIGHT LINES
- BOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type8videos
STRAIGHT LINE
- BOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - JEE Advanced Previous Year9videos
THEORY OF EQUATIONS
Similar Questions
Consider a triangle with vertices A(1,2),B(3,1), and C(−3,0). Find the equation of altitude through vertex A. the equation of median through vertex A. the equation of internal angle bisector of ∠A.
View SolutionConsider a triangle with vertices A(1;2) ;B(3;1) and C(-3;0) Find (i) equation of altitude through vertex A (ii) the equation of median through vertex A (iii) the equation of internal angle bisector of ∠A
View SolutionGiven the triangle with vertices A(−4,9),B(10,4),C(−2,−1). Find the equation of the altitude through A.
View SolutionIn the triangle ABC with vertices A (2, 3), B (4, –1) and C (1, 2), find the equation and length of altitude from the vertex A.
View SolutionThe vertices of a triangle are A(10,4),B(−4,9)andC(−2,−1).Find the equation of the altitude through A.
View SolutionConsider the ΔABC with vertices A(−2,3),B(2,1)andC(1,2). what is the foot of the altitude from the vertex A of the triangle ABC?
View SolutionIn a triangle ABC ,with vertices A(2,3),B(4,−1) and C(1,2) then foot of the altitude which is drawn from the vertex C is
View SolutionThe vertices of ΔABC are A(−3,2), B(0,3) and C(1,0). Find the equation of the median through B.
View SolutionThe vertices of a triangle are A (-1.3), B (1,-1) and C (5, 1). Find the length of the median through the vertex C.
View SolutionThe vertices of a triangle are (2,0) (0,2) (4,6) then the equation of the median through the vertex (2,0) is
View Solution

