Consider an object kept at the centre, in the XY plane, on which five coplanar force act as shown in the figure. The resultant force on the object is

6.5 N, 330∘
6.5 N, 300∘
6.5 N, 30∘
5.7 N, 331∘
The correct Answer is:A
Given that,
An object is at the centre of xy plane as shown in figure.

Five coplanar forces act on the object i.e. F1,F2,F3,F4,andF5
The equation for force acting along direction is given by F=Fx+jFy
=Fcosθ+jFsinθ
Force, F1 acting positive X - axis as 0∘ is given by,
F1=Fcosθ+jFsinθ (∵ From equation (1))
=19cosθ+j10sinθ
=19(1)+j19(θ)
⇒F1=19
Force F2 acting along 15. N and angle 60∘ is given by
F2=15cos60+15sin60 (∵ From equation (1))
=7.5+j12.99
Force F3 acting along 16 N and angle 180∘−45∘=135∘ is
given by,
F3=16cos135∘+16sin135∘ (∵From equation(1))
=−11.3+j11.3
Force F4 acting along 11 N and angle 180∘+30∘=210∘ is given by,
F4=11cos210∘+11sin210∘(∵ From equation(1))
=−9.53−j5.5
Force F5 acting along 22.0 N and angle 270∘ is given by,
F5=22cos270∘+22sin270∘ (∵ From equation(1))
=0−j22
=−j22.
The total force acting on the object is given by
FT=F1+F2+F3+F4+F5
Substituting corresponding values in the above equation,
⇒FT=(19)+(7.5+j12.99)+(−11.3+j11.3)+(−9.53−j5.5)+(−j22)
=(19+7.5−11.3−9.53)+j(12.99+11.3−5.5−22)
=5.67−3.21j
The resultant force acting on the object is given by,
FR=|FT|FT
=|5.67−3.21j|(5.67−3.21j)
=[√(5.67)2+(−3.21)2][tan−1(−3.215.67)]
=√42.453(360−tan−1(3.215.67))
=6.536∘−29.5
=6.5330.5
∴FR=6.5N,330∘
Topper's Solved these Questions
- EAMCET - 2018 (TS) SHIFT - 2BOOK - SIA PUBLICATIONCHAPTER - EAMCET - 2018 (TS) SHIFT - 2EXERCISE - Chemistry
18 Videos
- EAMCET - 2018 (TS) SHIFT - 2BOOK - SIA PUBLICATIONCHAPTER - EAMCET - 2018 (TS) SHIFT - 2EXERCISE - Chemistry
18 Videos
- EAMCET - 2018 (TS) SHIFT - 1BOOK - SIA PUBLICATIONCHAPTER - EAMCET - 2018 (TS) SHIFT - 1EXERCISE - EXERCISE - Chemistry
8 Videos
- EAMCET -2016 (AP)BOOK - SIA PUBLICATIONCHAPTER - EAMCET -2016 (AP)EXERCISE - CHEMISTRY
3 Videos
Similar Questions
Knowledge Check
Two forces, each equal to F, act as shown in figure. Their resultant is
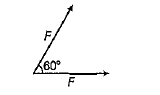 AF/2BFC√3D√5F
AF/2BFC√3D√5FTwo forces, each equal to F, act as shown in (figure) Their resultant is
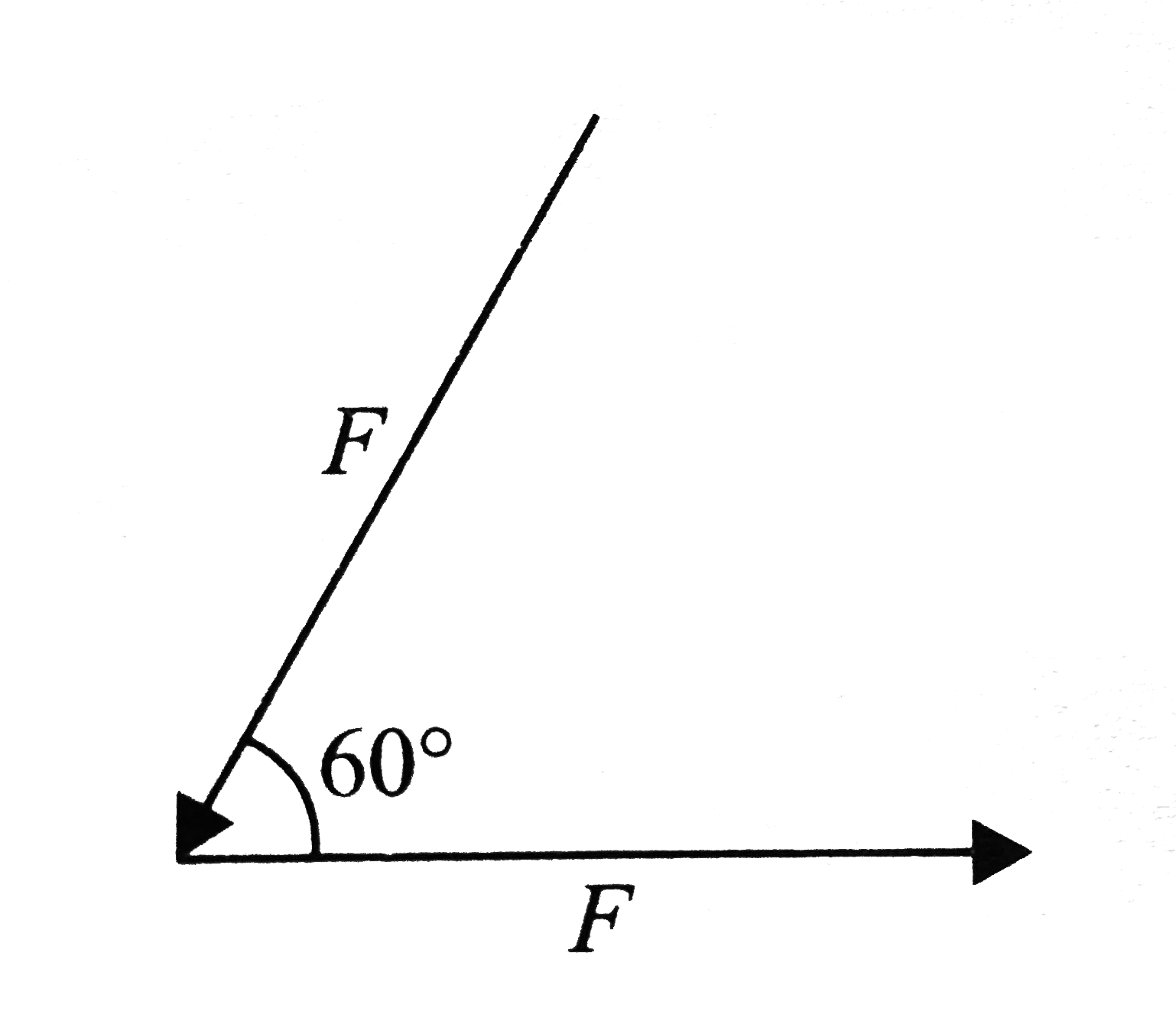 AF/2BFC√3FD√5F
AF/2BFC√3FD√5FIn the above question, the force acting on the object is
A30 NB−30NC3 ND−3N
The force acting on an object varies with the distance travelled by the object as shown in the figure. Find the work done by the force in moving the object from x=0m to x=14m.
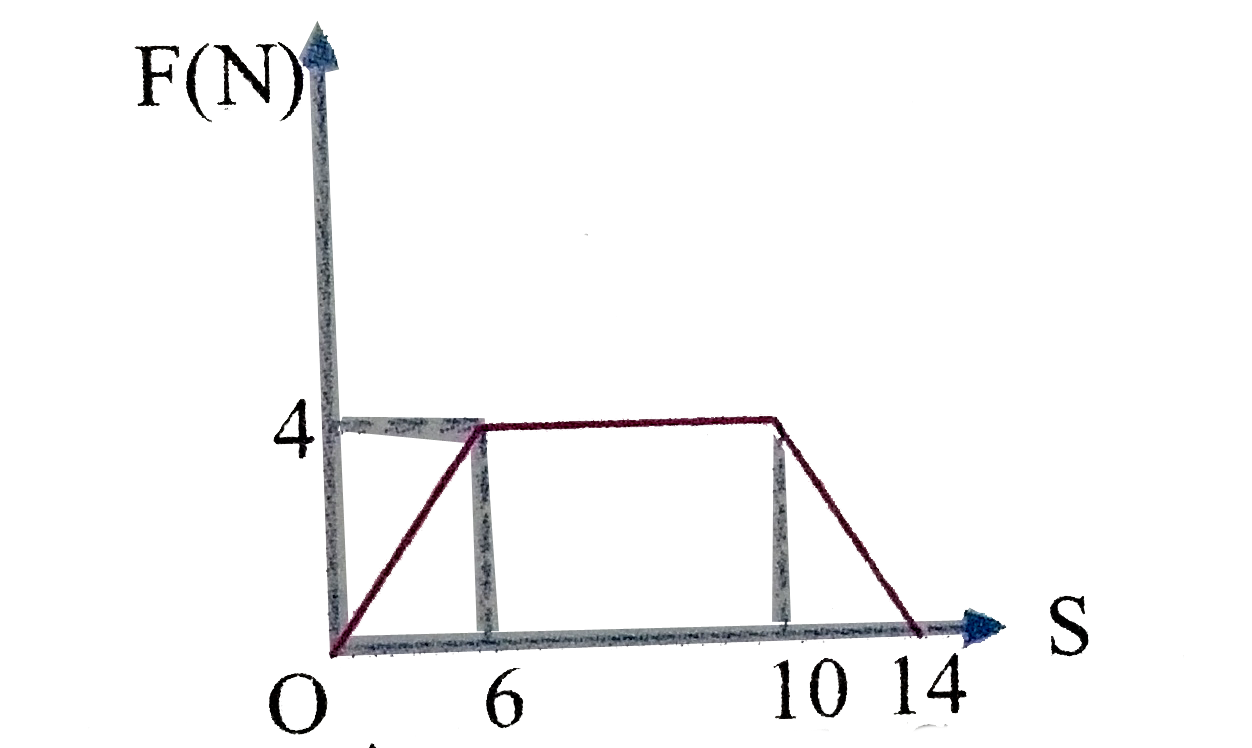 .
.
View SolutionIn the given figure O is the centre of regular pentagon ABCDE. Five forces each of magnitude F0 are acted as shown in figure. The resultant force is

View SolutionIn the given figure O is the centre of regular pentagon ABCDE. Five forces each of magnitude F0 are acted as shown in figure. The resultant force is

View SolutionA Force F acting on an object varies with distance x as shown in the figure.
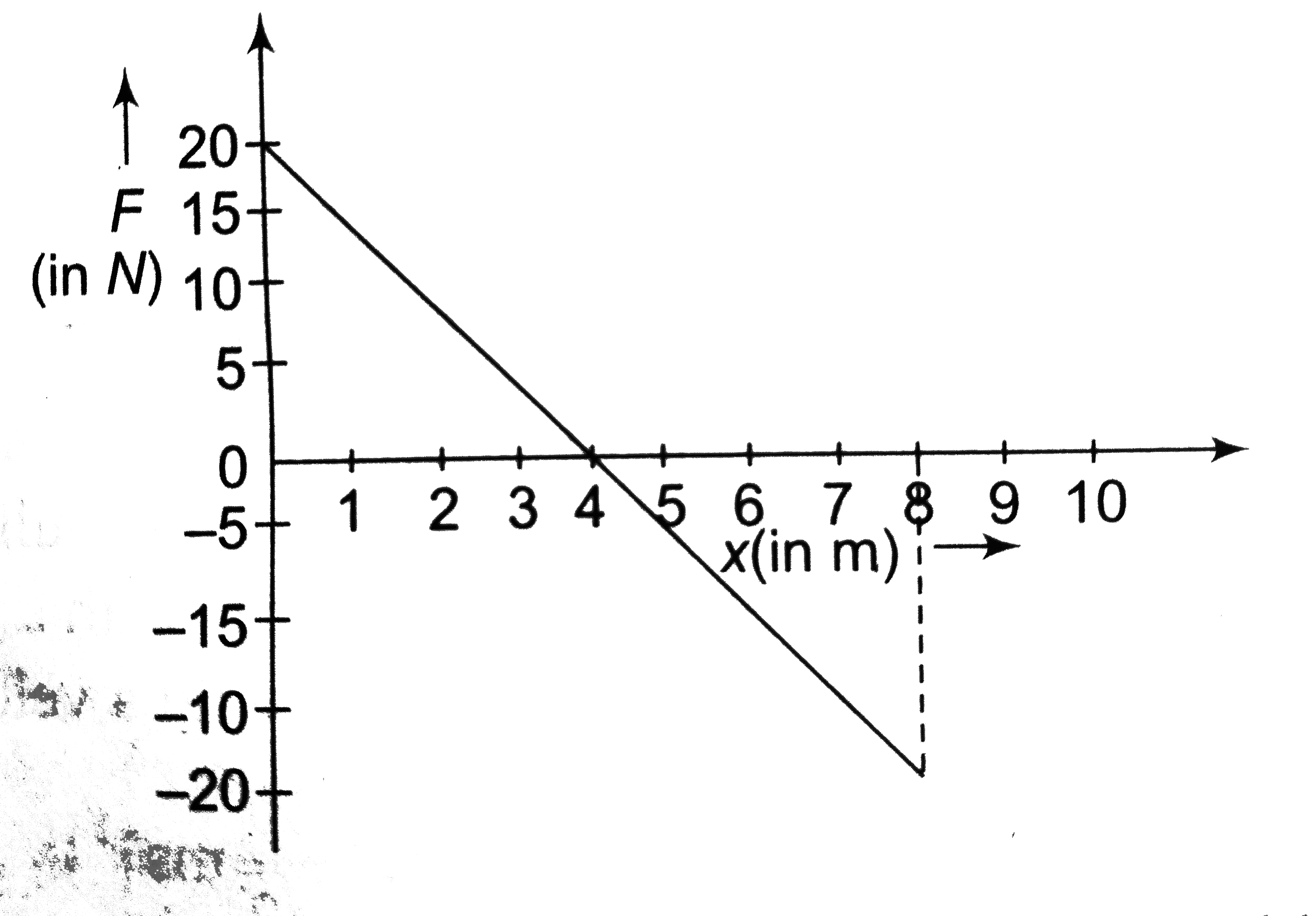
The work done by the force in moving the object from x=0 to x=8m is
View SolutionA force F acting on an object varies with distance x as shown in the figure. The work done by the force in moving the object from x=0 to x = 20 m is
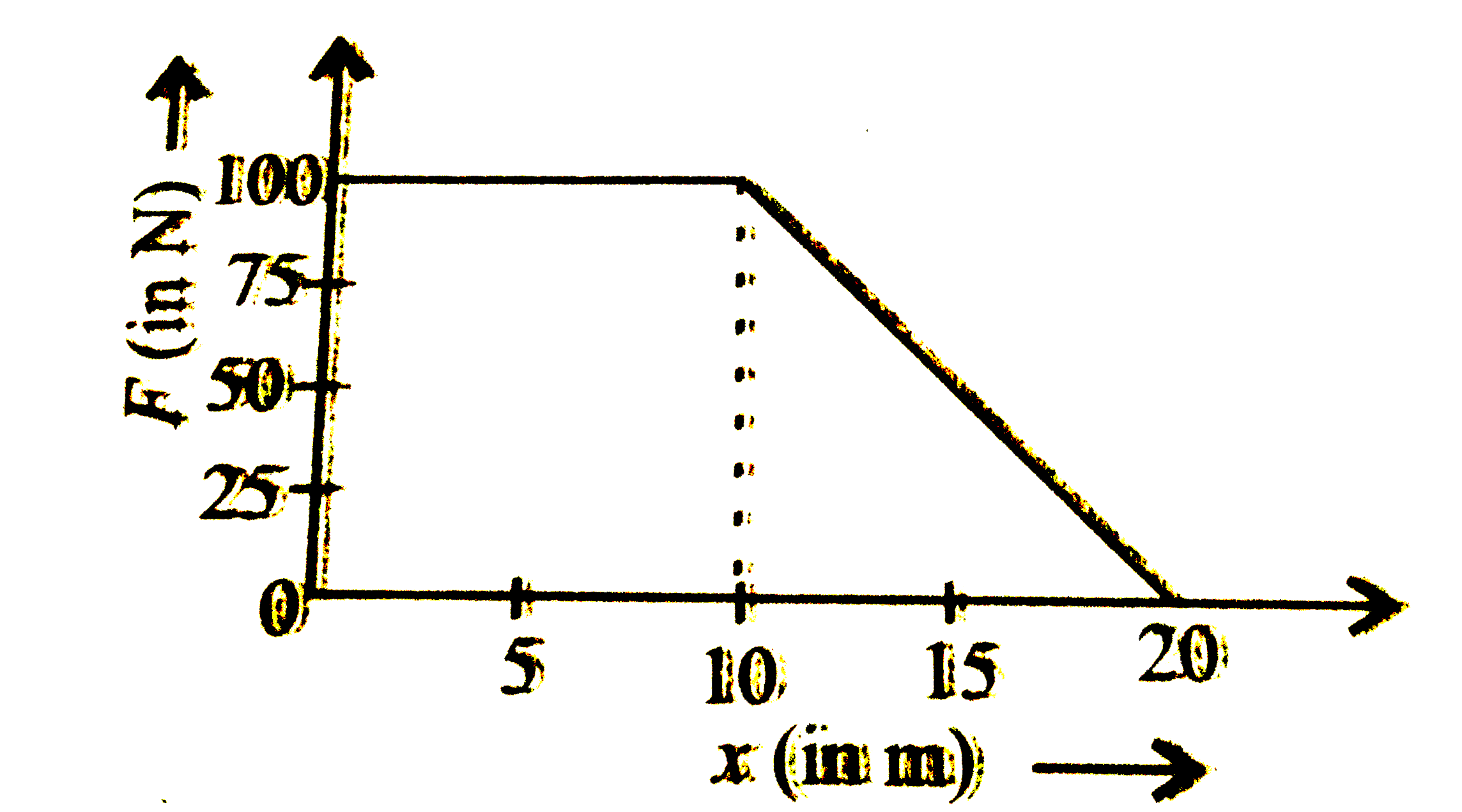
View Solution
