consider determinant Δ=∣∣aij∣∣ of order 3. If Δ=2 the match the following lists.

The correct Answer is:2
Let Δ=∣∣
∣
∣∣abcpqrxyz∣∣
∣
∣∣
(a)Δ1=(−1)i+jaij=∣∣
∣
∣∣a−bc−pq−rx−yz∣∣
∣
∣∣
=∣∣
∣
∣∣−a−b−cpqr−x−y−z∣∣
∣
∣∣ Multiplying C1 and C3 by (−1)
=∣∣
∣
∣∣abcpqrxyz∣∣
∣
∣∣=Δ=2
(b)Δ2+∣∣2i+jaij∣∣=∣∣
∣
∣∣22a23b24c23p24q25r24x25y26z∣∣
∣
∣∣
=22×23×24∣∣
∣
∣∣a2b22cp2q22rx2y22z∣∣
∣
∣∣
=29×2×22∣∣
∣
∣∣abcpqrxyz∣∣
∣
∣∣
=212×2=213
(C)Δ3=∣∣Cij∣∣=Δ2=4
(d)Δ4=(Δ3)2=42=24
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - DETERMINANTEXERCISE - Multiple Correct Answer5videos
DETERMINANT
- BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Multiple correct answers type11videos
DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS
Similar Questions
Knowledge Check
- Question 1 - Select One or More
If D is a determinant of order three and Δ is a determinant formed by the cofactors of determinant D then
AΔ=D2BD=0 implies Δ=0Cif D=27, then Δ is perfect cubeDNone of these - Question 2 - Select One or More
If D is determinant of order three of Δ is a determinant formed by the cofactors of determinant D: then
AΔ=D2BD=0impliesΔ=0CIf D=27 then Δ is perfect cubeDIf D=27, then Δ is a perfect square - Question 3 - Select One
Consider a matrix A=[aij] of order 3×3 such that aij=(k)1+j where k∈I
Match Column I with Column II.
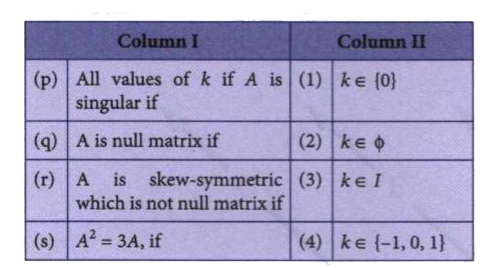
Now, choose the correct option from amongst the given codes :A(p)(q)(r)(s)(3)(1)(4)(2)B(p)(q)(r)(s)(4)(1)(2)(3)C(p)(q)(r)(s)(3)(1)(2)(4)D(p)(q)(r)(s)(2)(1)(3)(4)
Consider the determinants Δ=∣∣ ∣∣2−131111−11∣∣ ∣∣,Δ'=∣∣ ∣∣20−2−2−11−413∣∣ ∣∣
STATEMENT-1 Δ'=Δ2.
STATEMENT-2 : The determinant formed by the cofactors of the elements of a determinant of order 3 is equal in value to the square of the original determinant.View SolutionConsider the determinants Δ=∣∣ ∣∣2−131111−11∣∣ ∣∣,Δ'=∣∣ ∣∣20−2−2−11−413∣∣ ∣∣
STATEMENT-1 Δ'=Δ2.
STATEMENT-2 : The determinant formed by the cofactors of the elements of a determinant of order 3 is equal in value to the square of the original determinant.View SolutionConsider a matrix A=[aij] of order 3×3 such that aij=(k)i+j where k∈I.
Match List I with List II and select the correct answer using the codes given below the lists.
 View Solution
View SolutionConsider the lines represented by equation (x2+xy−x)×(x−y)=0 forming a triangle. Then match the following lists:
 View Solution
View SolutionConsider a matrix A=[aij] of order 3×3 such that aij=(k)i+j where k∈I.
Match List I with List II and select the correct answer using the codes given below the lists.
 View Solution
View Solution


