Consider the functions f1(x)=x,f2(x)=2+logex,x>0. The graphs of the functions intersectSolution in Bengali
once in (0, 1) but never in (1,∞)
once in (0, 1) and once in (e2,∞)
once in (0, 1) and once in (e,e2)
more than twice in (0,∞)
Step by step video & image solution for Consider the functions f_(1)(x)=x,f_(2)(x)=2+log_(e )x, x gt 0. The graphs of the functions intersect by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Let A and B be two square matrices of order 3 and AB=O3, where O3 denotes the null matrix of order 3. Then
View SolutionLet f(x)=ax(a>0) be written as f(x)=f1(x)+f2(x), where f1(x) is an function and f2(x) is an odd function. Then f1(x+y)+f1(x−y) equals
View SolutionFor x∈R−{0,1}, let f1(x)=1x,f2(x)=1−xandf3(x)=11−x be three given functions. If a function, J(x) satisfies (f2∘J∘f1)(x)=f3(x), then J(x) is equal to
View SolutionLet f(1):R→R,f2:[0,∞)→R,f3:R→Randf4:R→[0,∞) be a defined by
f1(x)={|x|if x<0exif x>0:f2(x)=x2,f3(x)={sinxif x<0xif x≥0 and f4(x)={f2(f1(x))if x<0f2(f1(f1(x)))−1if x≥0 Then, f4 is
View SolutionLet f1(x)andf2(x) be twice differentiable functions where F(x)=f1(x)+f2(x)andG(x)=f1(x)−f2(x),∀x∈R,f1(0)=2andf2(0)=1.Iff'1(x)=f2(x)andf'2(x)=f1(x),∀x∈R. then the number of solutions of the equation (F(x))2=9x4G(x)is...... .
View SolutionLet f1:R→R,f2:[0,∞)→R,f3:R→R be three function defined as
f1(x)={|x|x<0exx≥0,f2(x)=x2,f3(x)={f2(f1(x))x<0f2(f1(x))−1x≥0 then f3(x) is:
View Solutionमाना f(x)=ax(a>0) को (f(x)=f1+f2(x) के रूप मे लिखा गया है जबकि f1(x) एक सम फलन है। और f2(x) का विषम फलन है। तो f1(x+y)+f1(y−x) बराबर है।
View Solutionx∈R−{0,1} के लिए, तीन फलन f1(x)=1x,f2(x)=1−x तथा f3(x)=11−x दिए गए हैं। यदि एक फलन J(x),(f2⋅J⋅f1)(x)=f3(x) को सन्तुष्ट करता है, तो J (x) बराबर है
View Solutionमाना f(x)=ax(a>0) को (f(x)=f1+f2(x) के रूप मे लिखा गया है जबकि f1(x) एक सम फलन है। और f2(x) का विषम फलन है। तो f1(x+y)+f1(y−x) बराबर है।
View Solutionयदि fn(x)=efn−1(x)∀n∈N तथा f0(x)=x तो प्रदर्शित कीजिए कि ddx{fn(x)}=f1(x).f2(x)........fn(x) है।
View Solutionx in R-{0,1} For, three functions f_(1)(x)=(1)/(x),f_(2)(x)=1-x And f_(e)(x)=(1)/(1-x) Are given. If a function J(x),(f_(2)*J*f_(1))(x)=f_(3)(x) Satisfies, then J (x) is equal to
View Solutionफलन f1(x)=⎧⎪⎨⎪⎩1यदिx≤0x2+1यदि0<x<25यदिx≥2,f2(x)=log1/2(x−3),f3(x)=23−x तथा f4(x)=e{x} जहाँ {.} भिन्नात्मक भागफलन को प्रदर्शित करता है, के आरेख निम्न (क्रम में नहीं) है-
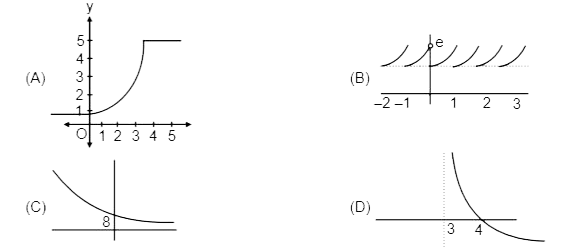
फलन f1(x),f2(x),f3(x) एवं f4(x) के आरेखों का सही क्रम होगा-
View SolutionLet f1(x)andf2(x) be twice differentiable functions where F(x)=f1(x)+f2(x)andG(x)=f1(x)−f2(x),∀x∈R,f1(0)=2andf2(0)=1.Iff'1(x)=f2(x)andf'2(x)=f1(x),∀x∈R then the number of solutions of the equation (F(x))2=9x4G(x)is...... .
View SolutionLet f1:R→R,f2:[0,∞)→R,f3:R→R be three function defined as
f1(x)={|x|x<0exx≥0,f2(x)=x2,f3(x)={f2(f1(x))x<0f2(f1(x))−1x≥0 then f3(x) is:
View SolutionLet f(1):R→R,f2:[0,∞)→R,f3:R→Randf4:R→[0,∞) be a defined by
f1(x)={|x|if x<0exif x>0:f2(x)=x2,f3(x)={sinxif x<0xif x≥0 and f4(x)={f2(f1(x))if x<0f2(f1(f1(x)))−1if x≥0 Then, f4 is
View SolutionLet f1:R→R,f2:[0,∞)→R,f3:R→Randf4:R→[0,∞) be a defined by
f1(x)={|x|if x<0exif x>0:f2(x)=x2,f3(x)={sinxif x<0xif x≥0 and f4(x)={f2(f1(x))if x<0f2(f1(f1(x)))−1if x≥0 then f2 of f1 is
View Solution
