D is the mid-point of side BC of ΔABC and E is the mid-point of BO. If O is the mid-point of AE, then ar(ΔBOE) = 1/k ar(ΔABC). Then k equals
The correct Answer is:8
To solve the problem, we need to determine the value of k such that the area of triangle BOE is equal to 1k times the area of triangle ABC.
1. Identify Midpoints:
- Let D be the midpoint of side BC in triangle ABC.
- Let E be the midpoint of segment BD.
- Let O be the midpoint of segment AE.
2. Area of Triangle ABD:
- Since D is the midpoint of BC, the median AD divides triangle ABC into two equal areas.
- Therefore, the area of triangle ABD is:
Area of △ABD=12Area of △ABC
3. Area of Triangle ABE:
- Since E is the midpoint of BD, the median AE divides triangle ABD into two equal areas.
- Therefore, the area of triangle ABE is:
Area of △ABE=12Area of △ABD=12(12Area of △ABC)=14Area of △ABC
4. Area of Triangle BOE:
- Since O is the midpoint of AE, the median BO divides triangle ABE into two equal areas.
- Therefore, the area of triangle BOE is:
Area of △BOE=12Area of △ABE=12(14Area of △ABC)=18Area of △ABC
5. Relate Areas:
- We know from the problem statement that:
Area of △BOE=1kArea of △ABC
- From our calculation, we found that:
Area of △BOE=18Area of △ABC
- Therefore, we can equate:
1kArea of △ABC=18Area of △ABC
6. Solve for k:
- By comparing both sides, we find:
1k=18⟹k=8
Final Answer:
Thus, the value of k is 8.
---
- Let D be the midpoint of side BC in triangle ABC.
- Let E be the midpoint of segment BD.
- Let O be the midpoint of segment AE.
- Since D is the midpoint of BC, the median AD divides triangle ABC into two equal areas.
- Therefore, the area of triangle ABD is:
Area of △ABD=12Area of △ABC
- Since E is the midpoint of BD, the median AE divides triangle ABD into two equal areas.
- Therefore, the area of triangle ABE is:
Area of △ABE=12Area of △ABD=12(12Area of △ABC)=14Area of △ABC
- Since O is the midpoint of AE, the median BO divides triangle ABE into two equal areas.
- Therefore, the area of triangle BOE is:
Area of △BOE=12Area of △ABE=12(14Area of △ABC)=18Area of △ABC
- We know from the problem statement that:
Area of △BOE=1kArea of △ABC
- From our calculation, we found that:
Area of △BOE=18Area of △ABC
- Therefore, we can equate:
1kArea of △ABC=18Area of △ABC
- By comparing both sides, we find:
1k=18⟹k=8
Thus, the value of k is 8.
Topper's Solved these Questions
- AREAS OF PARALLELOGRAMS AND TRIANGLESBOOK - MTG IIT JEE FOUNDATIONCHAPTER - AREAS OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Olympiad/HOTS Corner
14 Videos
- AREAS OF PARALLELOGRAMS AND TRIANGLESBOOK - MTG IIT JEE FOUNDATIONCHAPTER - AREAS OF PARALLELOGRAMS AND TRIANGLESEXERCISE - EXERCISE ( Subjective Problems ) (Long Answer Type )
3 Videos
- CIRCLES BOOK - MTG IIT JEE FOUNDATIONCHAPTER - CIRCLES EXERCISE - OLYMPIAD/HOTS CORNER
6 Videos
Similar Questions
D is the mid-point of side AB of the triangle ABC.E is the mid-point of CD and F is the mid-point of AE. Prove that 8× area of (ΔAFD)= area of ΔABC
View SolutionIn the given figure, D is the mid-point of BC, E is the mid-point of BD and O is the mid-point of AE. Find the ratio of area of ΔBOEandΔABC

View Solution
Knowledge Check
ABC and BDE are two equilateral triangles such that D is the mid-point of BC. Then, ar(ΔBDE)=12ar(ΔABC).
ATrueBFalseCPartially trueDNone of theseIf D id the mid-point of the side BC of a triangle ABC and AD is perpendicular to AC , then
Ab2=a2−c2Ba2+b2=5c2C3b2=a2−c2D3a2=b2−3c2If D id the mid-point of the side BC of a triangle ABC and AD is perpendicular to AC , then
Aa2+b2+c2=4b2Ba2+b2−c2=4b2Ca2−b2+c2=4b2Da2−b2−c2=4b2
P and Q are respectively the mid-points of sides AB and BC of a triangle ABC and R is the mid-point of AP, show that
ar(ΔPRQ) = 1/2 ar(ΔARC)
View SolutionIn the adjoining figure, D is the mid-point of side AB of ΔABC and P be any point on side BC. If CQ∣∣PD, then prove that:
area of ΔBPQ=12× area of ΔABC
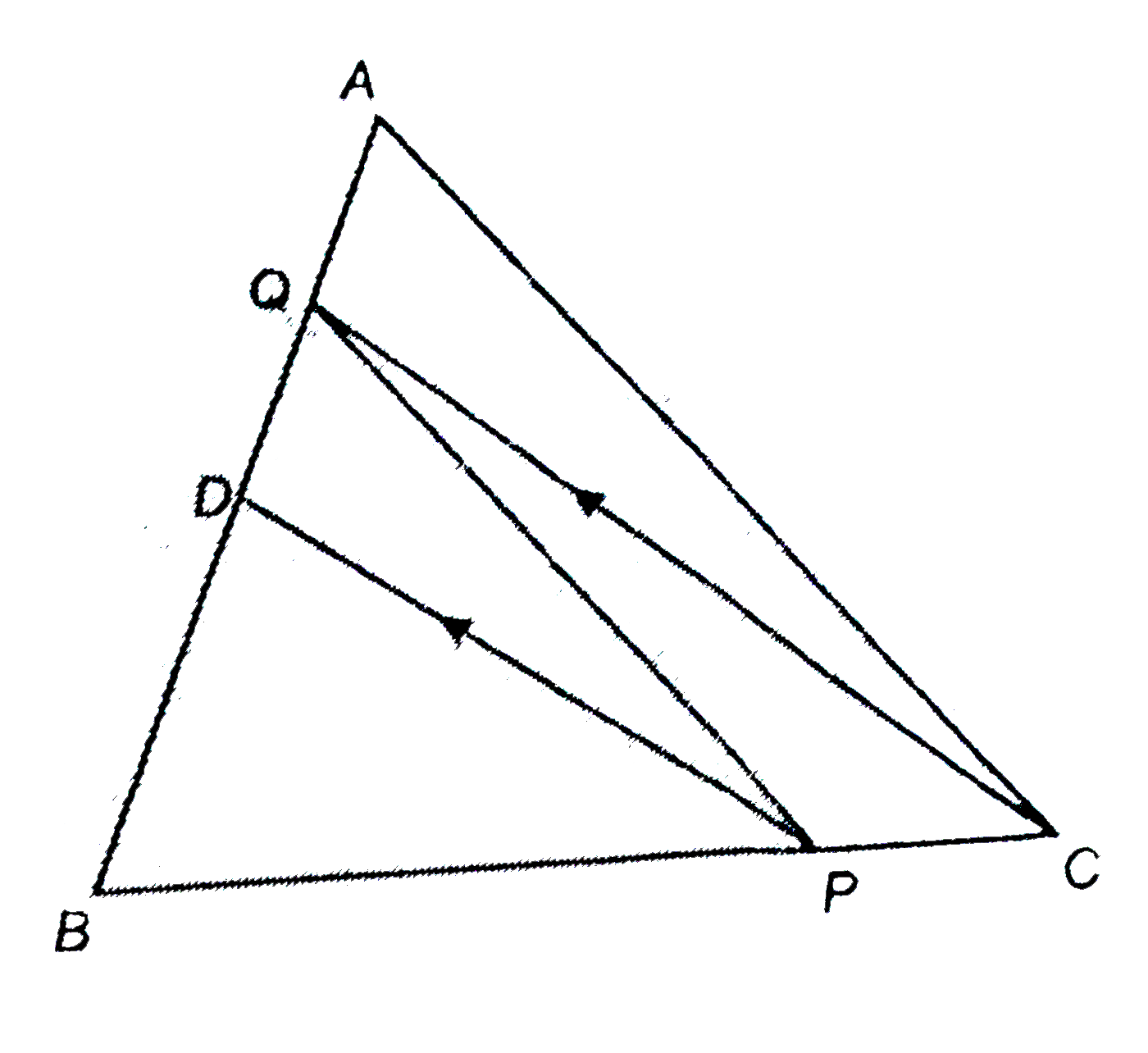
View SolutionIn a ΔABC, if D is the mid point of BC and AD is perpendicular to AC, then cosB=
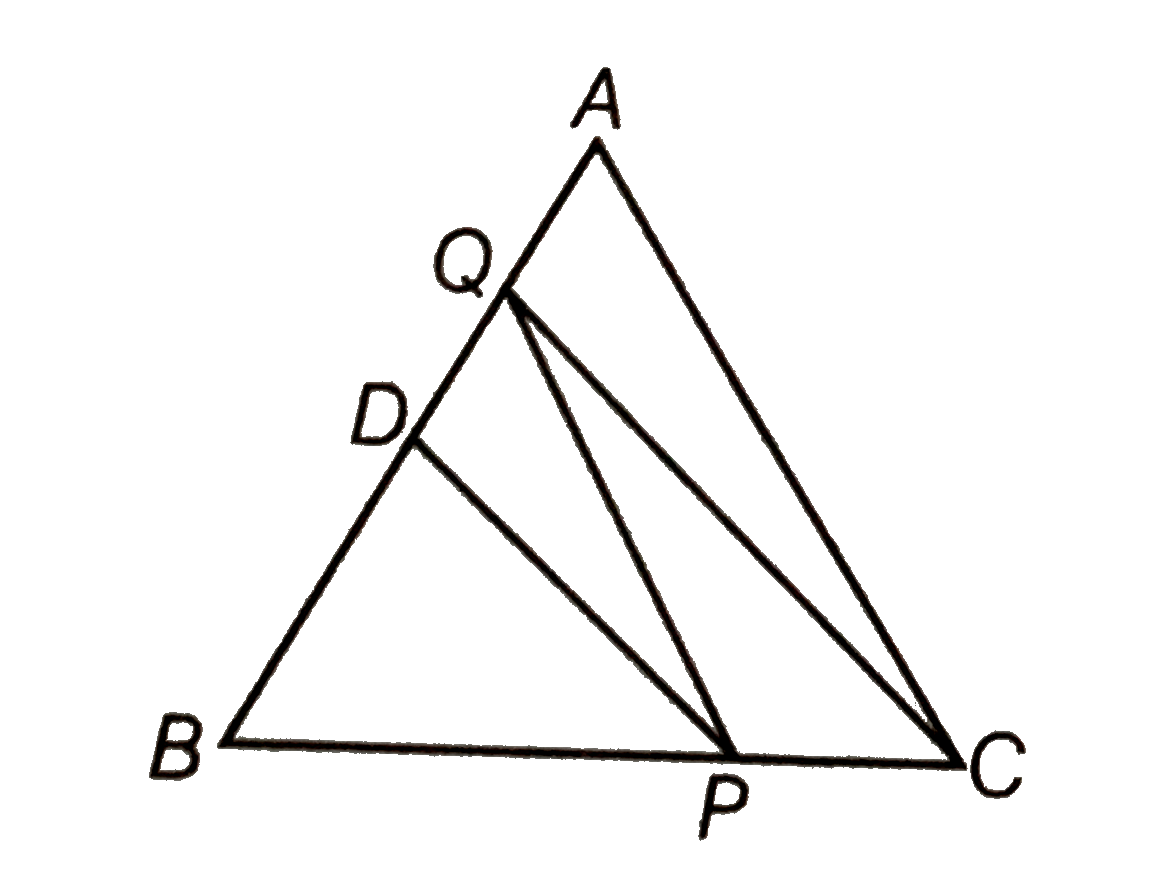
View SolutionIn Δ ABC, D is the mid-point of AB and P is any point on BC. If CQ∣∣PD meets AB and Q (shown in figure), then prove that
ar(ΔBPQ)=12ar(ΔABC).
View SolutionIn ΔABC, P is a point on BC such that BP:PC=4:5 and Q is the mid - point of BP. Then area (ΔABQ) : area (ΔABC) is equal to :
View Solution