ΔI1I2I3 is an excentral triangle of an equilateral triangle ΔABC such that I1I2=4 unit, if ΔDEF is pedal triangle of ΔABC, then Ar(ΔI1I2I3)Ar(ΔDEF)=
16
4
2
1
The correct Answer is:A
Step by step video, text & image solution for Delta I_(1)I_(2)I_(3) is an excentral triangle of an equilateral triangle Delta ABC such that I_(1)I_(2)=4 unit, if DeltaDEF is pedal triangle of DeltaABC, then (Ar(Delta I_(1)I_(2)I_(3)))/(Ar (DeltaDEF))= by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SOLUTION OF TRIANGLESBOOK - VK JAISWALCHAPTER - SOLUTION OF TRIANGLESEXERCISE - Exercise-2 : One or More than One Answer is/are Correct
15 Videos
- SOLUTION OF TRIANGLESBOOK - VK JAISWALCHAPTER - SOLUTION OF TRIANGLESEXERCISE - Exercise-3 : Comprehension Type Problems
16 Videos
- SEQUENCE AND SERIES BOOK - VK JAISWALCHAPTER - SEQUENCE AND SERIES EXERCISE - EXERCISE (SUBJECTIVE TYPE PROBLEMS)
21 Videos
- STRAIGHT LINES BOOK - VK JAISWALCHAPTER - STRAIGHT LINES EXERCISE - Exercise-5 : Subjective Type Problems
10 Videos
Similar Questions
Knowledge Check
If z1,z2,z3 are the vertices of an equilational triangle ABC such that |z1−i|=|z2−i|=|z3−i|, then |z1+z2+z3| equals to
A3√3B√3C3D13√3
If I1,I2,I3 are the centers of escribed circles of ΔABC, show that the area of ΔI1I2I3 is (abc)/(2r)
View SolutionΔABC and ΔDEF are equilateral triangles. If A(ΔABC):A(ΔDEF)=1:2 and AB=4, find DE.
View SolutionABC is an isosceles right Angle triangle . I is incentre. Find ratio of area of triangle ΔAICandΔABC
View SolutionIf origin and 2−i are two vertices of an equilateral triangle, then determine the third vertex.
View SolutionIf I1,I2,I3 are excentres of the triangle with vertices (0,0),(5,12),(16,12) then the orthocentre of ΔI1I2I3 is
View SolutionIf is the incentre of ΔABCandI1, is the excentre opposite to the vertex. 'A' of ΔABC, thern II1=
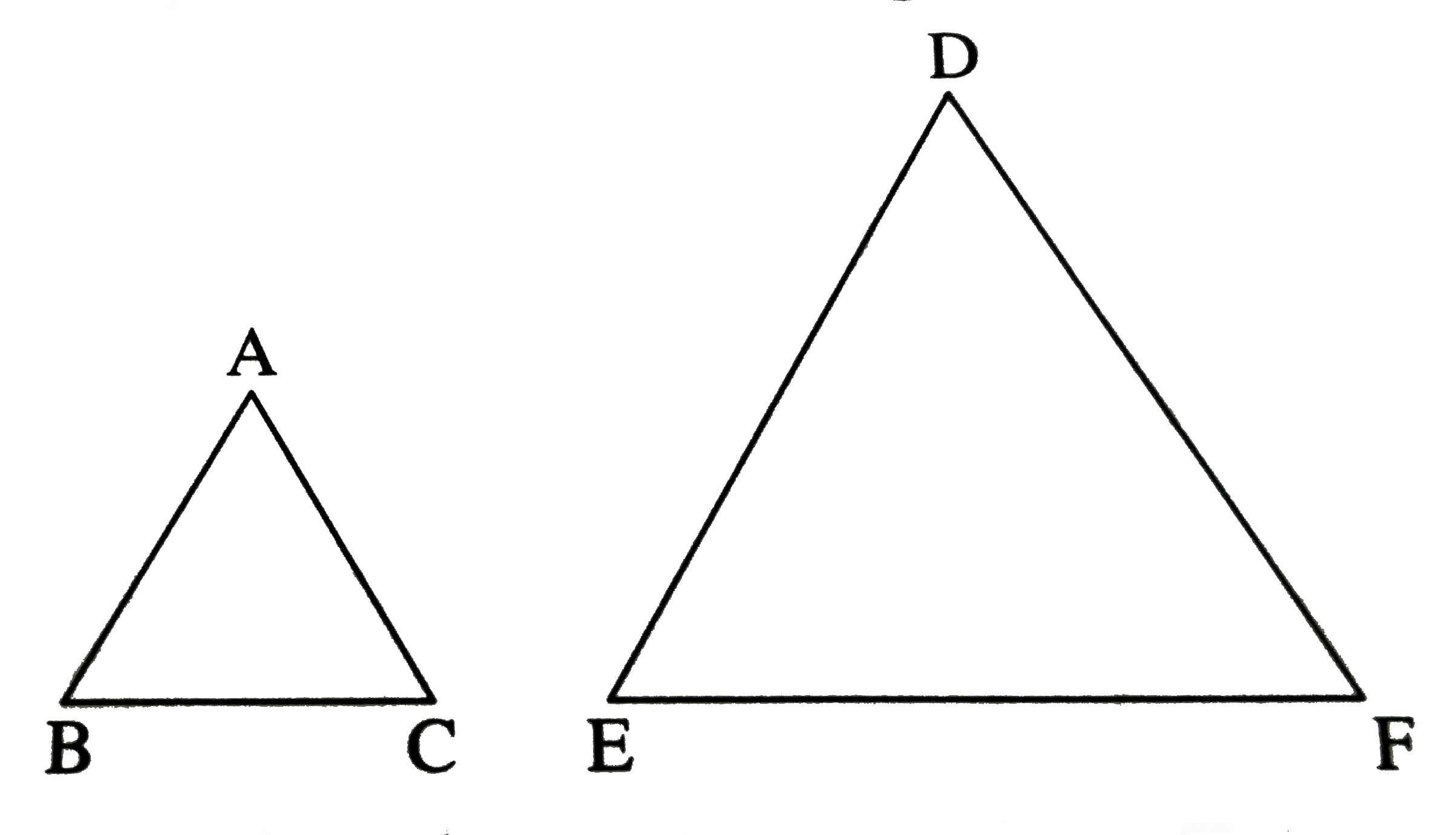
View SolutionΔABC and ΔDEF are equilateral triangles, A(ΔABC):A(ΔDEF)=1:2
If AB=4 then what is length of DE?
View Solution
