Describe Wheatstone's network with a neat circuit diagram. Obtain the expression for its balance condition.
Wheatstone Bridge Network
Circuit Diagram:
A Wheatstone bridge consists of four resistors arranged in a diamond shape. The circuit diagram can be represented as follows:
P±−−−±−−−+||||RQ||||±−−−±−−−+S
Where:
- P, Q, R, and S are the resistances.
- A galvanometer (G) is connected between the junctions of P and R, and Q and S.
- A battery is connected across the bridge to provide the necessary voltage.
Balance Condition Derivation
The balance condition of a Wheatstone bridge occurs when the potential difference across the galvanometer is zero, meaning no current flows through it. This condition can be expressed mathematically.
1. Applying Kirchhoff's Laws:
- In the Wheatstone bridge, we can apply Kirchhoff's loop rule to the two loops formed by the resistors and the galvanometer.
2. For Loop 1 (P and R):
- The voltage drop across P is VP=IP⋅P
- The voltage drop across R is VR=IR⋅R
3. For Loop 2 (Q and S):
- The voltage drop across Q is VQ=IQ⋅Q
- The voltage drop across S is VS=IS⋅S
4. Setting Up the Equations:
- When the bridge is balanced, the current through the galvanometer (G) is zero, which means:
VP=VRandVQ=VS
5. Expressing the Ratios:
- From the first loop:
VPVR=PR
- From the second loop:
VQVS=QS
6. Setting the Ratios Equal:
- For the bridge to be balanced:
PR=QS
7. Rearranging the Equation:
- This can be rearranged to give the balance condition:
P⋅S=Q⋅R
Conclusion
The Wheatstone bridge is balanced when the ratio of the resistances in one branch is equal to the ratio of the resistances in the other branch. This condition is crucial for precise measurements in electrical circuits.
---
- P, Q, R, and S are the resistances.
- A galvanometer (G) is connected between the junctions of P and R, and Q and S.
- A battery is connected across the bridge to provide the necessary voltage.
- In the Wheatstone bridge, we can apply Kirchhoff's loop rule to the two loops formed by the resistors and the galvanometer.
- The voltage drop across P is VP=IP⋅P
- The voltage drop across R is VR=IR⋅R
- The voltage drop across Q is VQ=IQ⋅Q
- The voltage drop across S is VS=IS⋅S
- When the bridge is balanced, the current through the galvanometer (G) is zero, which means:
VP=VRandVQ=VS
- From the first loop:
VPVR=PR
- From the second loop:
VQVS=QS
- For the bridge to be balanced:
PR=QS
- This can be rearranged to give the balance condition:
P⋅S=Q⋅R
Topper's Solved these Questions
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - DERIVATIONS-IIEXERCISE - Magnetism1videos
DERIVATIONS-II
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - DERIVATIONS-IIEXERCISE - Electromagnetic Induction6videos
DERIVATIONS-II
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - DERIVATIONS-IIEXERCISE - Electrostatics12videos
DERIVATIONS-II
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - DERIVATIONS-IEXERCISE - Assignments9videos
DERIVATIONS-I
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - DISTINGUISH BETWEENEXERCISE - Assingment3videos
DISTINGUISH BETWEEN
Similar Questions
Knowledge Check
- Question 1 - Select One
For resistances 10Ω,10Ω,10Ωand15Ω form a Wheatstone's network. What shunt is required across 15Ω resistor to balance the bridge ?
A10ΩB15ΩC20ΩD30Ω - Question 2 - Select One
The wheatstone's network is most sensitive when the ratio of its arms is
A1:1BzeroC10:1D1:100 - Question 3 - Select One
In the circuit given, the correct relation to a balanced Wheatstone bridge is
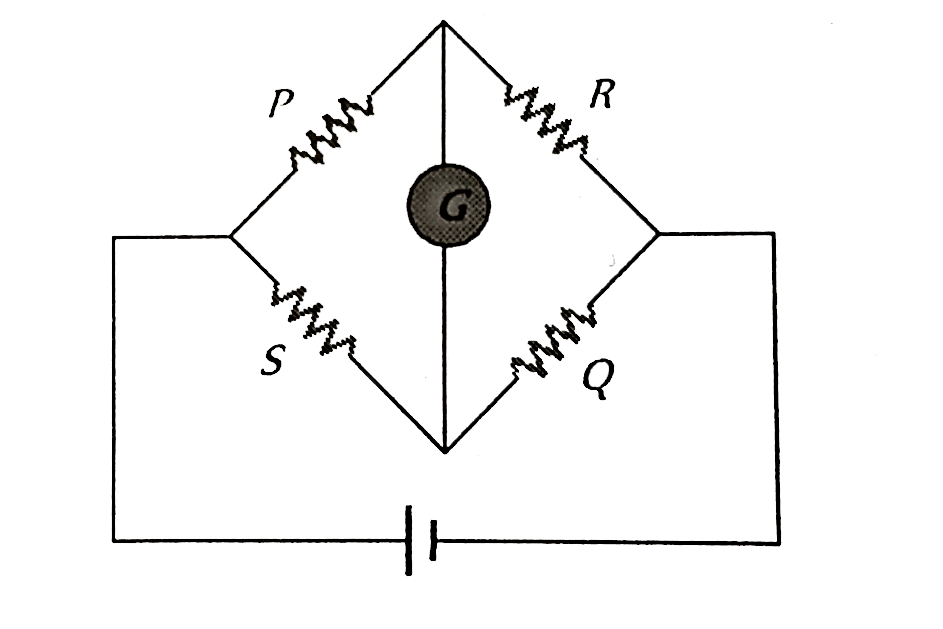 APQ=RSBPQ=SRCPR=SQDNone of these
APQ=RSBPQ=SRCPR=SQDNone of these
Draw the circuit diagram of a metre bridge to explain how it is based on Wheatstone bridge.
View SolutionDescribe an inclined plane and obtain an expression for its mechanical advantage.
View Solution(a) Derive the conduction of balance for wheatstone bridge. (b) Draw the circuit diagram of a meter bridge to explain how it is based on wheatstone bridge.
View SolutionThe angular width of the central maximum of the diffraction patternn in a single slit (of width a) experiment, with λ as the wavelenth of light, is
View SolutionExplain the determination of unknown resistance using meter bridge.
View Solution

