Dorbish Bowley’s Price Index Number is
P01(L)+P01(P)
P01(L)−P01(P)
P01(L)+P01(P)2×100
P01(L)+P01(P)2
The correct Answer is:D
To find Dorbish Bowley’s Price Index Number, we need to understand that it is calculated as the average of two price index numbers: the last prices price index number and the passes price index number. Here’s how to derive it step by step:
1. Identify the Price Index Numbers:
- Let P01 be the Last Prices Price Index Number.
- Let P02 be the Passes Price Index Number.
2. Formula for Dorbish Bowley’s Price Index Number:
- The formula for Dorbish Bowley’s Price Index Number (denoted as PDB) is given by:
PDB=P01+P022
- This formula indicates that we take the average of the two index numbers.
3. Substituting Values:
- If specific values for P01 and P02 are provided in a problem, substitute them into the formula.
- For example, if P01=120 and P02=80, then:
PDB=120+802
4. Calculating the Average:
- Perform the addition:
120+80=200
- Now divide by 2:
PDB=2002=100
5. Conclusion:
- The Dorbish Bowley’s Price Index Number is 100 in this example.
- If the question provides specific values, replace them accordingly to find the actual index number.
- Let P01 be the Last Prices Price Index Number.
- Let P02 be the Passes Price Index Number.
- The formula for Dorbish Bowley’s Price Index Number (denoted as PDB) is given by:
PDB=P01+P022
- This formula indicates that we take the average of the two index numbers.
- If specific values for P01 and P02 are provided in a problem, substitute them into the formula.
- For example, if P01=120 and P02=80, then:
PDB=120+802
- Perform the addition:
120+80=200
- Now divide by 2:
PDB=2002=100
- The Dorbish Bowley’s Price Index Number is 100 in this example.
- If the question provides specific values, replace them accordingly to find the actual index number.
Topper's Solved these Questions
- QUESTION BANK 2021BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Part II 5. INDEX NUMBERS (II Fill in the blanks.)
8 Videos
- QUESTION BANK 2021BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Part II 5. INDEX NUMBERS (III) State whether each of the following is True or False.)
9 Videos
- QUESTION BANK 2021BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUESTION BANK 2021EXERCISE - Part II 4. TIME SERIES (V) Activity based questions)
4 Videos
- PROBABILITY DISTRIBUTIONBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PROBABILITY DISTRIBUTIONEXERCISE - MULTIPLE CHOICE QUESTIONS
9 Videos
- THREE DIMENSIONAL GEOMETRY BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - MULTIPLE CHOICE QUESTIONS
8 Videos
Similar Questions
Knowledge Check
Fisher’s Price Index Number is
A√P01(L)×P01(P)BP01(L)×P01(P)C√P01(L)×P01(P)×100D√P01(L)×P01(P)We use price index numbers
ATo measure pricesBTo measure and compare prices,CTo measure quantityDNone of theseWalsh's Price Index Number is given by
A∑(q0√p0p1)∑(q1√p0p1)×100B∑(p0√q0q1)∑(p1√q0q1)×100C∑(q1√p0p1)∑(q0√p0p1)×100D∑(p1√q0q1)∑(p0√q0q1)×100
The average of Laspeyre’s and Paasche’s Price Index Numbers is called……………..Price Index Number.
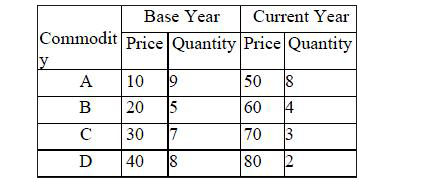
View SolutionIf Laspeyre’s and Paasche’s Price Index Numbers are 50 & 72 respectively, find Dorbish-Bowley’s and Fisher’s Price Index Numbers
View SolutionFisher's Price Index Number is given by ..............
View SolutionMarshall-Edgeworth's Price Index Number is given by …………..
View SolutionState true or False: ∑p1q1p0q1×100 is Paasche’s Price Index Number.
View Solution