Question

Draw a circle of radius 4.2 cm and centre O. Mark a point P at a distance of 7 cm from the centre. Draw tangents to the circle from Points P. (March '19)

Explanation

Analysis :
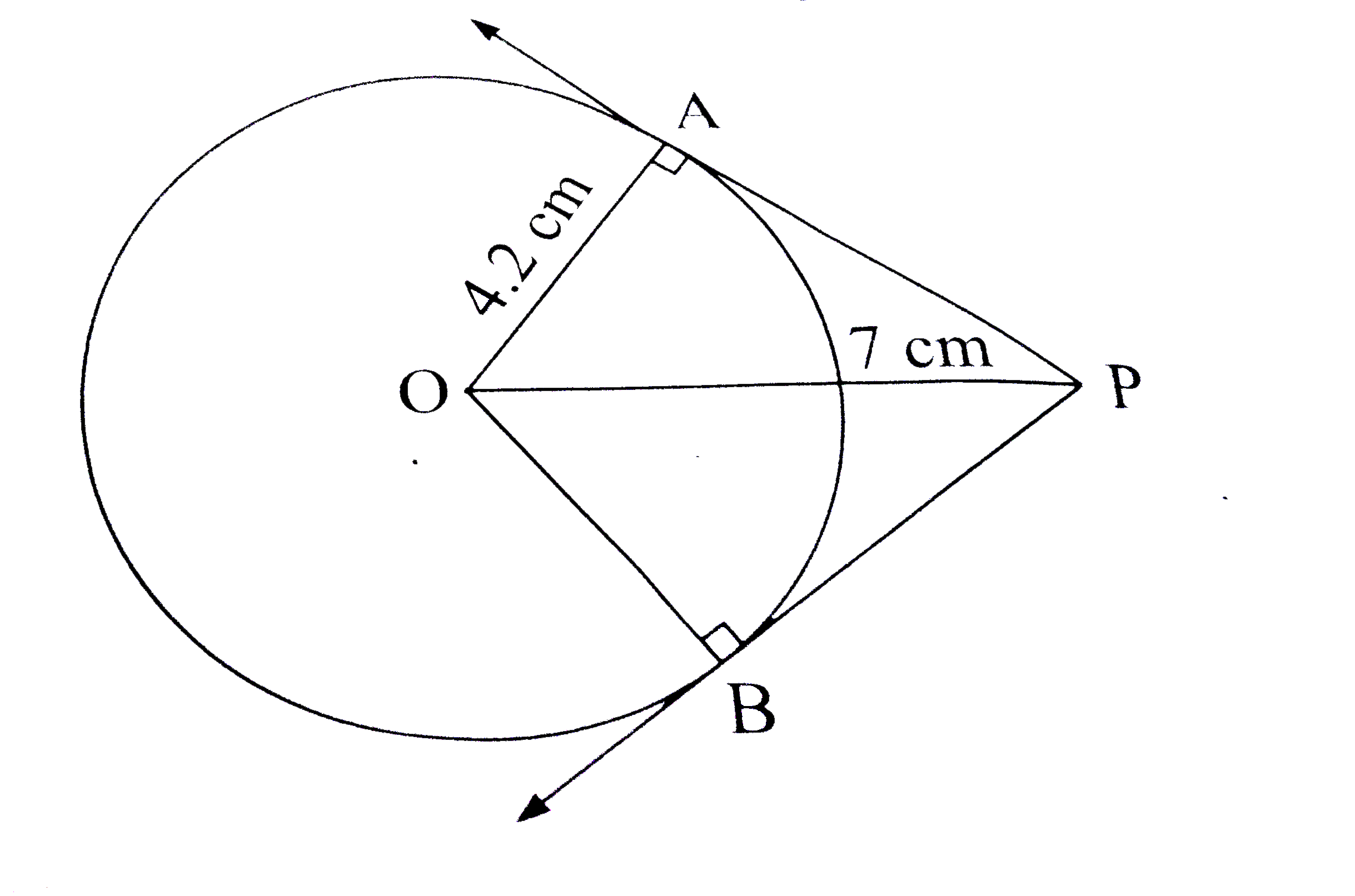
A circle of radius 4.2 cm can be drawn and point P at a distance of 7 cm can be located.n Consider the following analytical figure.
Suppose tangents through P touch the circle at point A and B then
`angleOAP=angleOBP=90^@" "("Tangent theorem")`
we know, ' Angle inscribed in a semi-circle is a right angle. '
`therefore` A and B lie on the semicircular arcs whose disameter is OP
A and B therefore would be the points of intersection of semicircular arcs with the circle.
`therefore` On drawing the perpendicular bisector of seg OP we can obtained the centre and the radius of the semicircular arcs.
Ponits of intersection of semicircular arcs and the circle are points A and B
`therefore` Tangents PA and PB can be drawn
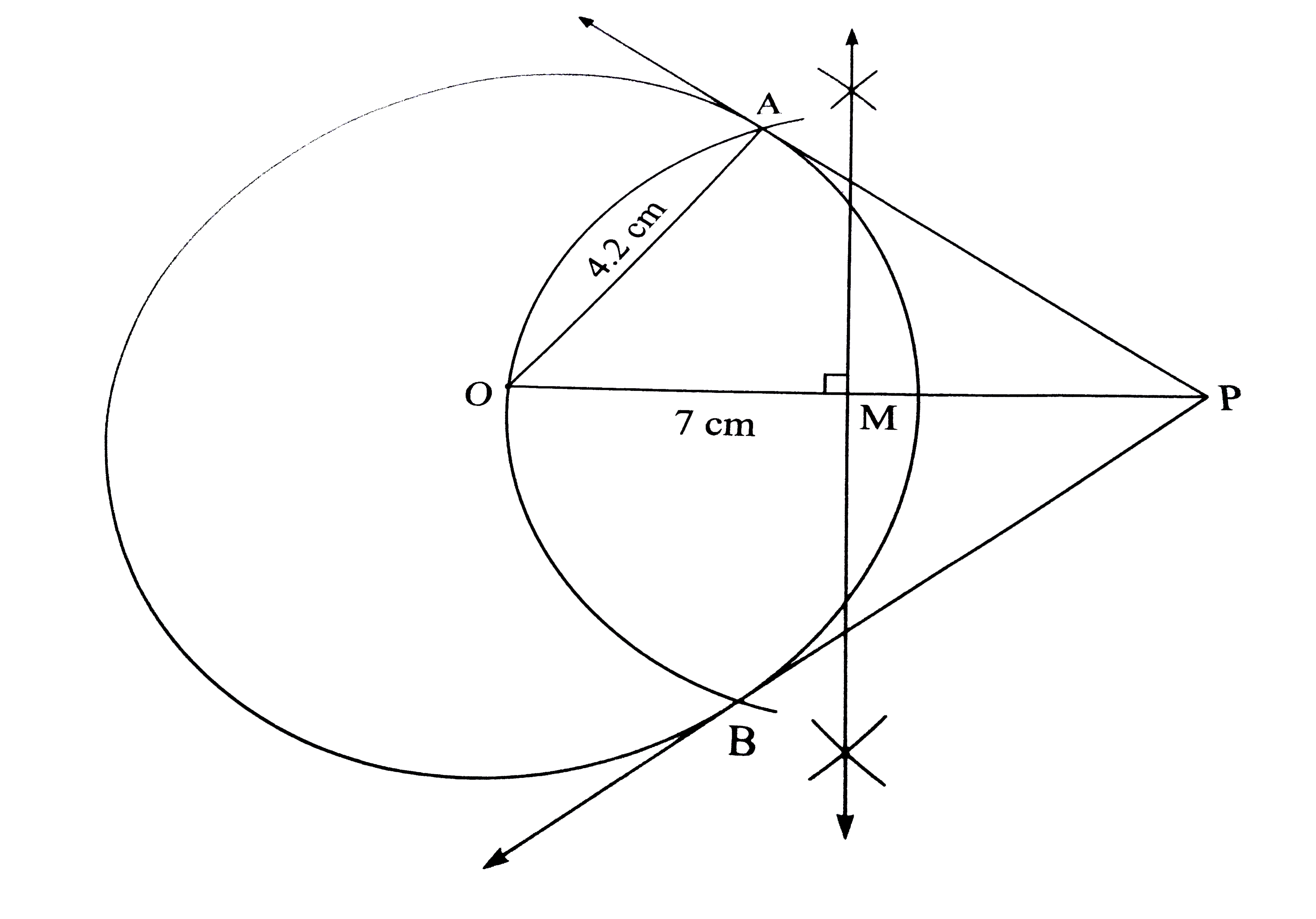
Steps of construction
(1) Draw a circle of radius 4.2 cm.
(2) Take a point P in the exterior of the circle such that d(O,P)= 7 cm
(3) Draw seg OP. Draw perpendicular bisector of seg OP to get its midpoint M
(4) Draw and arc with radius OM and centre M
(6) Draw line PA and PB
Construction :