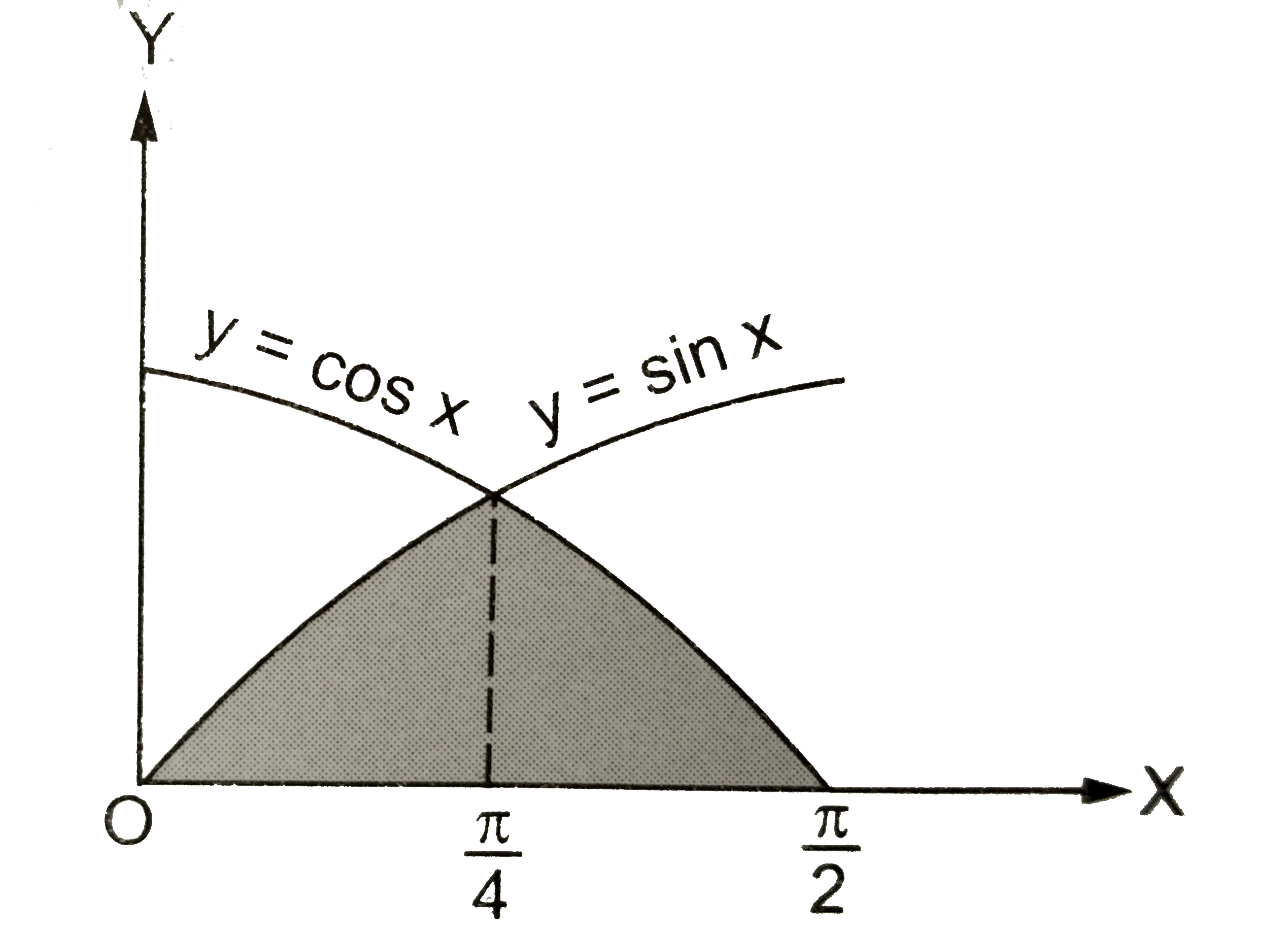
Draw a rough sketch of the curves y=sin x and y= cos x as x varies from 0 to π2 and find the area of the region enclosed between them and the x-axis
A rough sketch of y = sin and y = cos x form x=0 to x=π2 is shown hereewith
Required area =π/4∫0sinxdx+π/2∫π/4cosxdx
Topper's Solved these Questions
- AREA OF BOUNDED REGIONS BOOK - RS AGGARWALCHAPTER - AREA OF BOUNDED REGIONS EXERCISE - Exercise 17
39 Videos
- APPLICATIONS OF DERIVATIVESBOOK - RS AGGARWALCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Objective Questions
89 Videos
- BAYES'S THEOREM AND ITS APPLICATIONSBOOK - RS AGGARWALCHAPTER - BAYES'S THEOREM AND ITS APPLICATIONSEXERCISE - Exercise 30
18 Videos
Similar Questions
Draw a rough sketch of the curves y=sinx and y=cosx as x varies from 0 to π2. Find the area of the region enclosed by the curves and the y-axis.
View SolutionDraw a rough sketch of the curves y=sinxand y=cosx varies from 0 to π2 and find the area of the region enclosed by them and x-axis
View Solution
Knowledge Check
Area of the region enclosed between the curve x=1−y2 and the Y-axis is
A53B43C23D13
Find the area of the region enclosed between curves y=|x−1| and y=3−|x|.
View SolutionDraw the rough sketch of the curve y=x4−x2.
View SolutionFind the area of the region enclosed by the given curvesy=cosx,y=1−2xπ
View SolutionFind the area of the region enclosed by the curves y=xlogxandy=2x−2x2.
View SolutionFind the area of the region enclosed by curves y=sinx and y=cosx as x varies 0 to π2 with x-axis
View SolutionDraw a rough sketch of the curves y2=x+1andy2=−x+1 and find the area enclosed between them,
View SolutionMake a rough sketch of the graph of the function y=2sinx,0≤x≤π2 and determine the area enclosed between the curve, the x-axis and the line x=π2.
View Solution
