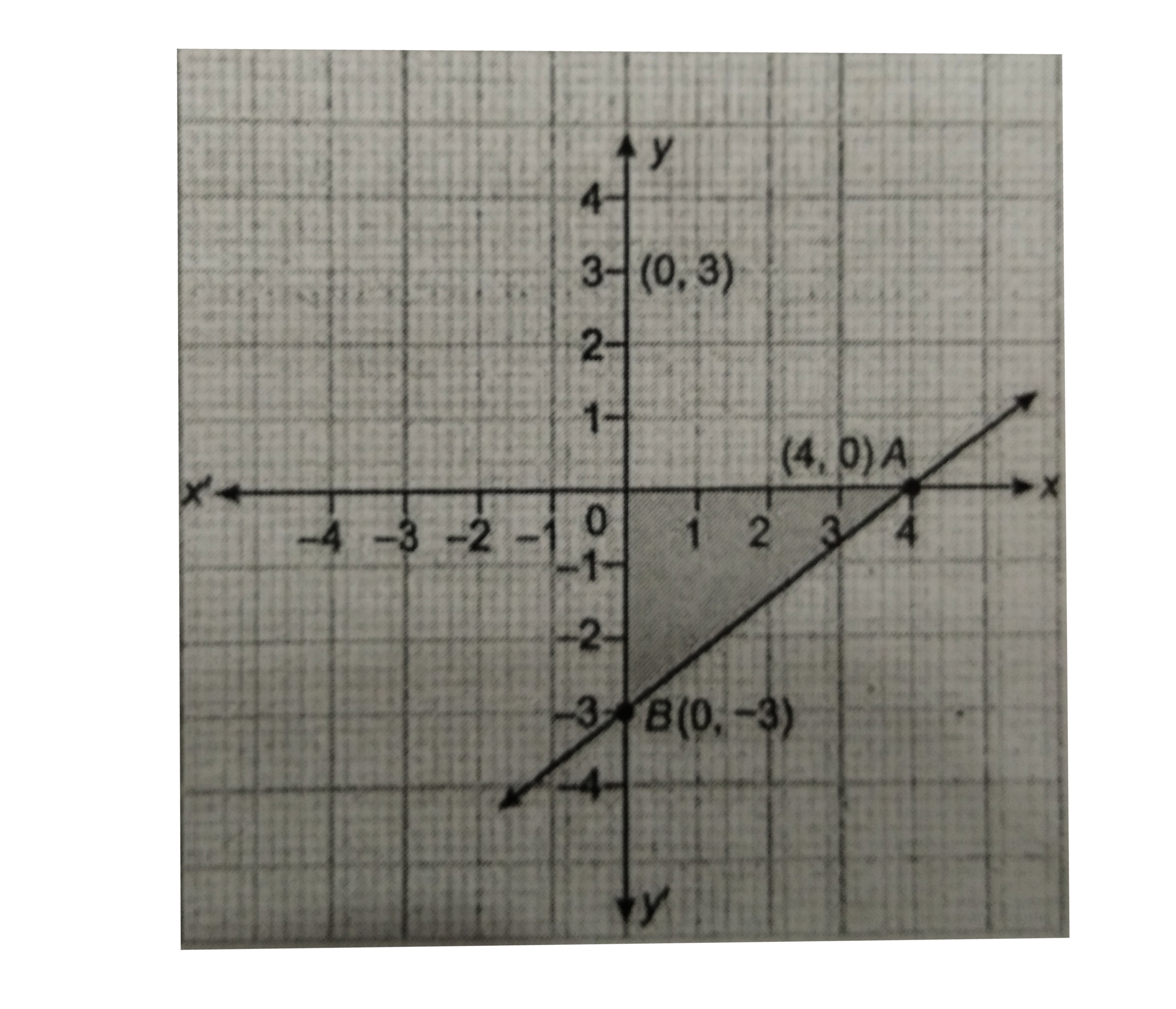
Draw the graph of the equation 3x-4y =12. comment on:
(i)x=0, y=3 is a solution of the equation.
(ii) The abscissa i.e. the value of x can never be 100 units.
(iii) Sum of intercept (parts made by straight line on the axes) on the axed is 7 units.
(iv) Length of line segment between the axes is 5 units
(v) Area of triangle formed by the line 3x-4y =12 and co ordinate axes.

Text Solution

Verified by Experts

We have
3x-4y =12
3x=4y+12
x=4y+12
3
Let y =0 $\Rightarrow$ x=4
Let y = -3 $\Rightarrow$ x= 0
Table for above values

Plot the points A(4,0) and B(0,-3) on the graph paper and join them . producer this line on both sides
(i) we see form the graph , that (0,3) does not lie on the given equation . so x=0 , y=3 is not the solution of
3x-4y=12
Also we can check algebraically (without seeing the graph)
putting x=0 and y =3 in 3x-4y=12 we get
3(0) - (3) =12
$\Rightarrow$ -12=12 which is not true.
Spo , x =0,y =3 connot be the solution of given eqautio 3x-4y = 12
(ii) if we take any real value of x, then we get the corresponding value of y and vice versa from the equation 3x-4y =12 so x= 100 can also be taken
(iii) From the graph we see the intercept made by line on the y axis =-3
so sum of intercepts =4+(-3)=1
There , it is not true that sunm of inteceps is 7.
(iv) Since length of OA =4 units and length of OB =3 units $∴$ In right angle triangle AOB by pythagoras stheorem
$A B^{2} = O A^{2} + O B^{2}$
$= {(4)}^{2} + {(3)}^{2} = 16 + 9 = 25$
$A B = \sqrt{25} = 5 u n i t s$
So length of line segment AB =5 units
(v) Area of triangle = $\frac{1}{2} \times O A \times O B = \frac{1}{2} \times 4 \times 3$ units
=6 sq units

Find the intercepts made by the line 3x+4y - 12 =0 on the axes :
Aa. 2 and 3
Bb. 4 and 3
Cc. 3 and 5
Dd. none of these
Find the intercepts made by the lines 3x + 4y - 12 = 0 on the axes:
A2 and 3
B4 and 3
C3 and 5
Dnone
The length of the portion of the straight line $3 x + 4 y = 12$ intercepted between the axes is
A5
B3
C4
D7