Each coefficient in the equation $a x^{2} + b x + c = 0$ is obtained by rolling a die. Find the probability that the equation has equal roots.

Video Solution

Text Solution

Verified by Experts

The three coefficient a,b and c are determined by rolling a die three times.
Each die has six possibilities.
$∴$ the sample space has $6 \times 6 \times 6 = 216$ points.
$∴ n (S) = 216$
Let A be the event that the equation has equal roots.
Then the discriminant $(Δ) = b^{2} - 4 a c = 0$ .

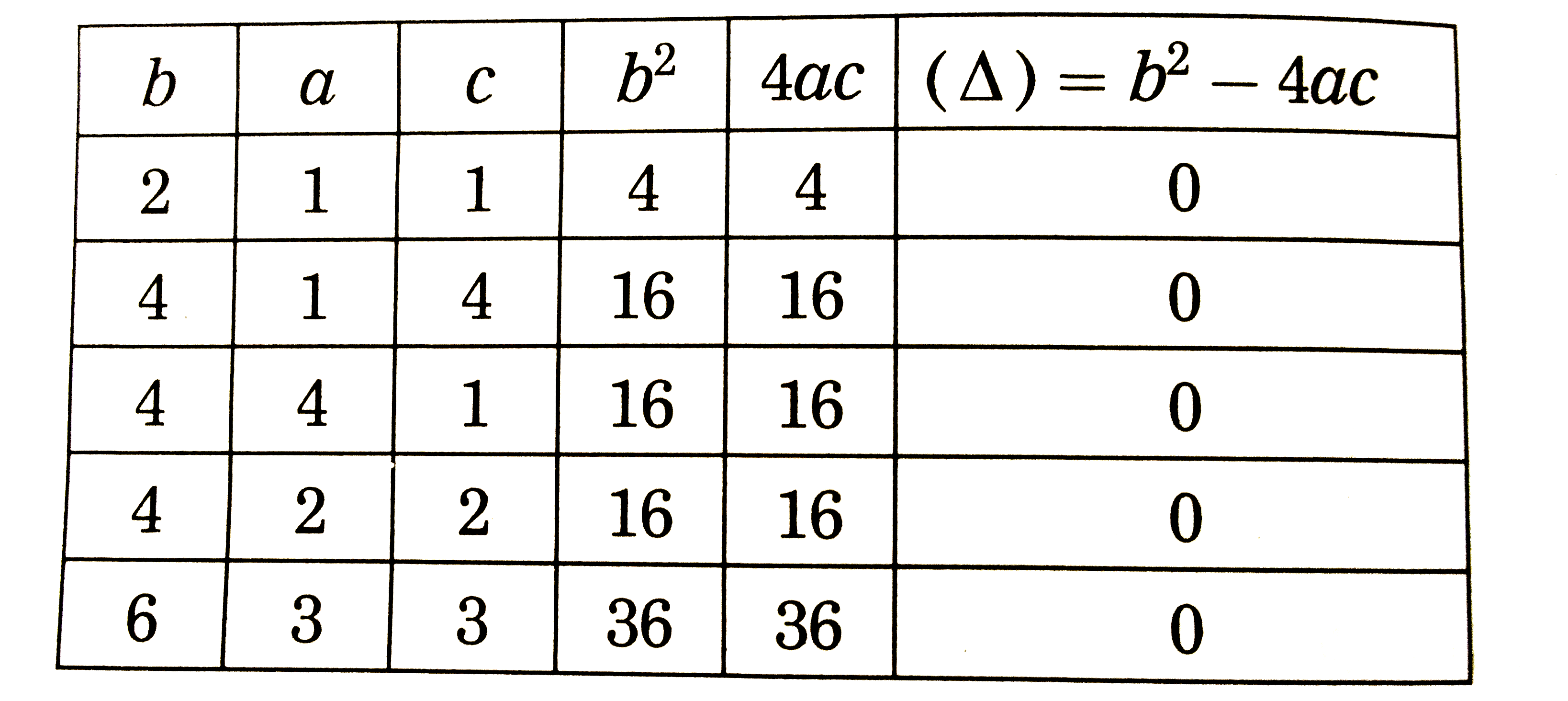
$A = {(2, 1, 1), (4, 1, 4), (4, 4, 1), (4, 2, 2), (6, 3, 3)}$
$: n (A) = 5$
$∴ P (A) = \frac{n (A)}{n (S)} ∴ P (A) = \frac{5}{216}$
Ans. The probability that the equation $a x^{2} + b x + c = 0$ has equal roots is $\frac{5}{216}$ .

|

Updated on:21/07/2023

Class 10 MATHS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If a sum of money double itself in 4 years at S.I. then the rate of interest per annum is?
Aa. 25%
Bb. 100%
Cc. 50%
Dd. none of these
Question 2 - Select One
The coefficients a, b and c of the quadratic equation, $a x^{2} + b x + c = 0$ are obtained by throwing a dice three times. The probability that this equation has equal roots is:
A $\frac{1}{72}$
B $\frac{5}{216}$
C $\frac{1}{36}$
D $\frac{1}{54}$
Question 3 - Select One
If 'head' means one and 'tail' means two,then coefficient of quadratic equation $a x^{2} + b x + c = 0$ are chosen by tossing three fair coins. The probability that roots of the equating are imaginary is
A $5 / 8$
B $3 / 8$
C $7 / 8$
D $1 / 8$ .

NAVNEET PUBLICATION - MAHARASHTRA BOARD-CHALLENGING QUESTIONS -EXAMPLE FOR PRACTICE

Home
Ask Doubt
Profile