Each of the letters H,N,S and Z has a rotational symmetry of order ____________.
To determine the rotational symmetry of the letters H, N, S, and Z, we need to analyze each letter and see how many times it looks the same when rotated within a full circle (360 degrees).
1. Understanding Rotational Symmetry:
- Rotational symmetry refers to the number of times a shape or figure can be rotated around a central point and still look the same. The order of rotational symmetry is the total number of such rotations in a full 360-degree turn.
2. Analyzing the Letter H:
- When we rotate the letter H by 180 degrees, it looks the same.
- If we rotate it again by another 180 degrees (making it a total of 360 degrees), it still looks the same.
- Therefore, the letter H has a rotational symmetry of order 2.
3. Analyzing the Letter N:
- Similar to H, when we rotate the letter N by 180 degrees, it also looks the same.
- Rotating it again by another 180 degrees (totaling 360 degrees) keeps it unchanged.
- Thus, the letter N also has a rotational symmetry of order 2.
4. Analyzing the Letter S:
- When we rotate the letter S by 180 degrees, it appears as a mirrored version of itself.
- Rotating it again by another 180 degrees (360 degrees in total) brings it back to its original position.
- Hence, the letter S has a rotational symmetry of order 2.
5. Analyzing the Letter Z:
- For the letter Z, when it is rotated by 180 degrees, it appears the same as well.
- A second rotation of 180 degrees (totaling 360 degrees) also keeps it unchanged.
- Therefore, the letter Z has a rotational symmetry of order 2.
6. Conclusion:
- All the letters H, N, S, and Z have a rotational symmetry of order 2.
Final Answer:
Each of the letters H, N, S, and Z has a rotational symmetry of order 2.
- Rotational symmetry refers to the number of times a shape or figure can be rotated around a central point and still look the same. The order of rotational symmetry is the total number of such rotations in a full 360-degree turn.
- When we rotate the letter H by 180 degrees, it looks the same.
- If we rotate it again by another 180 degrees (making it a total of 360 degrees), it still looks the same.
- Therefore, the letter H has a rotational symmetry of order 2.
- Similar to H, when we rotate the letter N by 180 degrees, it also looks the same.
- Rotating it again by another 180 degrees (totaling 360 degrees) keeps it unchanged.
- Thus, the letter N also has a rotational symmetry of order 2.
- When we rotate the letter S by 180 degrees, it appears as a mirrored version of itself.
- Rotating it again by another 180 degrees (360 degrees in total) brings it back to its original position.
- Hence, the letter S has a rotational symmetry of order 2.
- For the letter Z, when it is rotated by 180 degrees, it appears the same as well.
- A second rotation of 180 degrees (totaling 360 degrees) also keeps it unchanged.
- Therefore, the letter Z has a rotational symmetry of order 2.
- All the letters H, N, S, and Z have a rotational symmetry of order 2.
Each of the letters H, N, S, and Z has a rotational symmetry of order 2.
Topper's Solved these Questions
- PRACTICAL GEOMETRY SYMMETRY AND VISUALISING SOLID SHAPESBOOK - NCERT EXEMPLARCHAPTER - PRACTICAL GEOMETRY SYMMETRY AND VISUALISING SOLID SHAPESEXERCISE - THINK AND DISCUSS
6 Videos
- PERIMETER AND AREABOOK - NCERT EXEMPLARCHAPTER - PERIMETER AND AREAEXERCISE - Think and Discuss
7 Videos
- RATIONAL NUMBERSBOOK - NCERT EXEMPLARCHAPTER - RATIONAL NUMBERSEXERCISE - Application
2 Videos
Similar Questions
Knowledge Check
A square has a rotational symmetry of order
A1B2C3D4Which has no rotation of symmetry ?
AhexagonalBOrthochromicCCubicDTriclinicWhich has no rotaition of symmetry ?
AHexagonalBOrthorhombicCCubicDTriclinic
Order of rotational symmetry of
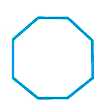
is _____________.
View SolutionOrder of rotational symmetry of a circle is ___________.
View SolutionOrder of rotational symmetry of a rectangle is ____________.
View SolutionRhombus is a figure that has _______lines of symmetry and has a rotational symmetry of order__________.
View SolutionThe given figure has rotational symmetry of order
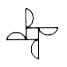
View Solution

 ___________.
___________.