Each question has four choices a, b, c, and d, out of which only one is correct. Each question contains STATEMENT 1 and STATEMENT 2. a. Both the statements are TRUE and statement 2 is the correct explanation for Statement 1. b. Both the statements are TRUE but Statement 2 is NOT the correct explanation for Statement 1. c. Statement 1 is TRUE and Statement 2 is FALSE. d. Statement 1 is FALSE and Statement 2 is TRUE. A vector has components and 1 with respect to a rectangular Cartesian system. The axes are rotted through an angel about the origin the anticlockwise sense. Statement 1: IF the vector has component and 1 with respect to the new system, then Statement 2: Magnitude of the origin vector and the new vector remains the same.Solution in Tamil
Both the statements are true, and Statement 2 is the correct explanation for Statement 1.
Both the statements are true, but Statement 2 is not the correct explanation for Statement 1.
Statement 1 is true and Statement 2 is false.
Statement 1 is false and Statement 2 is true.
The correct Answer is:A
or
or
or
Hence, a is the correct option.
Topper's Solved these Questions
- INTRODUCTION TO VECTORS BOOK - CENGAGECHAPTER - INTRODUCTION TO VECTORS EXERCISE - Exercise (Comprehension)
9 Videos
- INTRODUCTION TO VECTORS BOOK - CENGAGECHAPTER - INTRODUCTION TO VECTORS EXERCISE - LINKED COMPREHENSION TYPE
2 Videos
- INTRODUCTION TO VECTORS BOOK - CENGAGECHAPTER - INTRODUCTION TO VECTORS EXERCISE - Exercise (Multiple)
13 Videos
- INTEGRALSBOOK - CENGAGECHAPTER - INTEGRALSEXERCISE - Solved Examples And Exercises
222 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBOOK - CENGAGECHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - All Questions
529 Videos
Similar Questions
ABCD is a parallelogram. L is a point on BC which divides BC in the ratio 1:2. AL intersects BD at P.M is a point on DC which divides DC in the ratio 1:2 and AM intersects BD in Q.
Point P divides AL in the ratio
View SolutionLet OABCD be a pentagon in which the sides OA and CB are parallel and the sides OD and AB are parallel. Also OA:CB=2:1andOD:AB=1:3.

The ratio OXXC is
View SolutionThe vectors xˆi+(x+1)ˆj+(x+2)ˆk,(x+3)ˆi+(x+4)ˆj+(x+5)ˆkand(x+6)ˆi+(x+7)ˆj+(x+8)ˆk are coplanar if x is equal to
View SolutionStatement 1: For −1<a<4,∫dxx2+2(a−1)x+a+5 =λlog|g(x)|+c, where λandc are constants Statement 2: For −1<a<4,1x2+2(a−1)x+a+5iscont∈uouunction
View SolutionFind x satisfying [tan−1x]+[cot−1x]=2, where [.] represents the greatest integer function.
View SolutionEach question has four choices a,b,c and d out of which only one is correct. Each question contains Statement 1 and Statement 2. Make your answer as: If both the statements are true and Statement 2 is the correct explanation of statement 1. If both the statements are True but Statement 2 is not the correct explanation of Statement 1. If Statement 1 is True and Statement 2 is False. If Statement 1 is False and Statement 2 is True. It is given that a≻,b>0,c>0anda+b+c=abc Statement 1: All of the three numbers cannot be less than √3. Statement 2: In ABC. tanA+tanB+tanC=tanAtanBtanC
View SolutionEach question has four choices a,b,c and d out of which only one is correct. Each question contains Statement 1 and Statement 2. Make your answer as: a) If both the statements are true and Statement 2 is the correct explanation of statement 1. b) If both the statements are True but Statement 2 is not the correct explanation of Statement 1. c) If Statement 1 is True and Statement 2 is False. d) If Statement 1 is False and Statement 2 is True. Statement 1: If A,B,C are the angles of a triangle such that angle A is obtuse, then tanBtanC>1. Statement 2: In any triangle, tanA=tanB+tanCtanBtanC−1
View SolutionEach question has four choice: a, b, c and d, out of which only one is correct. Each question contains Statement 1 and Statement 2. Find the correct answer. Both the Statements are true but Statement 2 is the correct explanation of Statement 1. Both the Statement are True but Statement 2 is not the correct explanation of Statement 1. Statement 1 is True and Statement 2 is False. Statement 1 is False and Statement 2 is True Statement 1: The lines (a+b)x+(a−2b)y=a are con-current at the point (23,13). Statement 2: The lines x+y−1=0 and x−2y=0 intersect at the point (23,13).
View SolutionEach question has four choices a, b, c, and d, out of which only one is correct. Each question contains STATEMENT 1 and STATEMENT 2. Both the statements are TRUE and statement 2 is the correct explanation of Statement 1. Both the statements are TRUE but Statement 2 is NOT the correct explanation of Statement 1. Statement 1 is TRUE and Statement 2 is FALSE. Statement 1 is FALSE and Statement 2 is TRUE. Statement 1: For events AandB of sample space if P(AB)≥P(A) , then P(BA)≥P(B). Statement 2: P(AB)=P(A∩B)P(B) , (P(B)≠0).
View SolutionEach question has 5 choices (a) ,(b) ,(c ) (d) and (e ) out of which ONLY ONE is correct.
(a) Statement -1 is True, Statement -2 is True , Statement -2 is a correct explanation for Statement -1.
(b) Statement -1 is True, Statement -2 is True, Statement -2 is NOT a correct explanation for Statement -1.
(C ) Statement -1 is True, Statement -2 is False.
(d) Statement -1 is False, Statement -2 is True.
(e) Statement -1 and Statement -2 both are False.
Statement -1: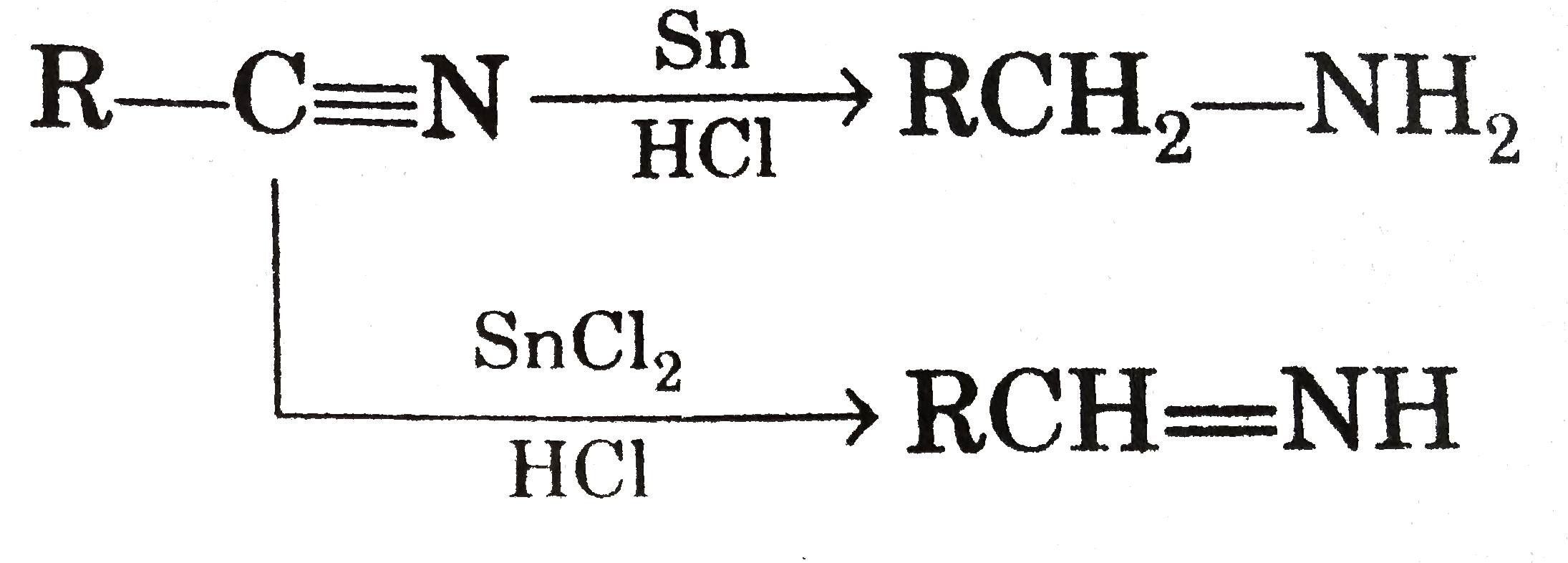
Statement-2: Sn can give maximum of 4 electron during reduction, whereas SnCl2 can give only 2.
View SolutionEach question has four choices a,b,c and d out of which only one is correct. Each question contains Statement 1 and Statement 2.
Make your answer as: If both the statements are true and Statement 2 is the correct explanation of statement 1.
If both the statements are True but Statement 2 is not the correct explanation of Statement 1.
If Statement 1 is True and Statement 2 is False.
If Statement 1 is False and Statement 2 is True.
Statement 1: In a triangle, the least value of the sum of cosines of its angles is unity.
Statement 2: cosA+cosB+cosC=1+4sinA2sinB2sinC2,ifA,B,C are the angles of a triangle.
View SolutionEach question has four choices a,b,c and d out of which only one is correct. Each question contains Statement 1 and Statement 2. Make your answer as: If both the statements are true and Statement 2 is the correct explanation of statement 1. If both the statements are True but Statement 2 is not the correct explanation of Statement 1. If Statement 1 is True and Statement 2 is False. If Statement 1 is False and Statement 2 is True. It is given that a≻,b>0,c>0anda+b+c=abc Statement 1: All of the three numbers cannot be less than √3. Statement 2: In ABC. tanA+tanB+tanC=tanAtanBtanC
View SolutionEach question has four choices a,b,c and d out of which only one is correct. Each question contains Statement 1 and Statement 2. Make your answer as: a) If both the statements are true and Statement 2 is the correct explanation of statement 1. b) If both the statements are True but Statement 2 is not the correct explanation of Statement 1. c) If Statement 1 is True and Statement 2 is False. d) If Statement 1 is False and Statement 2 is True. Statement 1: If A,B,C are the angles of a triangle such that angle A is obtuse, then tanBtanC>1. Statement 2: In any triangle, tanA=tanB+tanCtanBtanC−1
View SolutionEach question has four choices a,b,c and d out of which only one is correct. Each question contains Statement 1 and Statement 2. Make your answer as: If both the statements are true and Statement 2 is the correct explanation of statement 1. If both the statements are True but Statement 2 is not the correct explanation of Statement 1. If Statement 1 is True and Statement 2 is False. If Statement 1 is False and Statement 2 is True. If A+B+C=π, then Statement 1: cos2A+cos2B+cos2C has its minimum value 34. Statement 2: Maximum value of cosAcosBcosC is 18
View SolutionEach question has four choices, a,b,c and d, out of which only one is correct. Each question contains STATEMENT 1 and STATEMENT 2. (a) If both the statements are TRUE and STATEMENT 2 is the correct explanation of STATEMENT 1. (b) If both the statements are TRUE but STATEMENT 2 is NOT the correct explanation of STATEMENT 1. (c) If STATEMENT 1 is TRUE and STATEMENT 2 is FALSE. (d) If STATEMENT 1 is FALSE and STATEMENT 2 is TRUE. Statement 1: ∫exsinxdx=ex2(sinx−cosx)+c Statement 2: ∫ex(f(x)+f′(x))dx=exf(x)+c
View SolutionEach question has four choice: a, b, c and d, out of which only one is correct. Each question contains Statement 1 and Statement 2. Find the correct answer. Both the Statements are true but Statement 2 is the correct explanation of Statement 1. Both the Statement are True but Statement 2 is not the correct explanation of Statement 1. Statement 1 is True and Statement 2 is False. Statement 1 is False and Statement 2 is True Statement 1: The lines (a+b)x+(a−2b)y=a are con-current at the point (23,13). Statement 2: The lines x+y−1=0 and x−2y=0 intersect at the point (23,13).
View Solution
