Equation of the straight line meeting the cirle with centre at origin and radius equal to 5 in two points at equal distances of 3 units from the point (3,4) is
A
6x+8y=41
B
6x−8y+41=0
C
8x+6y+41=0
D
8x−6y+41=0
Video Solution
Text Solution
The correct Answer is:A
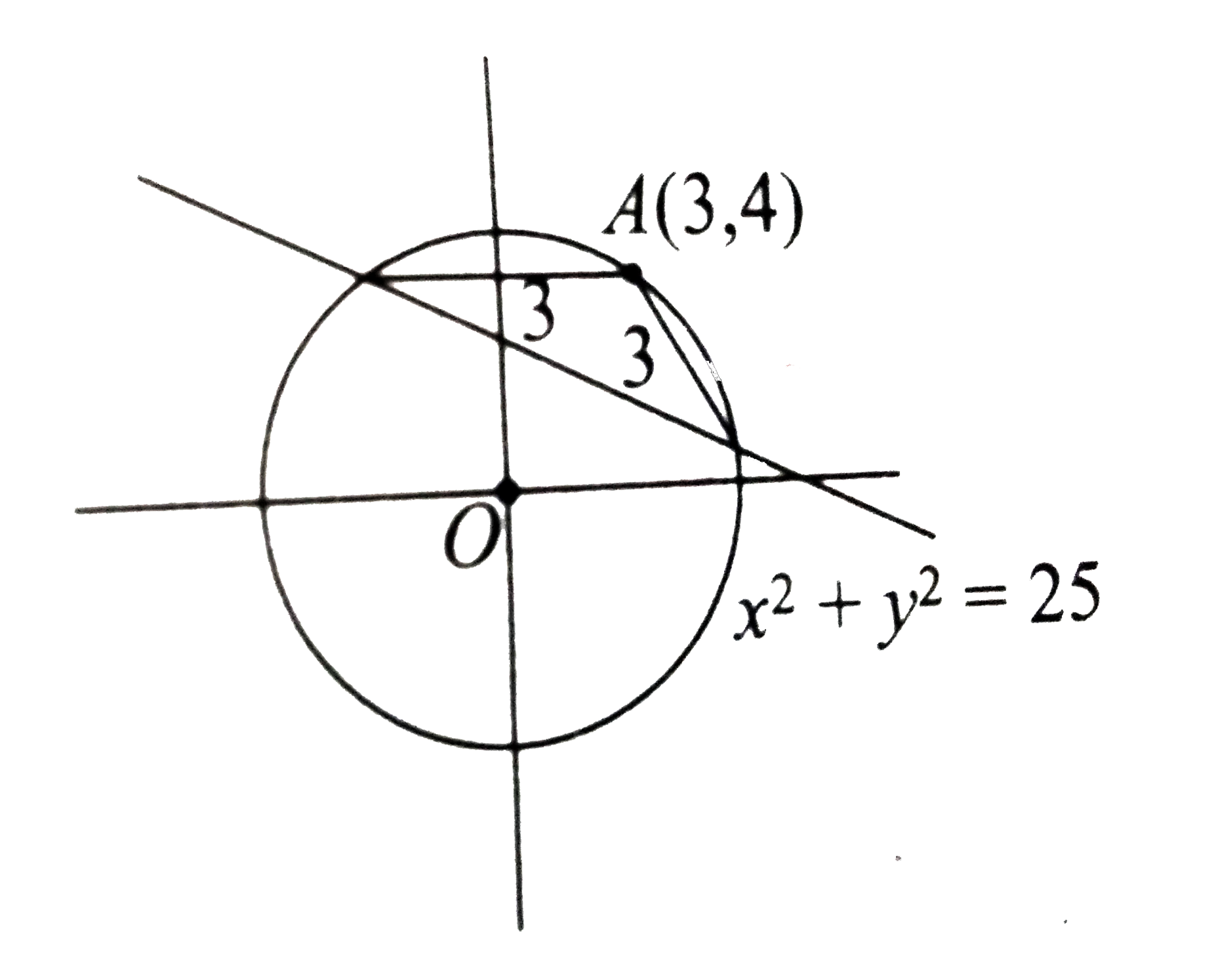
Equation of circle whose centre is at (3,4) and radius is equal to 3 is (x−3)2+(y−4)2=9
Given circle is x2+y2=25.
Now the equation of straight line is the common chord of the two circles.
⇒6x+8y=41
|
Topper's Solved these Questions
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
32 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Comprehension Type
8 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - JEE Advanced (Single Correct Answer Type)
14 Videos
- COMPLEX NUMBERSBOOK - CENGAGECHAPTER - COMPLEX NUMBERSEXERCISE - Question Bank
30 Videos
Similar Questions
Equation of a straight line meeting the circle x2+y2=100 in two points, each point is at a distance of 4 units from the point (8,6) on the circle, is ax+by−46=0 where a,b∈N then a+b is equal to
View SolutionThe number of lines that can be drawn through the point (4,−5) at a distance of 10 units from the point (1,3) is
View Solution
Knowledge Check
Equation of the straight line which meets the circle x2+y2=8 at two points where these points are at a distance of 2 units from the point A(2, 2) is
Ax+y=2Bx+y=3Cx+y=1Dx+y=0The distance of the point p(3,4) from the origin is
A3B4C7D5What point on the x-axis are at a distance of 4 units from the line 3x−4y−5=0
A(13,0)B(0,253)C(5,1)D(253,0)
The distance of the point P(4,3) from the origin is:
View SolutionFind the distance of the point (3, 4) from the origin.
View SolutionThe distance of the point P(-4,3) from the origin is
View SolutionFind the equation of a line perpendicular to the line 3x+y+5=0 and at a distance of 3 units from the origin.
View SolutionDistance of point (-3,4) from the origin is ……….
View Solution
