Equation x4+ax3+bx2+cx+1=0 has real roots (a,b,c are non-negative). Minimum non-negative real value of c is (a) 10 (b) 9 (c) 6 (d) 4
10
9
6
4
The correct Answer is:D
Since, a,b,c ≥ 0 roots must be negative. Let the roots be
x1,x2,x3,x4(<0). Then.
x1+x2+x3+x4=−a
x1x2+x1x3+x1x4+x2x3+x2x4+x3x4=b
x1x2x3+x2x3x4+x3x4x1+x3x4x1+x4x1x2=−c
x1x2x3x4=1
Now, (−x1)+(−x2)+(−x3)+(−x4)4≥[(−x1)(−x2)(−x3)(−x4)]1/4
(∵A.M≥G.M)
⇒a4≥1
Hence, the minimum value of a is 4. similarly,
x1x2+x1x3+.....+x3x46≥[x31x32x33x44]1/4
⇒b6≥1
or b≥6
Hence, the minimum value of is 6. Finally
x1x2x3−x2x3x4−x3x4x1−x4x1x24≥[x31x32x33x34]1/4
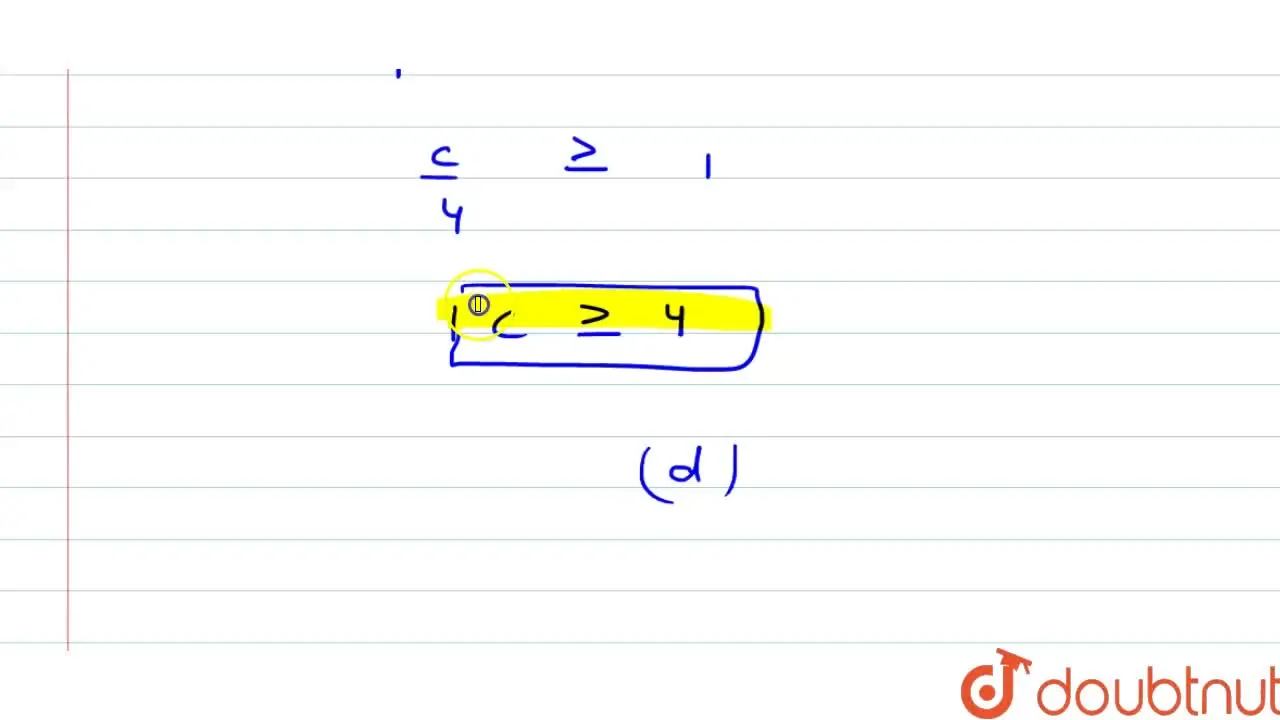
⇒c4≥1
or c ge 4
Hence, the minimum value of c is 4.
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - INEQUALITIES INVOLVING MEANS EXERCISE - Numerical value type10videos
INEQUALITIES INVOLVING MEANS
- BOOK - CENGAGE ENGLISHCHAPTER - INEQUALITIES INVOLVING MEANS EXERCISE - Jee Advanced (Single1videos
INEQUALITIES INVOLVING MEANS
- BOOK - CENGAGE ENGLISHCHAPTER - INEQUALITIES INVOLVING MEANS EXERCISE - Multiple correct answers type5videos
INEQUALITIES INVOLVING MEANS
- BOOK - CENGAGE ENGLISHCHAPTER - INEQUALITIES AND MODULUSEXERCISE - Single correct Answer21videos
INEQUALITIES AND MODULUS
- BOOK - CENGAGE ENGLISHCHAPTER - INTEGRALSEXERCISE - All Questions764videos
INTEGRALS
Similar Questions
Equation x4+ax3+bx2+cx+1=0 has real roots (a,b,c are non-negative).
Minimum non-negative real value of a is (a) 10 (b) 9 (c) 6 (d) 4View SolutionEquation x4+ax3+bx2+cx+1=0 has real roots (a,b,c are non-negative).
Minimum non-negative real value of b is (a) 12 (b) 15 (c) 6 (d) 10View Solutionax2+2bx−3c=0 has no real root and a+b>3c4 then
View SolutionThe quadratic equation ax2+bx+c=0 has real roots if:
View SolutionIf the equation cx2+bx−2a=0 has no real roots and a<b+c2 then
View SolutionIf the equation cx2+bx−2a=0 has no real roots and a<b+c2 then
View SolutionIf the equation f(x)=ax2+bx+c=0 has no real root, then (a+b+c)c is (A) =0 (B) >0 (C) <0 (D) not real
View SolutionIf ax2+bx+c=0 has no real roots and a, b, c ∈ R such that a+c>0, then
View SolutionIf a,b,c are real distinct numbers such that a3+b3+c3=3abc, then the quadratic equation ax2+bx+c=0 has (a) Real roots (b) At least one negative root (c) Both roots are negative (d) Non real roots
View SolutionLet f(x)=ax2+bx+ca ,b ,c∈R. If f(x) takes real values for real values of x and non-real values for non-real values of x , then (a)a=0 (b) b=0 (c) c=0 (d) nothing can be said about a,b,c.
View Solution