Explain how a square is
a parallelogram
The correct Answer is:parallelogram
Step by step video, text & image solution for Explain how a square is a parallelogram by Maths experts to help you in doubts & scoring excellent marks in Class 8 exams.
Updated on:21/07/2023Topper's Solved these Questions
- UNDERSTANDING QUADRILATERALSBOOK - MTG IIT JEE FOUNDATIONCHAPTER - UNDERSTANDING QUADRILATERALSEXERCISE - EXERCISE(MULTIPLE CHOICE QUESTION LEVEL-1)
35 Videos
- UNDERSTANDING QUADRILATERALSBOOK - MTG IIT JEE FOUNDATIONCHAPTER - UNDERSTANDING QUADRILATERALSEXERCISE - EXERCISE(MULTIPLE CHOICE QUESTION LEVEL-2)
13 Videos
- UNDERSTANDING QUADRILATERALSBOOK - MTG IIT JEE FOUNDATIONCHAPTER - UNDERSTANDING QUADRILATERALSEXERCISE - NCERT SECTION (EXERCISE 3.3)
12 Videos
- SQUARES AND SQUARE ROOTSBOOK - MTG IIT JEE FOUNDATIONCHAPTER - SQUARES AND SQUARE ROOTSEXERCISE - Olympiad/HOTS Corner
20 Videos
- VISUALISING SOLID SHAPESBOOK - MTG IIT JEE FOUNDATIONCHAPTER - VISUALISING SOLID SHAPESEXERCISE - OLYMPIAD/HOTS CORNER
10 Videos
Similar Questions
Knowledge Check
Assertion : Square is not a parallelogram.
Reason : The opposite sides of a square are parallel.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf assertion is false but reason is true
Explain how a square is
a rhombus
View SolutionQ3) Explain how a square is: (a) a quadrilateral (b) a parallelogram (c) a rhombus (d) a rectangle
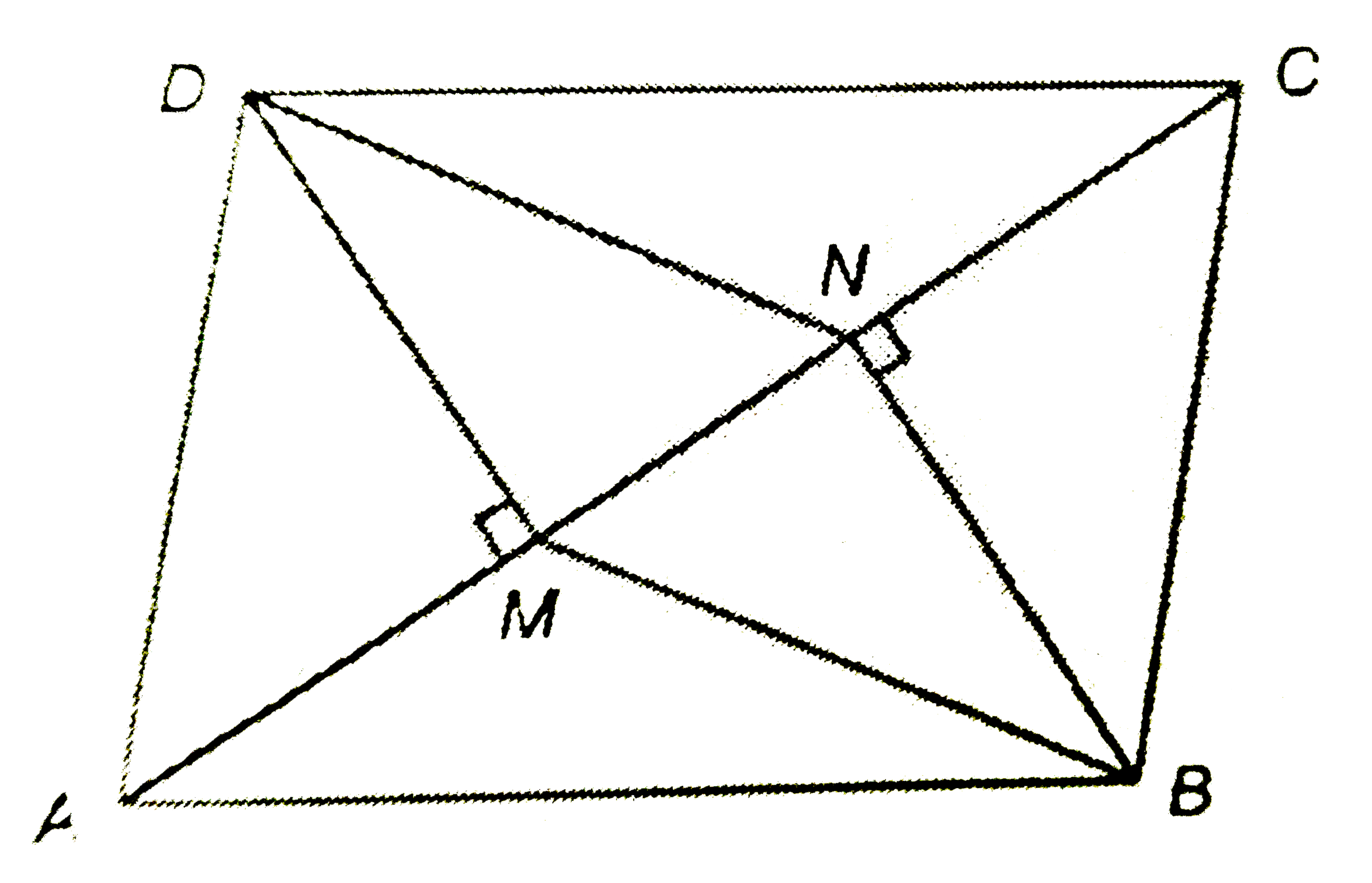
View SolutionIn the given figure, □ABCD is a parallelogram. If DM ⊥ AC and BN ⊥ AC, then show that □BNDM is a parallelogram.
View SolutionIf A(6,1) ,B(8,2) ,C(9,4) and D (7,3) are the vertices of □ ABCD , show that □ ABCD is a parallelogram.
View SolutionShow that □ ABCD is a parallelogram if A (4,8) ,B(5,5), C(2,4) ,D(1,7) .
View SolutionGive reasons for the following :
Square is also a parallelogram.
View SolutionGive reasons for the following:
(a) A square can be thought of as a special rectangle.
(b) A square can be thought of as a special rhombus.
( c) A rectangle can be thought of as a special parallelogram.
(d) A square is also a parallelogram.
View Solution
