Explain the meaining of each of the following terms:
(i)Variate (ii)Class interval (iii)Class size
(iv)Class mark (v)Class limit (vi)True class limits
(vii)Frequency of a class (viii)Cumulative frequency of a class
To explain the meanings of the given terms, we will break down each term step by step.
1. Variate:
- A variate is any characteristic or property that can take on different values. For example, height, weight, age, or test scores are all examples of variates because they can vary among individuals.
2. Class Interval:
- A class interval is a range of values that groups raw data into categories. For instance, if we have data on students' scores, we might group them into intervals like 0-10, 11-20, 21-30, etc. Each of these ranges is a class interval.
3. Class Size:
- Class size refers to the width of a class interval. It is calculated by subtracting the lower limit of the class interval from the upper limit. For example, in the class interval 10-20, the class size is 20 - 10 = 10.
4. Class Mark:
- The class mark is the midpoint of a class interval. It is calculated by taking the average of the upper and lower limits of the class interval. For example, for the class interval 10-20, the class mark is (10 + 20) / 2 = 15.
5. Class Limit:
- Class limits are the boundaries of a class interval. Each class interval has a lower limit and an upper limit. For example, in the class interval 10-20, 10 is the lower class limit and 20 is the upper class limit.
6. True Class Limits:
- True class limits are the actual boundaries of a class interval when considering the data's precision. They account for the fact that data may not be exact. For example, if the class interval is 10-20, the true class limits would be 9.5 (lower) and 20.5 (upper).
7. Frequency of a Class:
- The frequency of a class is the number of observations or data points that fall within that class interval. For example, if there are 5 students whose scores fall between 10 and 20, then the frequency of the class interval 10-20 is 5.
8. Cumulative Frequency of a Class:
- Cumulative frequency is the running total of frequencies up to a certain class interval. It is calculated by adding the frequency of the current class to the cumulative frequency of the previous class. For example, if the frequency of the class interval 10-20 is 5 and the frequency of the class interval 0-10 is 3, then the cumulative frequency for the class interval 10-20 would be 3 + 5 = 8.
- A variate is any characteristic or property that can take on different values. For example, height, weight, age, or test scores are all examples of variates because they can vary among individuals.
- A class interval is a range of values that groups raw data into categories. For instance, if we have data on students' scores, we might group them into intervals like 0-10, 11-20, 21-30, etc. Each of these ranges is a class interval.
- Class size refers to the width of a class interval. It is calculated by subtracting the lower limit of the class interval from the upper limit. For example, in the class interval 10-20, the class size is 20 - 10 = 10.
- The class mark is the midpoint of a class interval. It is calculated by taking the average of the upper and lower limits of the class interval. For example, for the class interval 10-20, the class mark is (10 + 20) / 2 = 15.
- Class limits are the boundaries of a class interval. Each class interval has a lower limit and an upper limit. For example, in the class interval 10-20, 10 is the lower class limit and 20 is the upper class limit.
- True class limits are the actual boundaries of a class interval when considering the data's precision. They account for the fact that data may not be exact. For example, if the class interval is 10-20, the true class limits would be 9.5 (lower) and 20.5 (upper).
- The frequency of a class is the number of observations or data points that fall within that class interval. For example, if there are 5 students whose scores fall between 10 and 20, then the frequency of the class interval 10-20 is 5.
- Cumulative frequency is the running total of frequencies up to a certain class interval. It is calculated by adding the frequency of the current class to the cumulative frequency of the previous class. For example, if the frequency of the class interval 10-20 is 5 and the frequency of the class interval 0-10 is 3, then the cumulative frequency for the class interval 10-20 would be 3 + 5 = 8.
Topper's Solved these Questions
- PRESENTATION OF DATA IN TABULAR FORMBOOK - RS AGGARWALCHAPTER - PRESENTATION OF DATA IN TABULAR FORMEXERCISE - EXERCISE 29
1 Videos
- PRESENTATION OF DATA IN TABULAR FORMBOOK - RS AGGARWALCHAPTER - PRESENTATION OF DATA IN TABULAR FORMEXERCISE - EXERCISE 30
1 Videos
- PRESENTATION OF DATA IN TABULAR FORMBOOK - RS AGGARWALCHAPTER - PRESENTATION OF DATA IN TABULAR FORMEXERCISE - Exercise 16
14 Videos
- POLYNOMIALSBOOK - RS AGGARWALCHAPTER - POLYNOMIALSEXERCISE - Multiple Choice Questions (Mcq)
32 Videos
- PROBABILITYBOOK - RS AGGARWALCHAPTER - PROBABILITYEXERCISE - Multiple Choice Questions (Mcq)
16 Videos
Similar Questions
Explain the meaning of the following terms: variate (ii) class-integral (iii) class-size class-mark (v) frequency class limites (vii) true class limits
View SolutionExplain the meaning of the following:
(i) Class-interval(ii) Class size (iii) Frequency (iv) Class limits (v) True class limits
View Solution
Knowledge Check
The mid-value of a class interval is 25 and the class size is 8. The class interval is
A37 - 45B21 - 29C36.5 - 44.5D36.5 - 46.5Class mark of a particular class is 6.5 and class size is 3, then class interval is:
A5-8B6.5−9.5C3.5−6.5D4.25Class mark of class interval 60–70 is
A60B70C65D72
Find the class marks and class size of the following distribution.

View SolutionUpper limit of class interval 75-85 is
View SolutionIn the class interval 20 - 30, the lower class limit is _______
View SolutionWrite the upper classs limit and the lower class limit of the class 35-40.
View SolutionDetermine the upper limit of the modal class of the following frequency distribution:
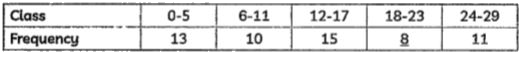
View Solution
