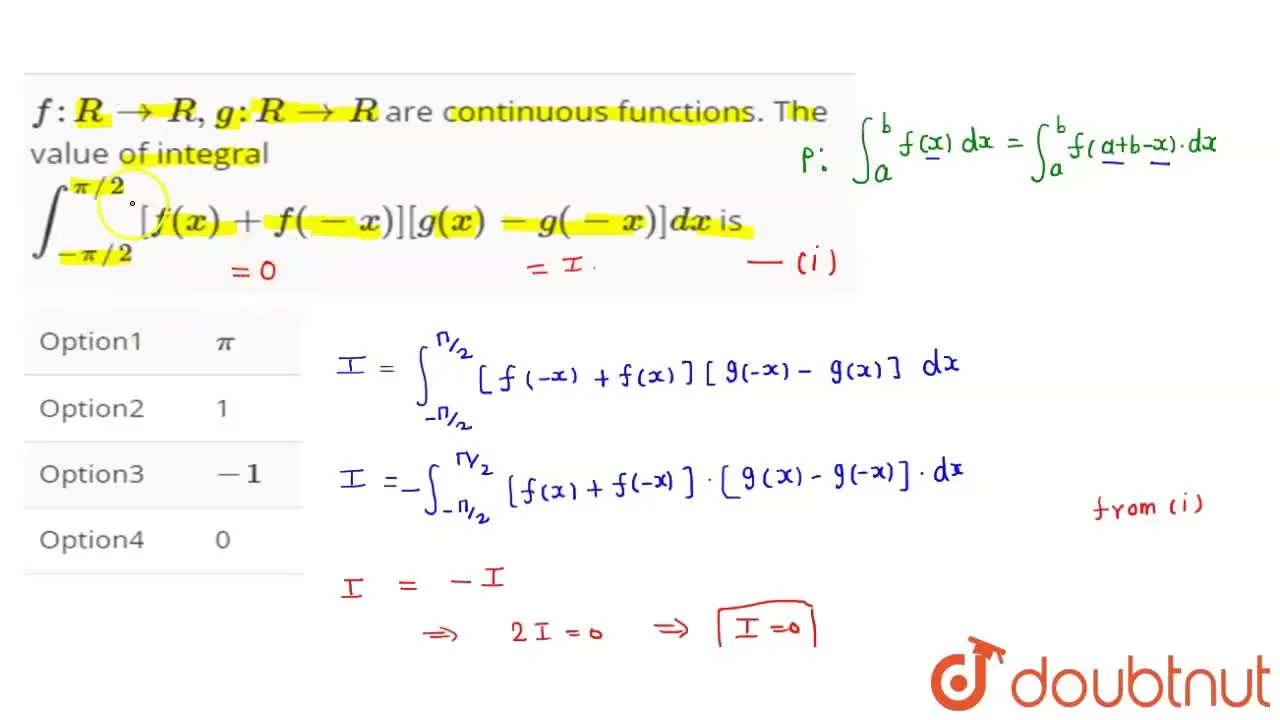
f:R→R,g:R→R are continuous functions. The value of integral ∫π/2−π/2[f(x)+f(−x)][g(x)−g(−x)]dx is
π
1
−1
0
The correct Answer is:D
Step by step video, text & image solution for f: R rarr R, g: R rarr R are continuous functions. The value of integral int_(-pi//2)^(pi//2) [f(x) + f(-x)] [g(x) -g(-x)] dx is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ML KHANNACHAPTER - DEFINITE INTEGRALEXERCISE - ProblemSet (3) True and False1videos
DEFINITE INTEGRAL
- BOOK - ML KHANNACHAPTER - DEFINITE INTEGRALEXERCISE - Problem Set (3) Fill in the blanks1videos
DEFINITE INTEGRAL
- BOOK - ML KHANNACHAPTER - DEFINITE INTEGRALEXERCISE - ProblemSet (2) Fill in the blanks1videos
DEFINITE INTEGRAL
- BOOK - ML KHANNACHAPTER - CORRELATION AND REGRESSION EXERCISE - SELF ASSESSMENT TEST10videos
CORRELATION AND REGRESSION
- BOOK - ML KHANNACHAPTER - DETERMINANTS EXERCISE - Self Assessment Test19videos
DETERMINANTS
Similar Questions
Let f:R→R and g:R→R be continuous functions. Then the value of the integral ∫π2π2[f(x)+f(−x)][g(x)−g(−x)]dx is
View SolutionLet f:R→andg:R→R be continuous function. Then the value of the integral ∫π2−π2[f(x)+f(−x)][g(x)−g(−x)]dxis π (b) 1 (c) −1 (d) 0
View SolutionIf f and g are two continuous function, then the value of ∫π/4−π/4{f(x)+f(−x)}{g(x)−g(−x)}dx is
View SolutionIf f:R→Randg:R→R are two given functions, then prove that 2min.{f(x)−g(x),0}=f(x)−g(x)−|g(x)−f(x)|
View SolutionLet f:R→R and g:R→R be two functions such that fog(x)=sinx2andgof(x)=sin2x Then, find f(x)andg(x).
View SolutionLet f:R→Randg:R→R be two functions such that (gof)(x)=sin2xand(fog)(x)=sin(x2). Find f(x)andg(x).
View Solutionf:R→R,f(x)=x3+3x+2 and g:R→R such that f(g(x))=x. Then value of ∫62g(x)dx is
View SolutionLet f:R→R and g:R→R be two functions such that fog (x) =sinx2 and gof (x) = sin2x. Then , find f(x) and g(x).
View SolutionLet f:R→Rbe a continuous function given by f(x+y)=f(x)+f(y) for all x,y,∈R, if ∫20f(x)dx=α, then ∫2−2f(x)dx is equal to
View SolutionLet f: R→R be a continuous function. Then limx→π/4π4∫sec2x2f(x)dxx2−π216 is equal to :
View Solution