Find A+BifA=[2536]andB=[7965]Solution in Punjabi
The correct Answer is:(A)→(p,r);(B)→(s);(C)→(q);(D)→(s)
(A)→(p,r),(B)→(s),(C)→(q),(D)→(s)
On comparing, we get
{4f(−1)−3}a2+{4f(1)−3}a+f(2)=0
{4f(−1)−3}b2+{4f(1)−3}b+f(2)=0
and {4f(−1)−3}c2+{4f(1)−3}C+f(2)=0
It is clear that a, b, c are the roots of
{4f(−1)−3}x2+{4f(1)−3}x+f(2)=0 then
4f(−1)−3=0,4f(1)−3=0,f(2)=0
⇒f(−1)=34,f(1)=34,f(2)=0 Let f(x)=(x−2)(ax+b)
Now, f(−1)=34⇒(−3)(−a+b)=34⇒a−b=14
f(1)=34⇒(−1)(−a+b)=34⇒a+b=−34
∴a=−14,b=−12
⇒f(x)=14(4−x2)
Graph of y=f(x)
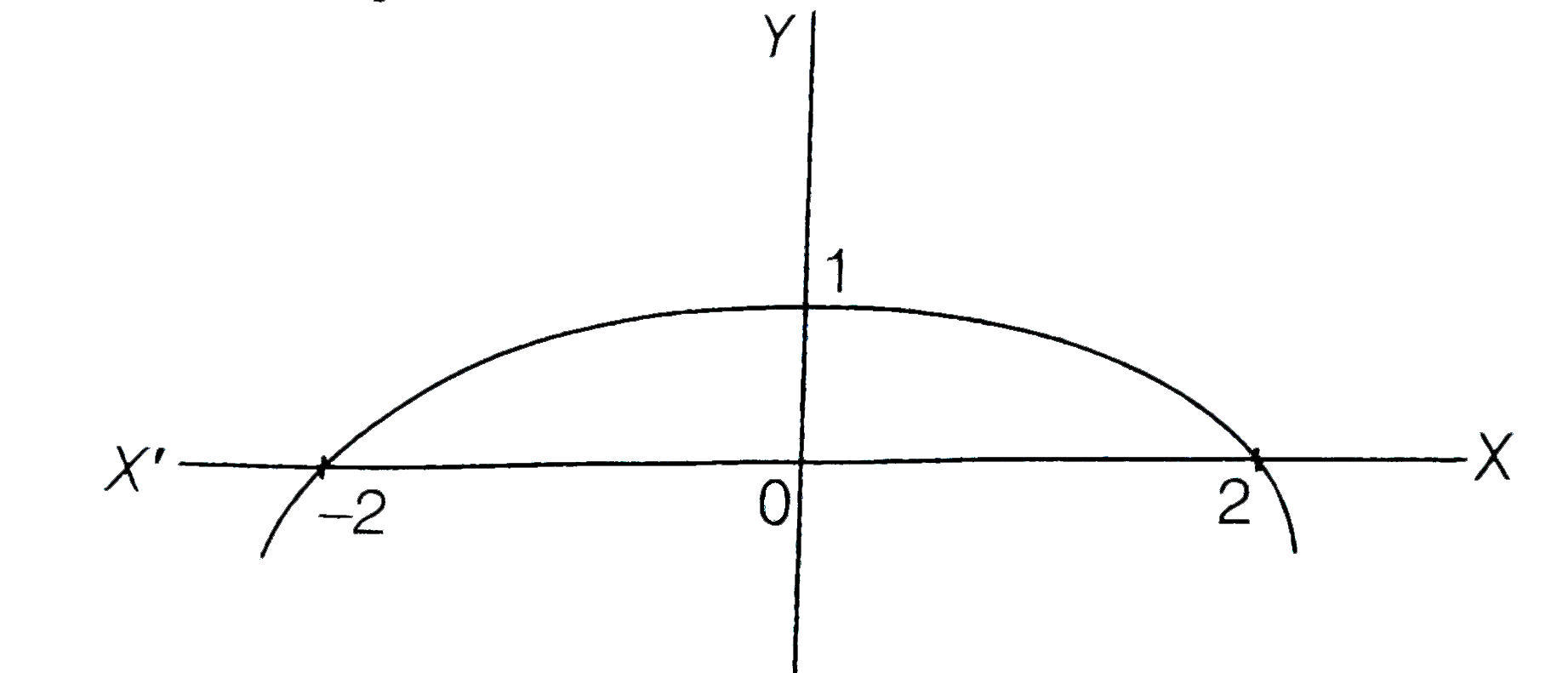
(A) x- coordinates of the point intersection of y=f(x) with
ther X- axis are - 2 and 2.
(B) Area =32∫2−214(4−x2)dx=34∫20(4−x2)dx
=34[4x−x33]20=34×163=4
(C) Maximum value of f(x) is 1.
(D) Length of intercept on the X_ axis is 4.
Topper's Solved these Questions
- MATRICES BOOK - ARIHANT MATHSCHAPTER - MATRICES EXERCISE - Exercise (Statement I And Ii Type Questions)
10 Videos
- MATRICES BOOK - ARIHANT MATHSCHAPTER - MATRICES EXERCISE - Exercise (Subjective Type Questions)
14 Videos
- MATRICES BOOK - ARIHANT MATHSCHAPTER - MATRICES EXERCISE - Exercise (Single Integer Answer Type Questions)
10 Videos
- MATHEMATICAL INDUCTION BOOK - ARIHANT MATHSCHAPTER - MATHEMATICAL INDUCTION EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
2 Videos
- MONOTONICITY MAXIMA AND MINIMABOOK - ARIHANT MATHSCHAPTER - MONOTONICITY MAXIMA AND MINIMAEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
31 Videos
Similar Questions
(Statement1 Assertion and Statement- 2 (Reason)
Each of these questions also has four alternative
choices, only one of which is the correct answer. You
have to select the correct choice as given below.
Statement - 1 If matrix A=[aij]3×3,B=[bij]3×3, where aij+aji=0andbij−bji=0 then A4B5 is non-singular
matrix.
Statement-2 If A is non-singular matrix, then |A|≠0.
View SolutionIf S is a real skew-symmetric matrix, then prove that I−S is non-singular and the matrix A=(I+S)(I−S)−1 is orthogonal.
View SolutionLet A,B,C,D be (not necessarily ) real matrices such that AT=BCD,BT=CDA,CT=DABandDT=ABC for the matrix S=ABCD the least value of k such that Sk=S is
View SolutionIf t₁+t₅+t15+t₁₀+t₂₀+t₂₄=225 Find the sum of first 24th term of that A.P?
View SolutionThe least value of α∈R for which 4ax2+1x≥1, for all x>0, is
View Solutionयदि A=[3725] और B=[6879] है तो सत्यापित कीजिए कि (AB)−1=B−1A−1 है।
View SolutionVerify (AB)−1=B−1A−1 for the matrices A and B where
A=[3725] and B=[6879]
View SolutionVerify (AB)−1=B−1A−1 for the matrices A and B where
A=[4165] and B=[2516]
View SolutionLet A= [3725] and B = [6879] .Verify that (AB)−1=B−1A−1
View SolutionLet A= [3725] and B = [6879] .Verify that (AB)−1=B−1A−1
View SolutionLet A= [3725] and B = [6879] .Verify that (AB)−1=B−1A−1
View SolutionLet A= [3725] and B = [6879] .Verify that (AB)−1=B−1A−1
View SolutionIf A=[3725],B=[6879] then verify (AB)−1=B−1A−1.
View SolutionIf A = [[3,7],[2,5]] ਅਤੇ B = [[6,8],[7,9]] Then check that (AB)^-1 = B^-1A^-1 Is
View SolutionLet A=[3725] and B=[6879] Verify that (AB)−1=B−1A−1
View SolutionLet A =[3725] and B=[6879]Verify that (AB)−1=B−1A−1
View Solution
