Find angle θ, 0 < θ < π2 , which increase twice as fast as sine
To solve the problem of finding the angle θ (where 0<θ<π2) that increases twice as fast as sine, we can follow these steps:
Step 1: Set up the equation
We know that θ increases twice as fast as sin(θ). This can be expressed mathematically as:
dθdt=2ddt(sin(θ))
Step 2: Differentiate sin(θ)
Using the chain rule, we differentiate sin(θ):
ddt(sin(θ))=cos(θ)dθdt
Substituting this into our equation from Step 1 gives:
dθdt=2cos(θ)dθdt
Step 3: Simplify the equation
Now we can simplify the equation. We can cancel dθdt from both sides, assuming dθdt≠0:
1=2cos(θ)
Step 4: Solve for cos(θ)
From the simplified equation, we can solve for cos(θ):
cos(θ)=12
Step 5: Find θ
Now, we need to find the angle θ that satisfies cos(θ)=12. The angle in the range 0<θ<π2 that satisfies this condition is:
θ=π3
Conclusion
Thus, the required angle θ is:
θ=π3
We know that θ increases twice as fast as sin(θ). This can be expressed mathematically as:
dθdt=2ddt(sin(θ))
Using the chain rule, we differentiate sin(θ):
ddt(sin(θ))=cos(θ)dθdt
Substituting this into our equation from Step 1 gives:
dθdt=2cos(θ)dθdt
Now we can simplify the equation. We can cancel dθdt from both sides, assuming dθdt≠0:
1=2cos(θ)
From the simplified equation, we can solve for cos(θ):
cos(θ)=12
Now, we need to find the angle θ that satisfies cos(θ)=12. The angle in the range 0<θ<π2 that satisfies this condition is:
θ=π3
Thus, the required angle θ is:
θ=π3
Topper's Solved these Questions
- APPLICATION OF DERIVATIVESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Long answer types questions
10 Videos
- APPLICATION OF DERIVATIVESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - OBJECTIVE TYPES QUESTIONS
25 Videos
- APPLICATION OF INTEGRALSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF INTEGRALSEXERCISE - Objective Type Questions
22 Videos
Similar Questions
Find an angle θ,0<θ<π2, which increases twice as fast as its sine.
View SolutionA body is thrown at an angle θ0 with the horizontal such that it attains a speed equal to √23 times the speed of projection when the body is at half of its maximum height. Find the angle θ0.
View SolutionFind an angle θ , which increases twice as fast as its cosine.
View SolutionFind an angle θ , which increases twice as fast as it sine.
View SolutionFind an angle θ, which increases twice as fast as its sine.
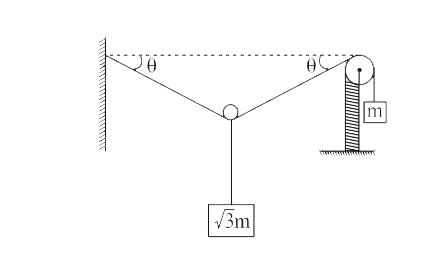
View SolutionThe system is in equilibrium. Find the angle θ
View SolutionIf 4sin2θ−1=0 and angle θ is less than 90∘, find the value of θ and hence the value of cos2θ+tan2θ.
View SolutionIf 4sin2θ−1=0 and angle θ is less than 90∘, find the value of θ and hence the value of cos2θ+tan2θ.
View SolutionFind the angle θ whose cosine is equal to its tangent.
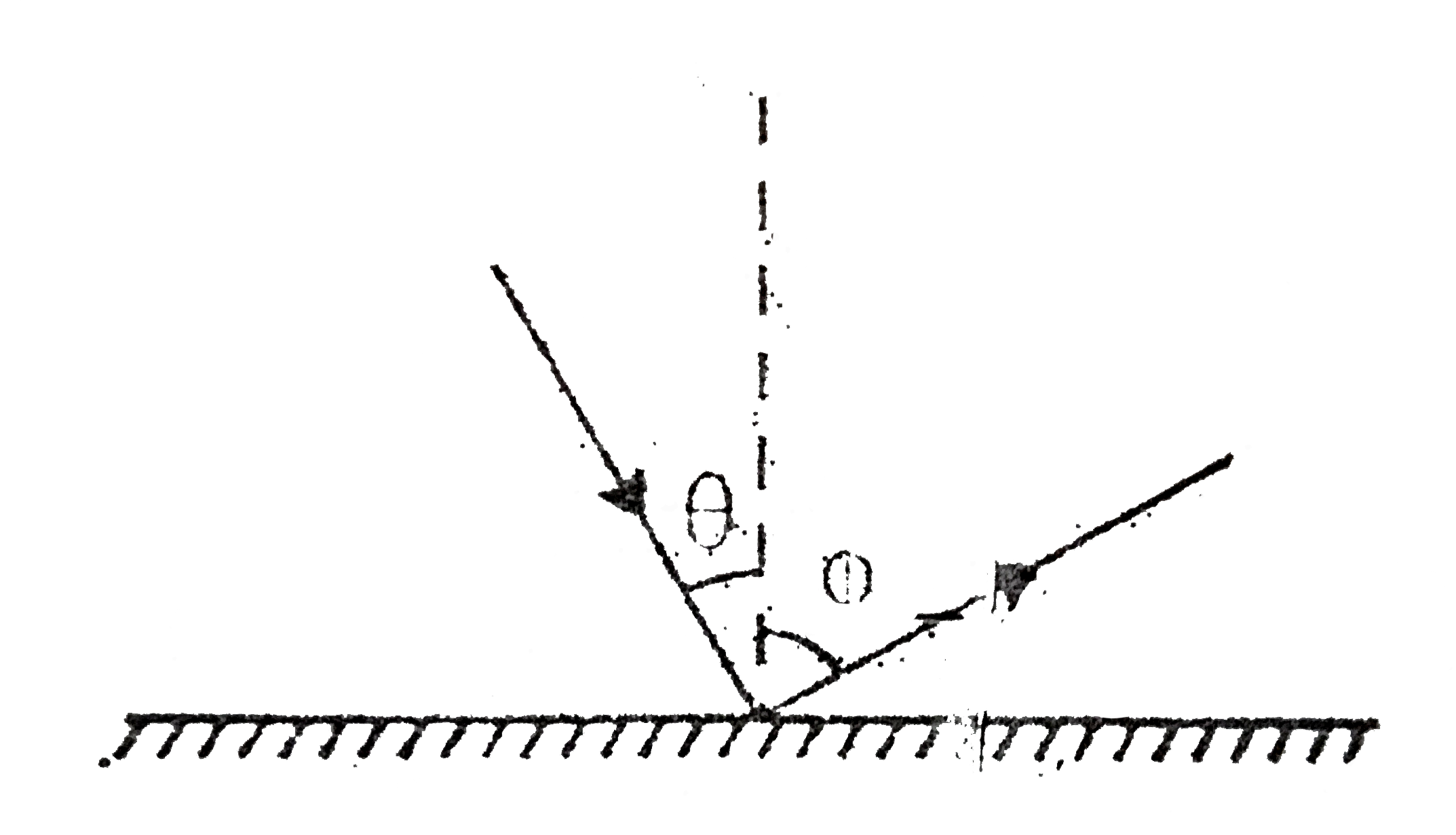
View SolutionA particle strikes a smooth horizontal surface at an angle θ>0 and it rebounds at an angle ϕ as shown
View Solution
