Find area parallelogram lines y=mx,y=mx+1,y=nxandy=nx+1 equal to:
To find the area of the parallelogram formed by the lines y=mx, y=mx+1, y=nx, and y=nx+1, we can follow these steps:
Step 1: Identify the lines and their intersections
We have the following lines:
1. y=mx
2. y=mx+1
3. y=nx
4. y=nx+1
Step 2: Find the coordinates of the intersection points
To find the vertices of the parallelogram, we need to determine the intersection points of these lines.
1. Intersection of y=mx and y=nx+1:
mx=nx+1⟹(m−n)x=1⟹x=1m−n
Substituting x into y=mx:
y=m(1m−n)=mm−n
So, the intersection point is:
A(1m−n,mm−n)
2. Intersection of y=mx+1 and y=nx:
mx+1=nx⟹(m−n)x=−1⟹x=−1m−n
Substituting x into y=nx:
y=n(−1m−n)=−nm−n
So, the intersection point is:
B(−1m−n,−nm−n)
3. Intersection of y=nx+1 and y=mx:
nx+1=mx⟹(n−m)x=−1⟹x=−1n−m
Substituting x into y=mx:
y=m(−1n−m)=−mn−m
So, the intersection point is:
C(−1n−m,−mn−m)
4. Intersection of y=nx+1 and y=mx+1:
nx+1=mx+1⟹(n−m)x=0⟹x=0
Substituting x=0 into either equation gives:
y=1
So, the intersection point is:
D(0,1)
Step 3: Calculate the area of the parallelogram
The area of the parallelogram can be calculated using the formula:
Area=Base×Height
Here, we can take the base as the distance between the lines y=mx and y=mx+1, which is 1, and the height as the horizontal distance between the lines y=mx and y=nx.
The height can be calculated as:
Height=∣∣∣1m−n∣∣∣
Thus, the area of the parallelogram is:
Area=Base×Height=1×∣∣∣1m−n∣∣∣=1|m−n|
Final Answer
The area of the parallelogram formed by the lines is:
Area=1|m−n|
We have the following lines:
1. y=mx
2. y=mx+1
3. y=nx
4. y=nx+1
To find the vertices of the parallelogram, we need to determine the intersection points of these lines.
mx=nx+1⟹(m−n)x=1⟹x=1m−n
Substituting x into y=mx:
y=m(1m−n)=mm−n
So, the intersection point is:
A(1m−n,mm−n)
mx+1=nx⟹(m−n)x=−1⟹x=−1m−n
Substituting x into y=nx:
y=n(−1m−n)=−nm−n
So, the intersection point is:
B(−1m−n,−nm−n)
nx+1=mx⟹(n−m)x=−1⟹x=−1n−m
Substituting x into y=mx:
y=m(−1n−m)=−mn−m
So, the intersection point is:
C(−1n−m,−mn−m)
nx+1=mx+1⟹(n−m)x=0⟹x=0
Substituting x=0 into either equation gives:
y=1
So, the intersection point is:
D(0,1)
The area of the parallelogram can be calculated using the formula:
Area=Base×Height
Here, we can take the base as the distance between the lines y=mx and y=mx+1, which is 1, and the height as the horizontal distance between the lines y=mx and y=nx.
Height=∣∣∣1m−n∣∣∣
Area=Base×Height=1×∣∣∣1m−n∣∣∣=1|m−n|
The area of the parallelogram formed by the lines is:
Area=1|m−n|
Similar Questions
Find the value of m so that lines y=x+1,2x+y=16andy=mx−4 may be concurrent.
View SolutionIf the lines represented by 2x2−5xy+2y2=0 be the sides of a parallelogram and the line 5x+2y=1 be one of its diagonal. Find the equation of the other diagonal, and area of the parallelogram .
View SolutionFind the area bounded by the parabola x2=y and line y=1.
View SolutionFor what value of m are the three lines y=x+1,y=2(x+1)andy=mx+3 concurrent ?
View SolutionLet m be a positive integer and let the lines 13x+11y=700 and y=mx−1 intersect in a point whose coordinates are integer. Then m equals to :
View SolutionFind the perpendicular distance between the lines
y=mx+c,y=mx+d
View Solution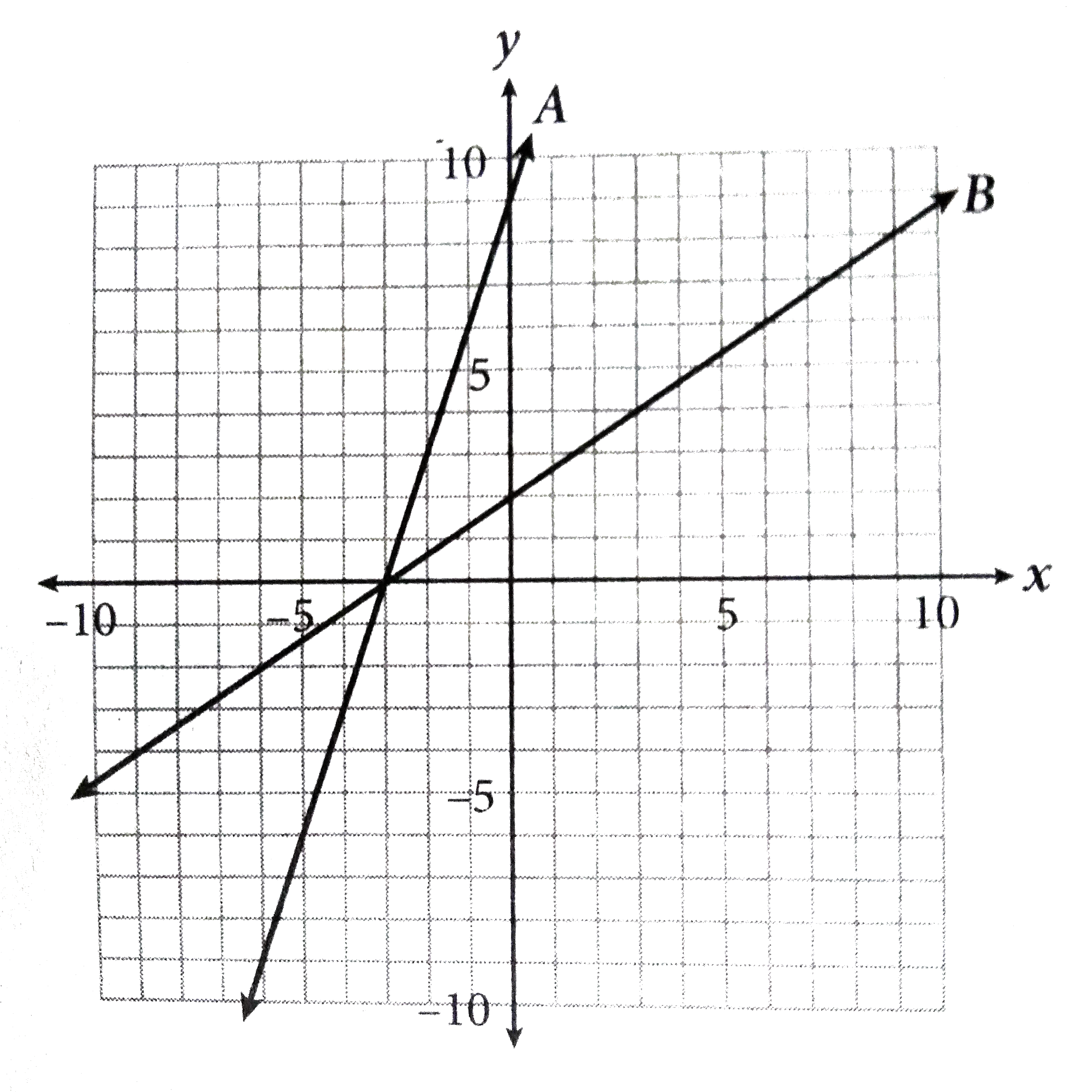
If the equation of line A shown on the graph is given by y=mx+b, and the equation of line B is given by y=k(mx+b), what is the value of k?
View SolutionFind the value of m, for which the line y=mx+25√33 is a normal to the conic x216−y29=1.
View SolutionThe distance between the lines y=mx+c1 and y=mx+c2 is
View SolutionFor positive l, m and n, if the points x=ny+mz,y=lz+nx,z=mx+ly intersect in a straight line, when
Q. l, m and n satisfy the equation
View Solution
