Find ∫logxdx
To find the integral of logxdx, we will use the method of integration by parts. The formula for integration by parts is:
∫udv=uv−∫vdu
Step 1: Choose u and dv
Let:
- u=logx (which we will differentiate)
- dv=dx (which we will integrate)
Step 2: Differentiate u and Integrate dv
Now, we need to find du and v:
- Differentiate u:
du=1xdx
- Integrate dv:
v=∫dx=x
Step 3: Apply the Integration by Parts Formula
Now, we can apply the integration by parts formula:
∫logxdx=uv−∫vdu
Substituting the values we found:
∫logxdx=xlogx−∫x⋅1xdx
Step 4: Simplify the Integral
The integral simplifies as follows:
∫logxdx=xlogx−∫1dx
Step 5: Compute the Remaining Integral
Now, compute the remaining integral:
∫1dx=x
So, we have:
∫logxdx=xlogx−x
Step 6: Add the Constant of Integration
Finally, we add the constant of integration C:
∫logxdx=xlogx−x+C
Final Answer
Thus, the integral of logxdx is:
∫logxdx=xlogx−x+C
---
- u=logx (which we will differentiate)
- dv=dx (which we will integrate)
- Differentiate u:
du=1xdx
- Integrate dv:
v=∫dx=x
∫logxdx=uv−∫vdu
Substituting the values we found:
∫logxdx=xlogx−∫x⋅1xdx
∫logxdx=xlogx−∫1dx
∫1dx=x
So, we have:
∫logxdx=xlogx−x
∫logxdx=xlogx−x+C
∫logxdx=xlogx−x+C
Topper's Solved these Questions
- INTEGRALSBOOK - NCERT ENGLISHCHAPTER - INTEGRALSEXERCISE - EXERCISE 7.1
22 Videos
- INTEGRALSBOOK - NCERT ENGLISHCHAPTER - INTEGRALSEXERCISE - EXERCISE 7.8
6 Videos
- INTEGRALSBOOK - NCERT ENGLISHCHAPTER - INTEGRALSEXERCISE - EXERCISE 7.3
24 Videos
- DIFFERENTIAL EQUATIONSBOOK - NCERT ENGLISHCHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - EXERCISE 9.1
12 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBOOK - NCERT ENGLISHCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Solved Examples
13 Videos
Similar Questions
Knowledge Check
Find x
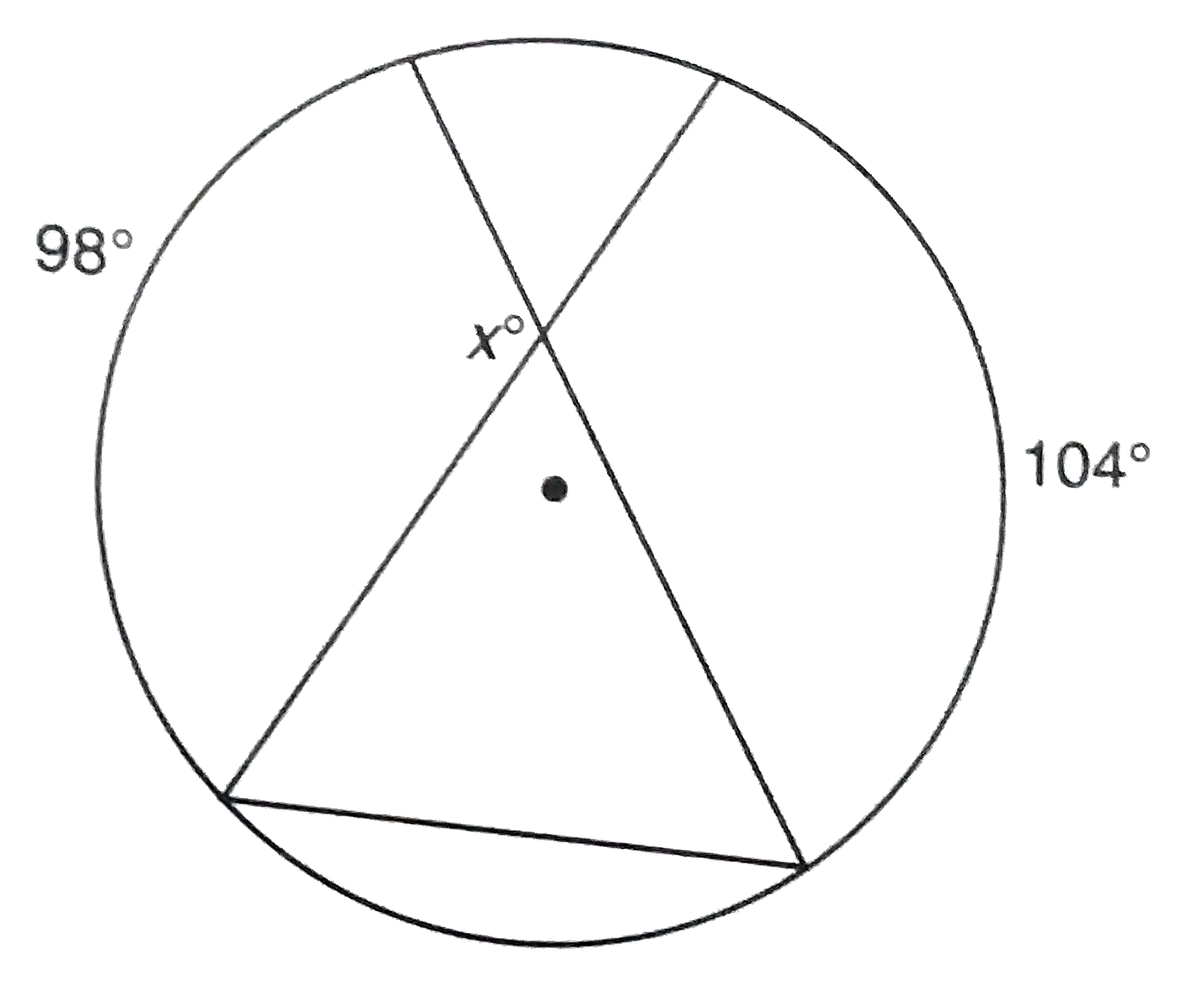 A49∘B52∘C79∘D101∘
A49∘B52∘C79∘D101∘Find x.
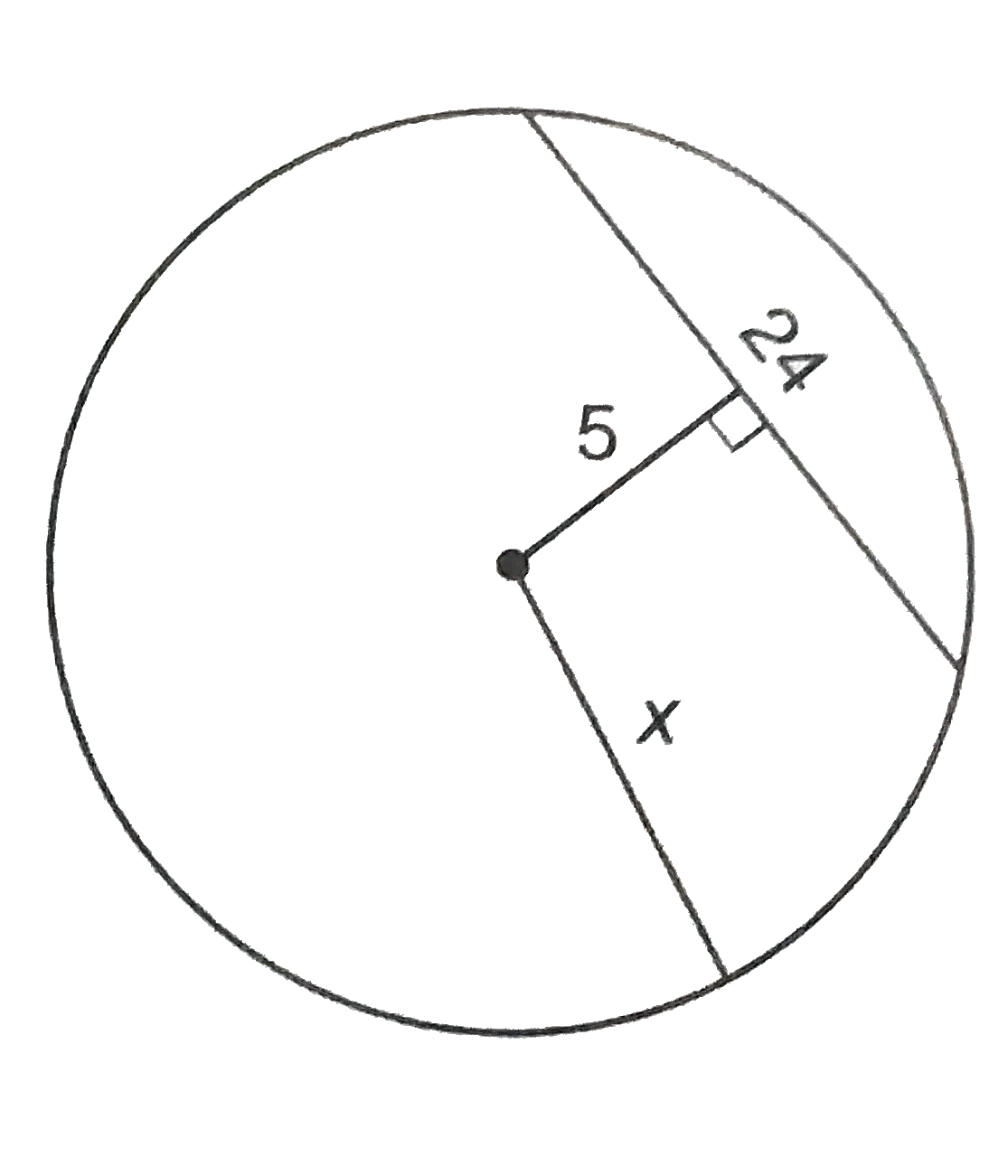 A7B11C12D13
A7B11C12D13Find x.
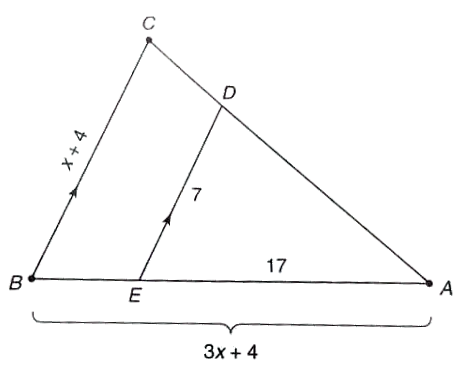 A2.16B3C4.33D10
A2.16B3C4.33D10