Find the area of the shaded design in Fig. 12.17, where ABCD is a square of side 10 cm and semicircles are drawn with each side of the square asdiameter.
If we look closely at the given figure, we can see,
Area of 4 semi circles = Area of shaded region + Area of square ABCD
So, Area of shaded region,AS = Area of 4 semi circles - Area of square ABCD
Here, Radius of circle, r=CD2=102=5cm
Area of semi-circle, =12πr2
So, area of 4 semi-circles, ASC=4⋅12πr2=2πr2=2∗3.14∗5∗5
ASC=157cm2
Now, Area of square, ASQ=CD2=100cm2
So, Area of shaded region, AS=157−100=57cm2
Similar Questions
Find the area of the shaded design in Fig. 12.17, where ABCD is a square of side 10 cm and semicircles are drawn with each side of the square asdiameter.
View SolutionFind the area of the shaded region in Fig. 12.16, where ABCD is a square of side 14 cm.
View SolutionFind the area of the shaded region in Fig where ABCD is a square of side 14 cm.
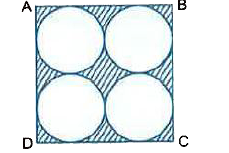
View SolutionFind the area of the shaded region in figure where ABCD is a square of side 14 cm.
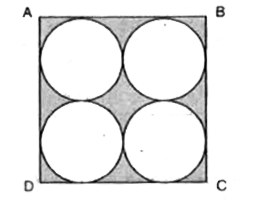
View SolutionFind the area of the shaded region as shown in figure, where ABCD is square of side 14 cm.
View SolutionFind the area of the shaded region in Fig. 12.21, if ABCD is a square of side 14 cm and APD and BPC are semicircles.
View SolutionABCD is a square of 10 cm and semicircles are drawn with each side of the square as diameter. Find the area of the shaded region. (π=3.14)
View SolutionFind the area of the shaded region in Fig. 15.48, where ABCD is a square of side 10 cm. (Use π=3.14) (FIGURE)
View SolutionIn the figure, ABCD is a square of side 14 cm. Semi-circles are drawn with each side of square as diameter. Find the area of the shaded region.
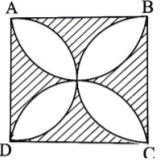
View SolutionFind the area of the shaded region in figure, if ABCD is a square of side 7 cm. and APD and BPC are semi-circles
View Solution
