Question

Find the areas of the region $\{x,y\}:y^2\le 4x,4x^2+4y^2\le 9\},$
using integration.

Explanation

Clearly , we have to find the area enclosed between the curves ` y^2 =4x and 4x^2+4y^2=9`
The curve `y^2=4x y^2 =9`
The curve `y^2=4x` is a right-handed parabola with its vertex at (0.0) and radius equal to (3/2) units .

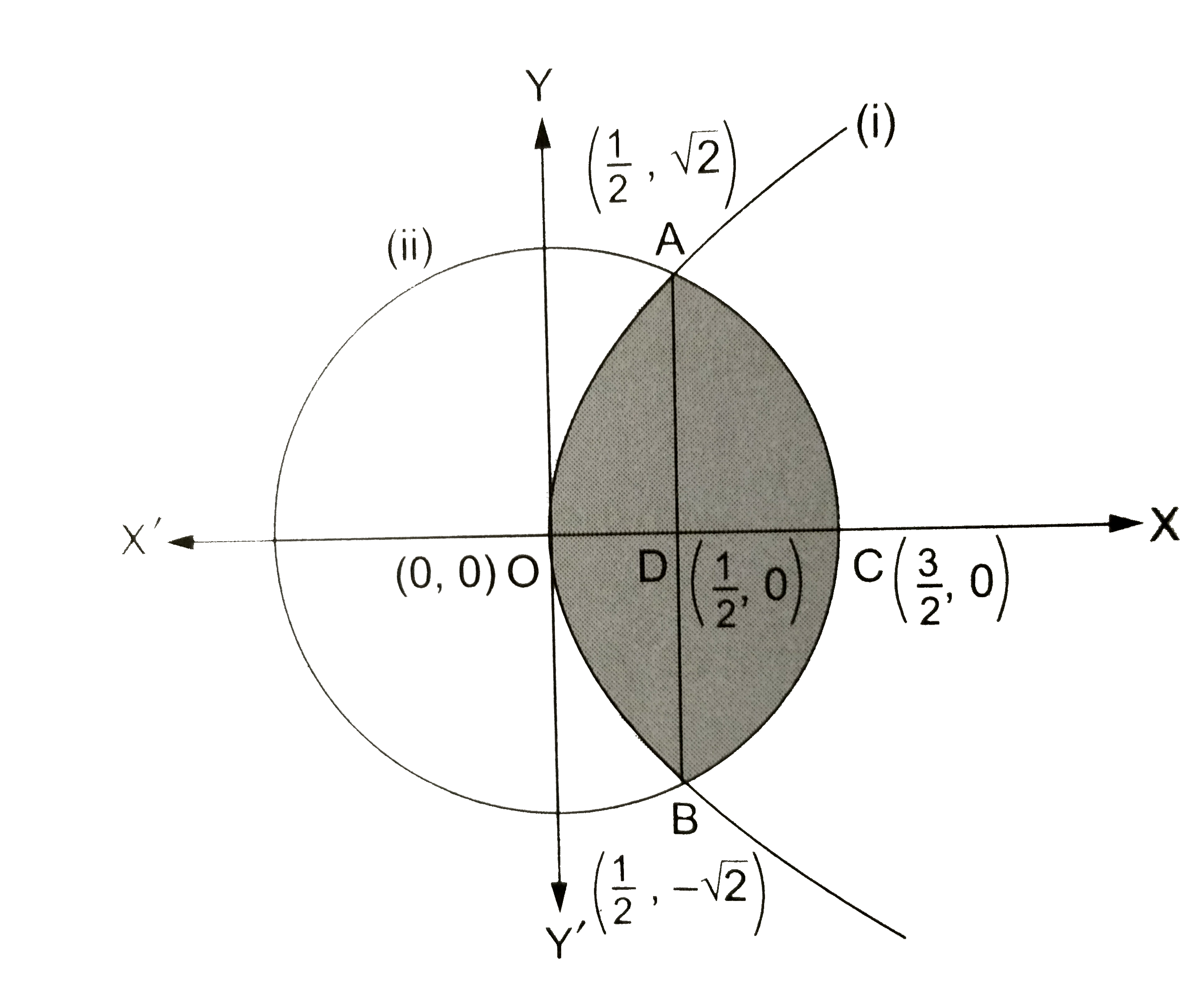
The shaded region shows the required area .
Now `y^2=4x`
and `4x^2+4y^9=9`
Putting the value of `y^2` from (i) into (ii) , we get
`4x^2+16x-9=0`
`rArrr 4x^2+18x-2x-9=0`
`2x(2x+9)-(2x-9)=0`
`rArr x= -9/2 `in (i) we get
`y^2=-18 rArr y` in imaginary
Putting `x=1/2` in (i) we get `y^2 =2 rArr y= pm sqrt(2)`
So, the given curves interset at the point `A(1/2,sqrt(2))` and B`(1/2,-sqrt(2))`
Since the equation of each of the given cuves contains only even powers of y, each curve is symmetrical about the x- axis.
Required ara =2(area ODCAO)
2(area ODAO+area DCAD)
`=2{underset(0)overset(1//2)int `(y of the parabola) dx + `underset(1//2)overset(3//2)int` (y of the circle )dx }
`=2{underset(0)overset(1//2)int2sqrt(x)dx+underset(1//2)overset(3//2)intsqrt(9/4-x^2)dx}`
`=2{[4/3x^(3//2)]_0^(1/2)+[(xsqrt(9/4-x^2))/(2)+9/8sin^(-1)(x/(3//2))]_(1//2)^(3//2)}`
`=4/(3sqrt2)+(9/4sin^(-1)1)-((sqrt(2))/(2)+9/8sin^(-1)""1/3)`
`{(sqrt(2))/6+9/4(pi/2-sin^(-1)""1/3)}`sq units
`={(sqrt(2))/(6)+9/3cos^(-1)(1/3)}`sq units.
Hence , the required area is `={(sqrt(2))/(6)+9/4cos^(-1)(1/3)}`sq uni