Find the co-ordinates of the points which divide the line segment joining A (-2, 2) and B(2, 8) into four equal parts.
Let P, Q and R be the poins on line segment AB such that
AP=PQ=QR=RB
Let AP=PQ=QR=RB=k
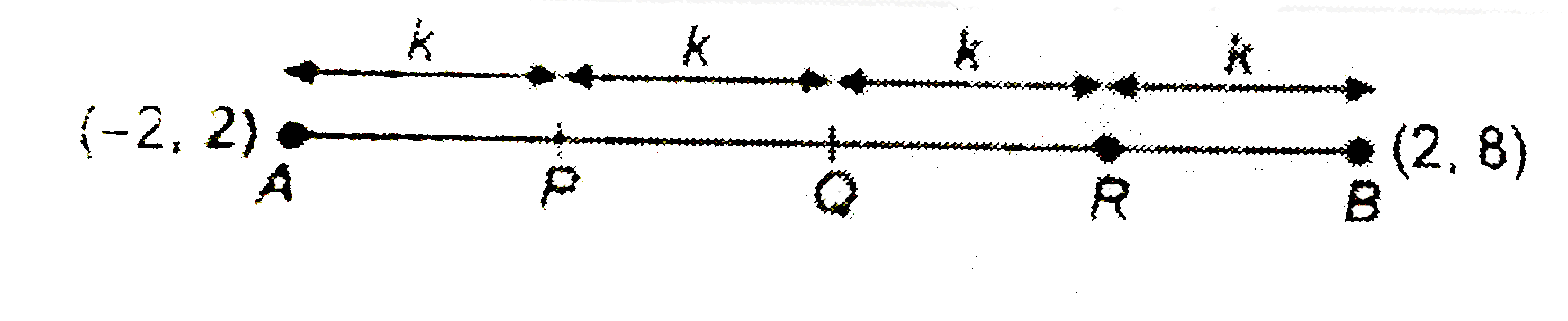
Now, APPB=k3k=13
Therefore, P divides AB internally in the ration 1 : 3.
∵ Internally ratio=(m1x2+m2x1m1+m2,m1y2+m2y1m1+m2)
P=(1×2+3(−2)1+3,1×8+3×21+3)=(2−64,8+64)=(−44,144)=(−1,72)
Again, ARRB=3kk=31
Therefore, R divides AB internally in the ratio 3 : 1.
∴ R=(3×2+1×(−2)3+1,3×8+1×23+1)
=(6−24,24+24)=(44,264)=(1,132)
Also, AQQB=2k2k=11
∴ Q is the mid-point of AB.
∴ Q=(−2+22,2+82)[∵mid-point=(x1+x22,y1+y22)]
i.e., Q=(02,102)=(0,5)
So, required points are (−1,72),(0,5)and(1,132).
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHANCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Exercise 7a20videos
CO-ORDINATE GEOMETRY
- BOOK - NAGEEN PRAKASHANCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Exercise 7b18videos
CO-ORDINATE GEOMETRY
- BOOK - NAGEEN PRAKASHANCHAPTER - CO-ORDINATE GEOMETRYEXERCISE - Miscellaneous Examples8videos
CO-ORDINATE GEOMETRY
- BOOK - NAGEEN PRAKASHANCHAPTER - CIRCLESEXERCISE - Revision Exercise Long Answer Questions3videos
CIRCLES
- BOOK - NAGEEN PRAKASHANCHAPTER - CONSTRUCTIONSEXERCISE - PROBLEMS FROM NCERT/ EXEMPLAR10videos
CONSTRUCTIONS
Similar Questions
Find the coordinates of the points which divide the line segment joining A(2,2)andB(2,8) into four equal parts.
View SolutionFind the coordinates of the points which divide the line segment joining A(−2,2)andB(2,8) into four equal parts.
View SolutionFind the coordinates of the points which divide the line segment joining A(-2,2) and (2,8) into four equal parts.
View SolutionFind the coordinates of points which divide the line segment joining A (5, - 6) and B (-1, 8) into four equal parts.
View SolutionFind the points which divide the line segment joining (8,12) and (12,8 ) into four equal parts.
View SolutionSolve the following questions. (Any two )
(ii) Find the co-ordinates of the points which divides the segment joining P(-6,10) and Q(-10,-2) into four equal parts .View SolutionFind the coordinates of the points which divide the line segment joining the points (−4, 0) and (0, 6) in four equal parts.
View SolutionFind the coordinates of the point which divides the line segment joining A(-2, 2) and B(2, 8) in the ratio 3 : 1.
View SolutionFind the coordinates of the points which divide the line segment joining the points (−4, 0) and (0, 6) in four equal parts.
View SolutionCalculate the co-ordinates of the point P which divides the line segment joining:
A (-4, 6) and B (3, -5) in the ratio 3:2.View Solution
