Find the equation of the circle with radius 5 whose centre lies on xaxis and passes through the point (2, 3).
It is given that the centre of the cricle lies on the x - axis.
So let C(k,0) be the centre of the circle.
Also, it is given that it passes through the point P(2,3).
∴ radius of the circle = |CP|
=√(k−2)2+(0−3)2
=√k2−4k+13
⇒√k2−4k+13=5 [∵ radius = 5 units ]
⇒k2−4k+13=25⇒k2−4k−12=0
⇒(k−6)(k+2)=0⇒k=6ork=−2
∴ centre of the circles is (6,0)or(−2,0).
Hence, the required equation of the circle is
(x−6)2+(y−0)2=52or(x+2)2+(y−0)2=52
⇒x2+y2−12x+11=0orx2+y2+4x−21=0
Thus, there are two circles satisfying the given conditions.
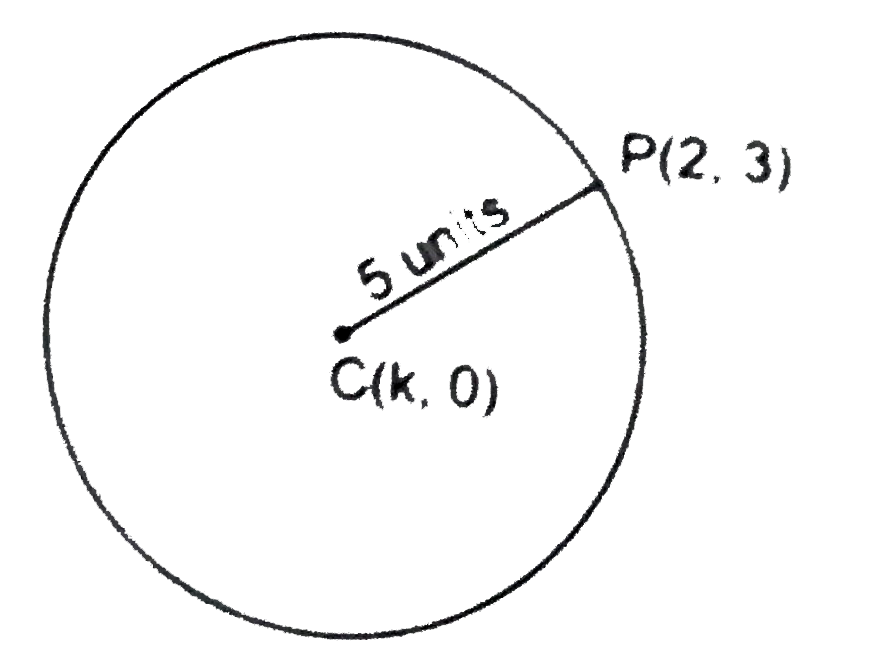
Topper's Solved these Questions
- BOOK - RS AGGARWALCHAPTER - CIRCLE EXERCISE - EXERCISE 21 A14videos
CIRCLE
- BOOK - RS AGGARWALCHAPTER - CIRCLE EXERCISE - EXERCISE 21 B18videos
CIRCLE
- BOOK - RS AGGARWALCHAPTER - BINOMIAL THEOREMEXERCISE - EXERCISE 10B16videos
BINOMIAL THEOREM
- BOOK - RS AGGARWALCHAPTER - COMBINATIONSEXERCISE - Exercise 9D18videos
COMBINATIONS
Similar Questions
Knowledge Check
- Question 1 - Select One
The equation of the circle with radius √5 units whose centre lies. On y-axis and passes through the point (2, 3) can be
Ax2+(y−4)2=5Bx2+(y+4)2=5C(x−4)2+y2=5D(x+4)2+y2=5
Find the equation of a circle of radius 5 whose centre lies on x-axis and passes through the point (2,3).
View SolutionFind the equation of the circle of radius 5 whose centre lies on y-axis and passes through (3,2).
View SolutionFind the equation of the circle of radius 5 whose centre lies on y-axis and which passes through the point (3, 2).
View SolutionThe number of equation of the circle with radius 5 whose centre lies on X-axis and passes through the point (2,3) is
View SolutionThe equation of the circle whose centre lies on the x-axis and which passes through the points (0,1),(1,1) is
View SolutionFind the equation of the circle whose centre lies on the line x−4y=1 and which passes through the points (3,7)and(5,5).
View SolutionFind the equation of the circle whose centre is (2,−5) and which passes through the point (3,2)
View Solution

