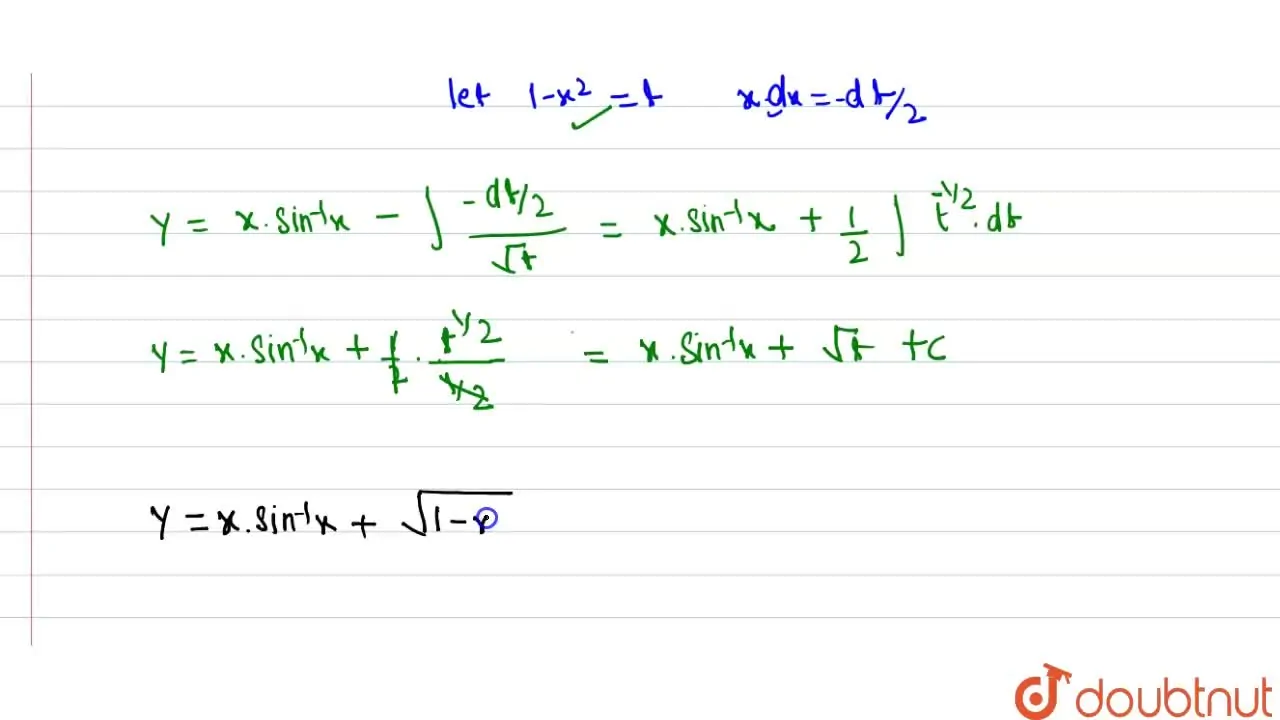Find the general solution of the differential equation
dydx=sin−1x.
We have ,
dydx=sin−1x
⇒dy=sin−1xdx [separating the variables]
⇒∫dy=∫sin−1xdx [integrating both sides]
⇒y=∫{(sin−1xI)⋅1II}dx+C, where C is an arbitrary constant
=(sin−1x)⋅x−∫1√1−x2⋅xdx+C [integrating by parts]
=(sin−1x)⋅x+12∫−2x√1−x2dx+C
=(sin−1x)⋅x+12∫1√tdt+C, where (1−x2)=t
=(sin−1x)⋅x+12×2√t+C
=(sin−1x)x+√1−x2+C.
Hence, y=(sin−1x)x+√1−x2+C is the required solution.
Hence, y=x(sin−1x)+√1−x2+C is the required solution of the given differential equation.
Related Playlists
- BOOK - RS AGGARWALCHAPTER - DIFFERENTIAL EQUATIONS WITH VARIABLE SEPARABLEEXERCISE - Exercise 19A16videos
DIFFERENTIAL EQUATIONS WITH VARIABLE SEPARABLE
- BOOK - RS AGGARWALCHAPTER - DIFFERENTIAL EQUATIONS WITH VARIABLE SEPARABLEEXERCISE - Exercise 19B60videos
DIFFERENTIAL EQUATIONS WITH VARIABLE SEPARABLE
- BOOK - RS AGGARWALCHAPTER - DIFFERENTIAL EQUATIONS AND THEIR FORMATION EXERCISE - Exercise 18C16videos
DIFFERENTIAL EQUATIONS AND THEIR FORMATION
- BOOK - RS AGGARWALCHAPTER - DIFFERENTIATIONEXERCISE - Exercise 10I51videos
DIFFERENTIATION
Similar Questions
Find the general solution of the differential equations dydx=sin−1x
View SolutionFind the general solution of the differential equation dydx−y=cosx
View SolutionFind the general solution of the differential equation dydx=log(x+1).
View SolutionThe general solution of the differential equation dydx=2x+5 is
View SolutionFind the general solution of the differential equations dydx+y=1(y≠1)
View SolutionFind the general solution of the differential equations:dxdy+2y=sinx
View SolutionGeneral solution of differential equation x dydx+y=0.
View SolutionFind the general solution of the differential equation dydx=1+y21+x2.
View SolutionFind the general solution of the differential equation
log(dydx)=(ax+by).View SolutionThe general solution of the differential equation xdydx+x=0 is ?
View Solution