Find the mean deviation about the median for the following data:
11, 3, 8, 7, 5, 14, 10, 2, 9
The correct Answer is:3
To find the mean deviation about the median for the given data set 11,3,8,7,5,14,10,2,9, we will follow these steps:
Step 1: Arrange the Data
First, we need to arrange the data in ascending order:
- The data in ascending order is: 2,3,5,7,8,9,10,11,14
Step 2: Find the Median
Since there are 9 data points (which is odd), the median is the middle value. The median can be calculated using the formula:
Median=Value at position n+12
where n is the number of data points. Here, n=9:
Median=Value at position 9+12=Value at position 5
The 5th value in the ordered list is 8. Thus, the median is 8.
Step 3: Calculate Deviations from the Median
Next, we calculate the absolute deviations of each data point from the median:
- For 2: |2−8|=6
- For 3: |3−8|=5
- For 5: |5−8|=3
- For 7: |7−8|=1
- For 8: |8−8|=0
- For 9: |9−8|=1
- For 10: |10−8|=2
- For 11: |11−8|=3
- For 14: |14−8|=6
Step 4: Sum of Deviations
Now, we sum all the absolute deviations:
Sum of deviations=6+5+3+1+0+1+2+3+6=27
Step 5: Calculate Mean Deviation
Finally, we calculate the mean deviation by dividing the total sum of deviations by the number of data points:
Mean Deviation=Sum of deviationsn=279=3
Conclusion
The mean deviation about the median for the given data is 3.
---
First, we need to arrange the data in ascending order:
- The data in ascending order is: 2,3,5,7,8,9,10,11,14
Since there are 9 data points (which is odd), the median is the middle value. The median can be calculated using the formula:
Median=Value at position n+12
where n is the number of data points. Here, n=9:
Median=Value at position 9+12=Value at position 5
The 5th value in the ordered list is 8. Thus, the median is 8.
Next, we calculate the absolute deviations of each data point from the median:
- For 2: |2−8|=6
- For 3: |3−8|=5
- For 5: |5−8|=3
- For 7: |7−8|=1
- For 8: |8−8|=0
- For 9: |9−8|=1
- For 10: |10−8|=2
- For 11: |11−8|=3
- For 14: |14−8|=6
Now, we sum all the absolute deviations:
Sum of deviations=6+5+3+1+0+1+2+3+6=27
Finally, we calculate the mean deviation by dividing the total sum of deviations by the number of data points:
Mean Deviation=Sum of deviationsn=279=3
The mean deviation about the median for the given data is 3.
Topper's Solved these Questions
- MEASURES OF DISPERSIONBook:ICSEChapter:MEASURES OF DISPERSIONExercise:EXERCISE 21 (b)
Explore 19 Videos
- MEASURES OF CENTRAL TENDENCYBook:ICSEChapter:MEASURES OF CENTRAL TENDENCYExercise:EXERCISE
Explore 14 Videos
- MOCK TEST PAPER-2021Book:ICSEChapter:MOCK TEST PAPER-2021Exercise:SECTION - C
Explore 10 Videos
Similar Questions
Find the mean deviation about the mean for the following data :

View SolutionFind the mean deviation about the mean for the following data :

View SolutionFind the mean deviation about the mean for the following data.
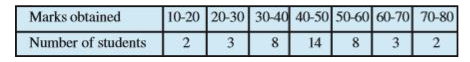
View SolutionFind the mean deviation about the mean for the following data :

View SolutionFind the mean deviation from the median for the following data:
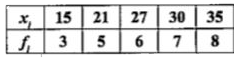
View SolutionFind the mean deviation about median for the following data:

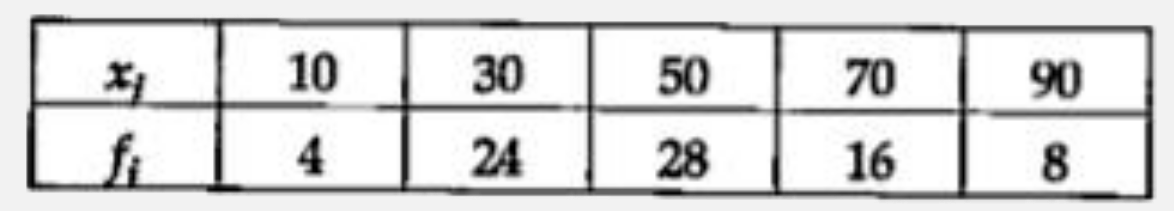
View SolutionFind the mean deviation about mean for the following data:
View SolutionFind the mean deviation about the median for the following data:3,9,5,3,12,10,18,4,7,19,21.
View SolutionFind the mean deviation about the mean for the following data :12, 3, 18, 17, 4, 9, 17, 19, 20, 15, 8, 17, 2, 3, 16, 11, 3, 1, 0, 5
View SolutionFind the mean deviation about the median for the following data:4,6,9,3,10,13,2
View Solution